What Is Least Squares Estimation

What Is Least Squares Estimation Youtube The method of least squares is a parameter estimation method in regression analysis based on minimizing the sum of the squares of the residuals (a residual being the difference between an observed value and the fitted value provided by a model) made in the results of each individual equation. (more simply, least squares is a mathematical. The least squares method is a form of regression analysis that provides the overall rationale for the placement of the line of best fit among the data points being studied. it begins with a set of.
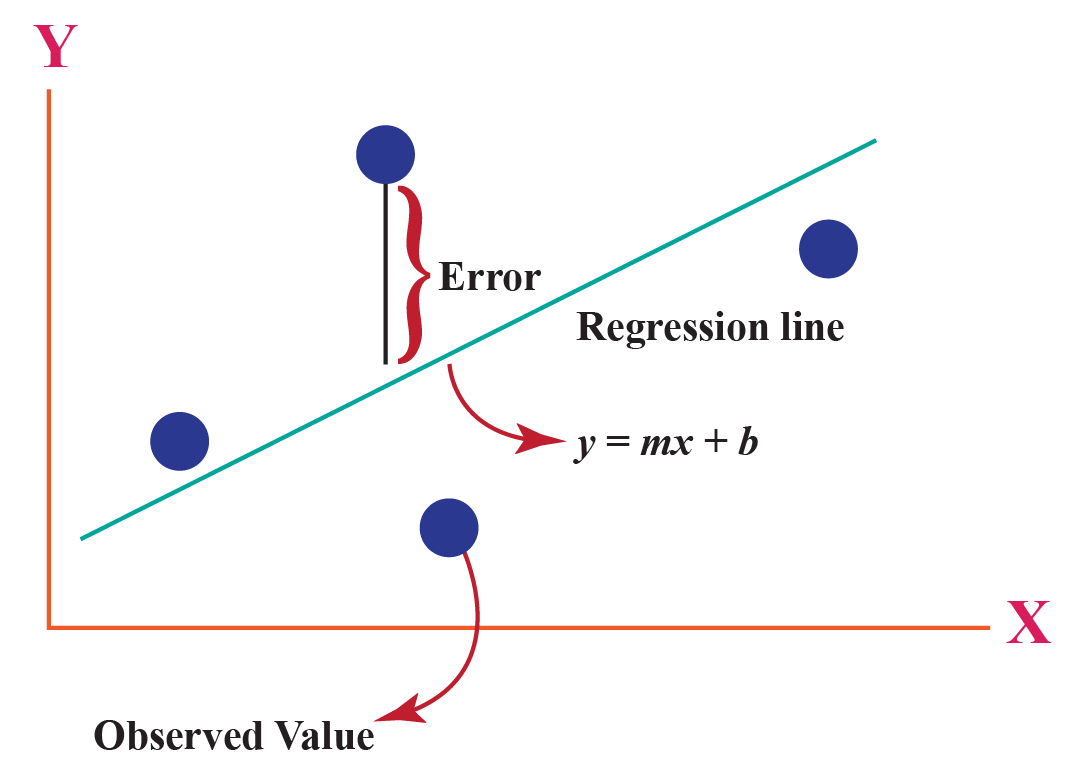
Least Squares Cuemath The steps to find the line of best fit by using the least square method is discussed below: step 1: denote the independent variable values as xi and the dependent ones as yi. step 2: calculate the average values of xi and yi as x and y. step 3: presume the equation of the line of best fit as y = mx c, where m is the slope of the line and c. A least squares regression line represents the relationship between variables in a scatterplot. the procedure fits the line to the data points in a way that minimizes the sum of the squared vertical distances between the line and the points. it is also known as a line of best fit or a trend line. in the example below, we could look at the data. Following are the steps to calculate the least square using the above formulas. step 1: draw a table with 4 columns where the first two columns are for x and y points. step 2: in the next two columns, find xy and (x) 2. step 3: find ∑x, ∑y, ∑xy, and ∑ (x) 2. step 4: find the value of slope m using the above formula. The formula. y = a bx. the formula, for those unfamiliar with it, probably looks underwhelming – even more so given the fact that we already have the values for y and x in our example. having said that, and now that we're not scared by the formula, we just need to figure out the a and b values.
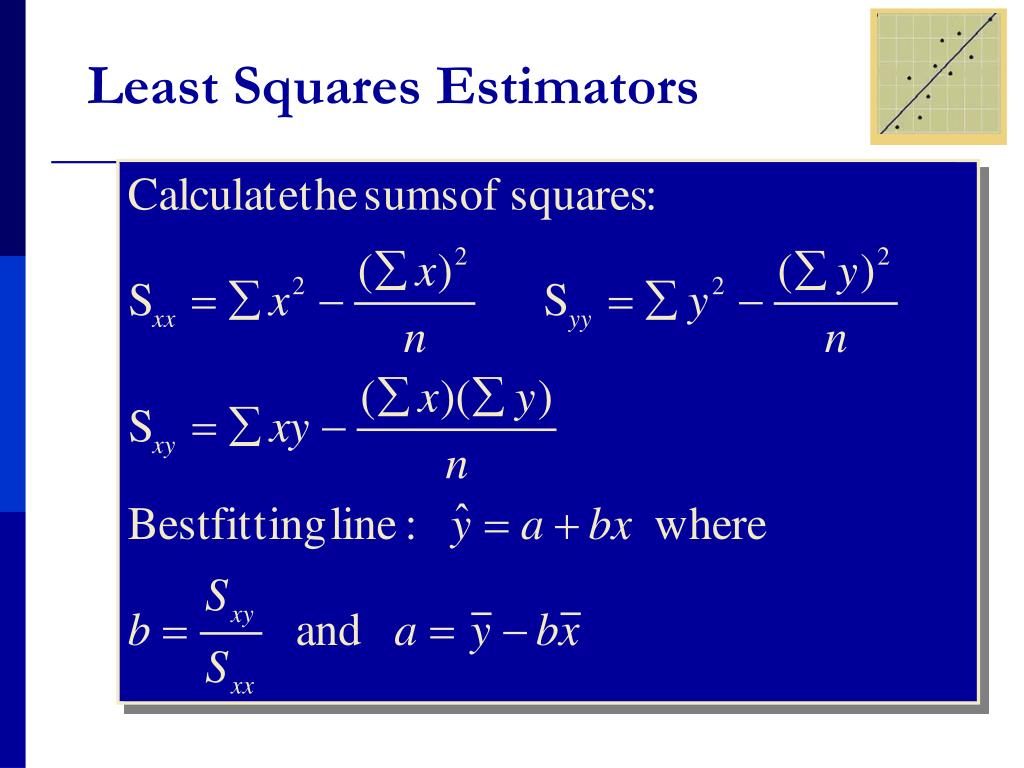
Ppt Introduction To Probability And Statistics Powerpoint Following are the steps to calculate the least square using the above formulas. step 1: draw a table with 4 columns where the first two columns are for x and y points. step 2: in the next two columns, find xy and (x) 2. step 3: find ∑x, ∑y, ∑xy, and ∑ (x) 2. step 4: find the value of slope m using the above formula. The formula. y = a bx. the formula, for those unfamiliar with it, probably looks underwhelming – even more so given the fact that we already have the values for y and x in our example. having said that, and now that we're not scared by the formula, we just need to figure out the a and b values. Be careful! least squares is sensitive to outliers. a strange value will pull the line towards it. use the app. have a play with the least squares calculator. not just for lines. this idea can be used in many other areas, not just lines. a "circle of best fit" but the formulas (and the steps taken) will be very different. The least squares estimate (lse) is a fundamental statistical method used to determine the best fitting line through a set of data points. this technique minimizes the sum of the squares of the residuals, which are the differences between observed and predicted values. by applying lse, analysts can derive a linear equation that represents the.

Comments are closed.