What Happens When You Master Exponential Equations

What Happens When You Master Exponential Equations Youtube Uncontrolled population growth, as in the wild rabbits in australia, can be modeled with exponential functions. equations resulting from those exponential functions can be solved to analyze and make predictions about exponential growth. in this section, we will learn techniques for solving exponential functions. So we can set the exponents to be equal. 3 x = 6. subtracting 3 from both sides, x = 3. therefore, the solution of the given exponential equation is x = 3. example 2: solve the exponential equation 7 3x 7 = 490. solution: 490 cannot be written as a power of 7. so we cannot make the bases to be the same here.
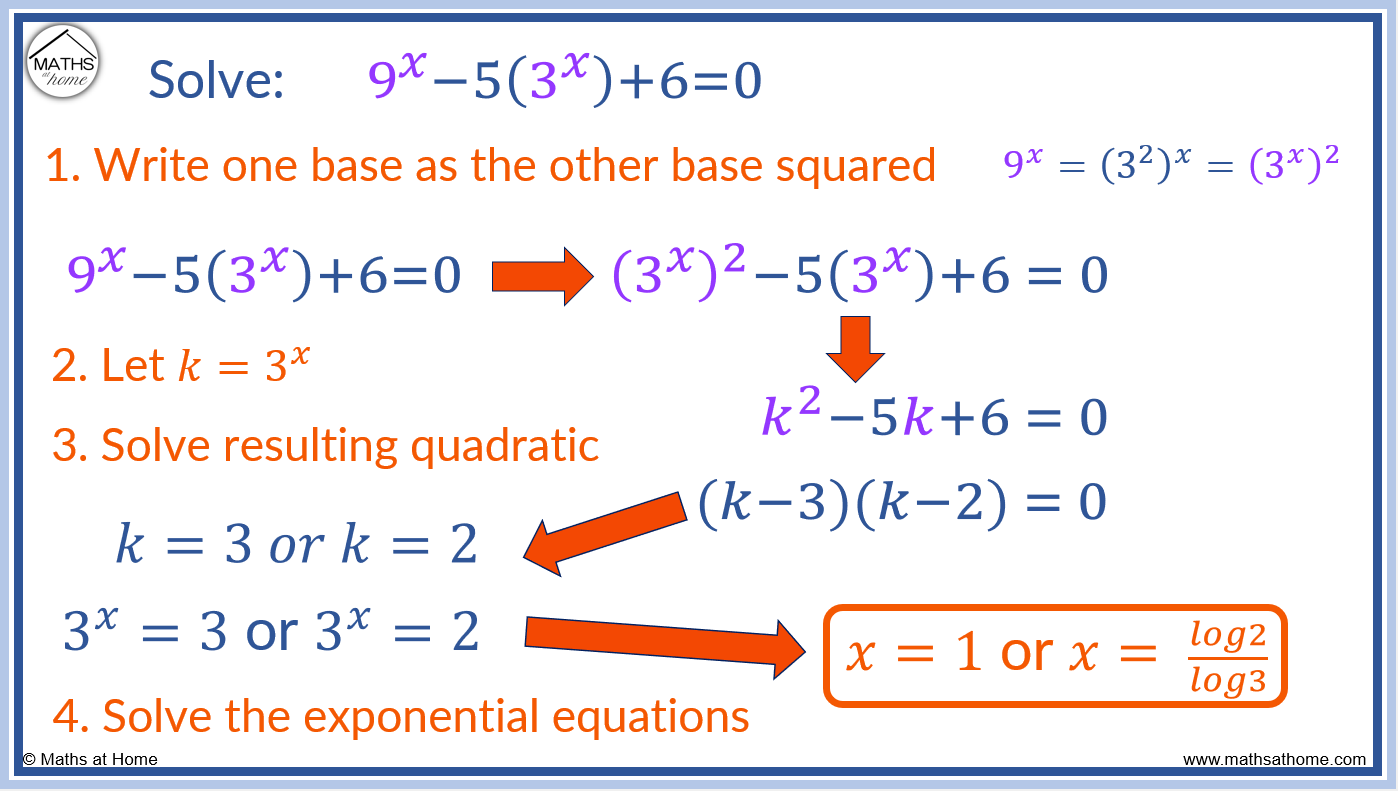
How To Solve An Exponential Equation Mathsathome To solve exponential equations, we need to consider the rule of exponents. these rules help us a lot in solving these type of equations. in solving exponential equations, the following theorem is often useful: here is how to solve exponential equations: manage the equation using the rule of exponents and some handy theorems in algebra. use the theorem above that we just proved. if the bases. We could use one of the inverse properties of exponentials and logarithms listed in theorem 6.3 to write 129 = 2log2 (129). we’d then have 2x = 2log2 (129), which means our solution is x = log2(129). this makes sense because, after all, the definition of log2(129) is ‘the exponent we put on 2 to get 129.’. If bx = by then x =y if b x = b y then x = y. note that this fact does require that the base in both exponentials to be the same. if it isn’t then this fact will do us no good. let’s take a look at a couple of examples. example 1 solve each of the following. 53x =57x−2 5 3 x = 5 7 x − 2. 4t2 = 46−t 4 t 2 = 4 6 − t. 3z = 9z 5 3 z = 9. As you might've noticed, an exponential equation is just a special type of equation. it's an equation that has exponents that are $$ \red{ variables}$$.

What Happens When You Master Exponential Equations Youtube If bx = by then x =y if b x = b y then x = y. note that this fact does require that the base in both exponentials to be the same. if it isn’t then this fact will do us no good. let’s take a look at a couple of examples. example 1 solve each of the following. 53x =57x−2 5 3 x = 5 7 x − 2. 4t2 = 46−t 4 t 2 = 4 6 − t. 3z = 9z 5 3 z = 9. As you might've noticed, an exponential equation is just a special type of equation. it's an equation that has exponents that are $$ \red{ variables}$$. Exponential equations are classified into three categories: with the same bases on both sides: 4^x = 4^2. with different bases can be made the same: 4^x= 16 which can be written as 4^x = 4^2. with different bases that cannot be made the same: 4^x = 15. when the bases on both sides of the equation are not the same, we use logarithms to solve the. If we are given equations involving exponentials or the natural logarithm, remember that you can take the exponential of both sides of the equation to get rid of the logarithm or take the natural logarithm of both sides to get rid of the exponential. example. solve 0.5 = e x. then ln (0.5) = ln (e x) ln (0.5) = x.

Exponential Equations Examplanning Exponential equations are classified into three categories: with the same bases on both sides: 4^x = 4^2. with different bases can be made the same: 4^x= 16 which can be written as 4^x = 4^2. with different bases that cannot be made the same: 4^x = 15. when the bases on both sides of the equation are not the same, we use logarithms to solve the. If we are given equations involving exponentials or the natural logarithm, remember that you can take the exponential of both sides of the equation to get rid of the logarithm or take the natural logarithm of both sides to get rid of the exponential. example. solve 0.5 = e x. then ln (0.5) = ln (e x) ln (0.5) = x.

Comments are closed.