W2 First Order Differential Equations Part 1 Module Differential

W2 First Order Differential Equations Part 1 Module Pdf Ordinary Explain what is meant by separating the variables of a first order. differen tial equation. 2. determine whether a first order differential equation is separable. 3. solve a variety of equations using this technique. separation of var i ables. equations which can be written in t he form 𝑑𝑦. 𝑑𝑥 =𝑓(𝑥),𝑑𝑦. Study with quizlet and memorize flashcards containing terms like to solve first order linear ode in form y prime p(x) = q(x), integrating factor solve by plugging u(x) into:, method to solve 1st order linear nonlinear ode in form dy dx = g(x)f(y) or dy dx = g(x) f(y) and more.

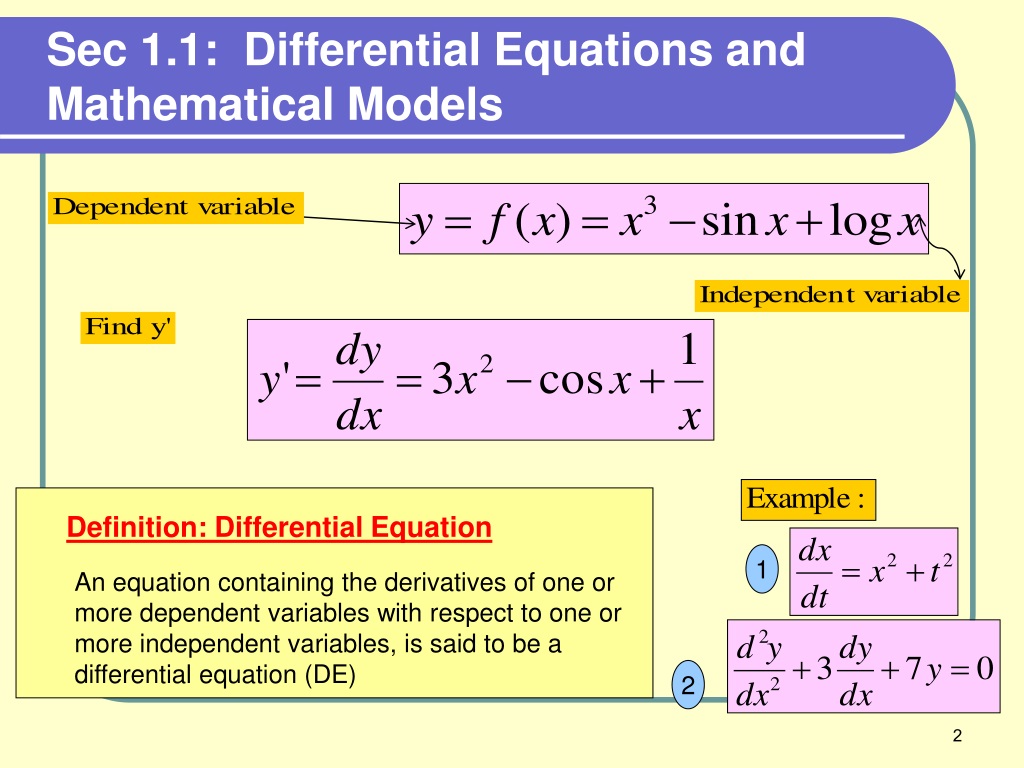
First Order Differential Equation Flowchart Linear equations – in this section we solve linear first order differential equations, i.e. differential equations in the form \(y' p(t) y = g(t)\). we give an in depth overview of the process used to solve this type of differential equation as well as a derivation of the formula needed for the integrating factor used in the solution process. A first order differential equation is an equation of the form f(t, y, ˙y) = 0. a solution of a first order differential equation is a function f(t) that makes f(t, f(t), f ′ (t)) = 0 for every value of t. here, f is a function of three variables which we label t, y, and ˙y. it is understood that ˙y will explicitly appear in the equation. A first order equation is separable if it can be written the form. dy dx = f(x)g(y) special cases result when either f(x) = 1 or g(y) = 1. in the first case the equation is said to be autonomous. separable equations. the general solution to equation 1.2.1 is obtained in terms of two integrals:. 1 1.5 2 3. separable equations even rst order odes are complicated enough that exact solutions are not easy to obtain in general .one type that can be solved exactly is a separable equation, which is a rst order ode of the form f(y) dy dx = g(x) (3.1) for functions f;g. this can be integrated directly, if you recall the chain rule d dx (f(y(x.

Solution Module2 First Order Differential Equations Studypool A first order equation is separable if it can be written the form. dy dx = f(x)g(y) special cases result when either f(x) = 1 or g(y) = 1. in the first case the equation is said to be autonomous. separable equations. the general solution to equation 1.2.1 is obtained in terms of two integrals:. 1 1.5 2 3. separable equations even rst order odes are complicated enough that exact solutions are not easy to obtain in general .one type that can be solved exactly is a separable equation, which is a rst order ode of the form f(y) dy dx = g(x) (3.1) for functions f;g. this can be integrated directly, if you recall the chain rule d dx (f(y(x. The differential equation for the problem can be expressed in a slightly different form from a first order differential equation in (7.24) to be: dt ( x ) q q for the copper wire. dx k 3 . 95. cu. q. the solution of the above differential equation is: t ( x ) x c where c=integration constant. Our study of first order differential equations requires an additional assumption, namely that the equation can be solved for y0. this means that we can write the equation in the form y0 = f(x,y). (f) 2.1. linear differential equations a first order differential equation y0 = f(x,y) is a linear equation if the function f is a “linear.
Ppt Chapter 1 First Order Differential Equations Powerpoint The differential equation for the problem can be expressed in a slightly different form from a first order differential equation in (7.24) to be: dt ( x ) q q for the copper wire. dx k 3 . 95. cu. q. the solution of the above differential equation is: t ( x ) x c where c=integration constant. Our study of first order differential equations requires an additional assumption, namely that the equation can be solved for y0. this means that we can write the equation in the form y0 = f(x,y). (f) 2.1. linear differential equations a first order differential equation y0 = f(x,y) is a linear equation if the function f is a “linear.

First Order Differential Equation Flowchart

Comments are closed.