Ppt Sine Ratio Powerpoint Presentation Free Download Id 5200645 Example of right triangle trigonometry calculations with steps. take a right triangle with hypotenuse c = 5 c = 5 and an angle \alpha=38\degree α= 38°. surprisingly enough, this is enough data to fully solve the right triangle! follow these steps: calculate the third angle: β = 90 ° − α. \beta = 90\degree \alpha β = 90°−α. The cotangent function: cot(θ) = x y cot (θ) = x y. example 1.2.1 1.2. 1. the point (3, 4) is on the circle of radius 5 at some angle θ θ. find the six trigonometric function values of θ θ. solution. we have x = 3 x = 3, y = 4 y = 4, and r = 5 r = 5. using the previously listed definitions we have.
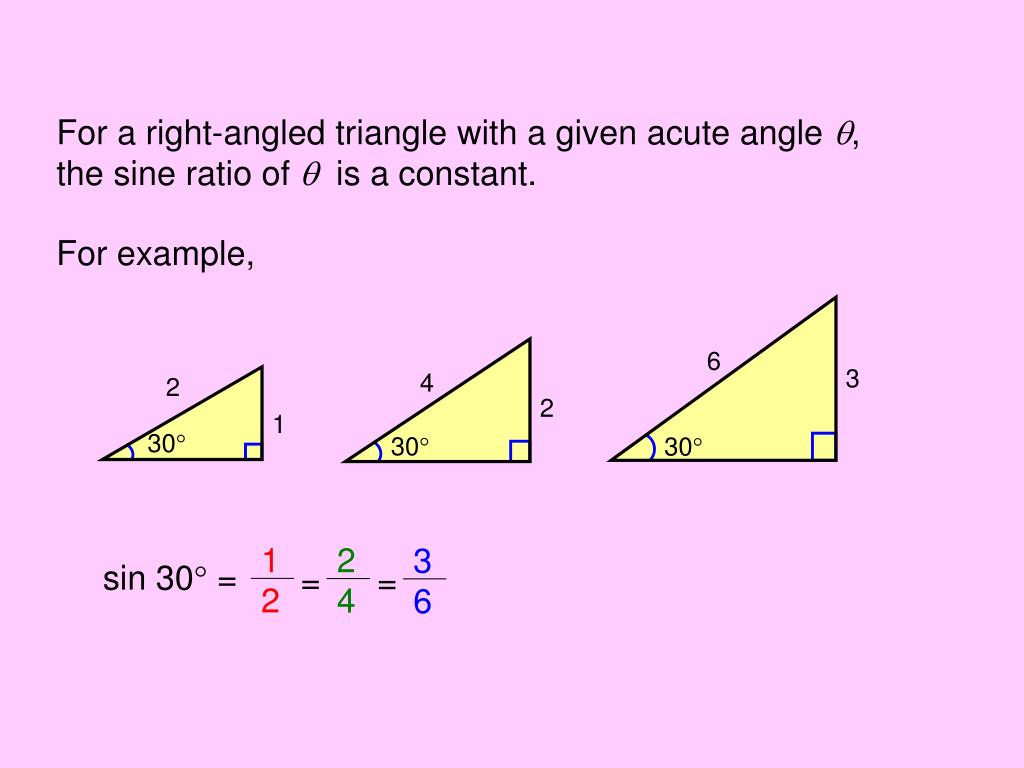
Trigonometric Ratios L5 Using Sine Ratio In Right Angles Triangles 1 use measurements to calculate the trigonometric ratios for acute angles #1 10, 57 60. 2 use trigonometric ratios to find unknown sides of right triangles #11 26. 3 solve problems using trigonometric ratios #27 34, 41 46. 4 use trig ratios to write equations relating the sides of a right triangle #35 40. The trigonometric function relating the side opposite to an angle and the side adjacent to the angle is the tangent. so we will state our information in terms of the tangent of 57°, letting h be the unknown height. tanθ = opposite adjacent tan(57°) = h 30 solve for h. h = 30tan(57°) multiply. h ≈ 46.2 use a calculator. Example. find the size of angle a°. step 1 the two sides we know are a djacent (6,750) and h ypotenuse (8,100). step 2 soh cah toa tells us we must use c osine. step 3 calculate adjacent hypotenuse = 6,750 8,100 = 0.8333. step 4 find the angle from your calculator using cos 1 of 0.8333: cos a° = 6,750 8,100 = 0.8333. Hypotenuse. therefore, we will use the tangent ratio: opposite sin( )θ= hypotenuse 14 sin(55 ) x d= x⋅sin(55 ) 14d= 14 sin(55 ) x = d x ≈17.0908 finding missing angles of right triangles we will now learn to use the three basic trigonometric ratios to find missing angles of right triangles. tutorial:.
The Sine Ratio Youtube Example. find the size of angle a°. step 1 the two sides we know are a djacent (6,750) and h ypotenuse (8,100). step 2 soh cah toa tells us we must use c osine. step 3 calculate adjacent hypotenuse = 6,750 8,100 = 0.8333. step 4 find the angle from your calculator using cos 1 of 0.8333: cos a° = 6,750 8,100 = 0.8333. Hypotenuse. therefore, we will use the tangent ratio: opposite sin( )θ= hypotenuse 14 sin(55 ) x d= x⋅sin(55 ) 14d= 14 sin(55 ) x = d x ≈17.0908 finding missing angles of right triangles we will now learn to use the three basic trigonometric ratios to find missing angles of right triangles. tutorial:. If needed, draw the right triangle and label the angle provided. identify the angle, the adjacent side, the side opposite the angle, and the hypotenuse of the right triangle. find the required function: sine as the ratio of the opposite side to the hypotenuse. cosine as the ratio of the adjacent side to the hypotenuse. When we are given the length of one side of the right triangle and an angle 𝜃 in the right triangle, different from the right angle, we can use right triangle trigonometry to compute the lengths of the remaining sides. we have three different choices for trigonometric ratios: sine, cosine, and tangent.
Comments are closed.