Using Mathematical Induction To Prove A Formula
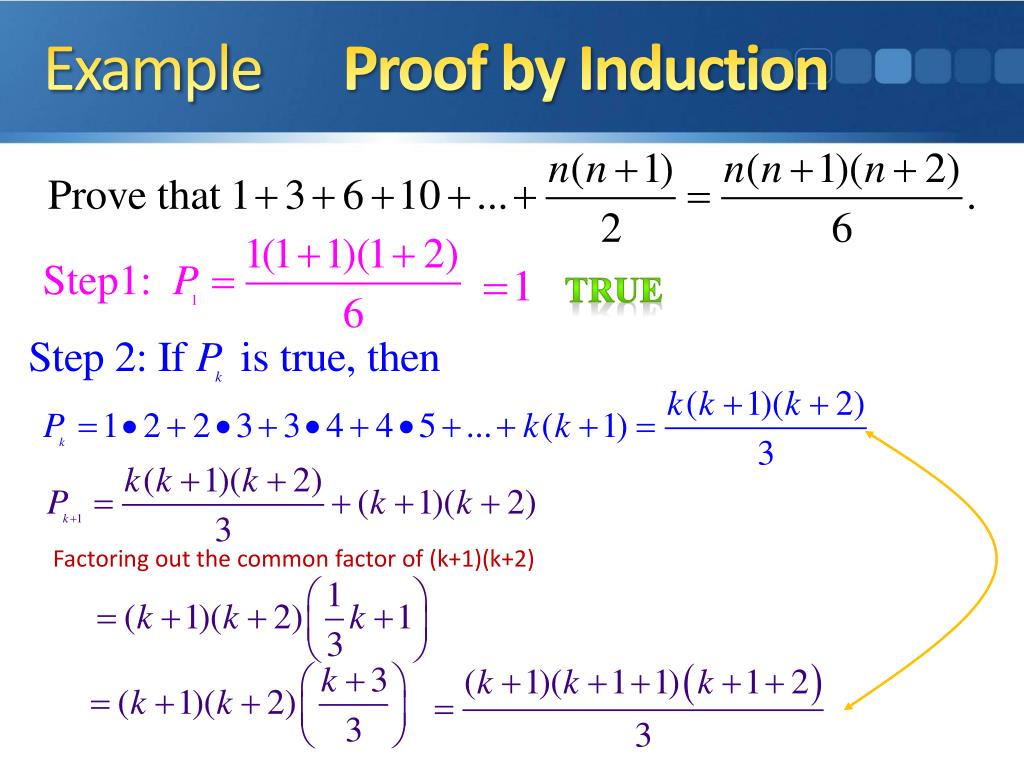
Ppt Mathematical Induction Powerpoint Presentation Free Download Thus far, we have learned how to use mathematical induction to prove identities. in general, we can use mathematical induction to prove a statement about \(n\). this statement can take the form of an identity, an inequality, or simply a verbal statement about \(n\). we shall learn more about mathematical induction in the next few sections. Mathematical induction (or weak mathematical induction) is a method to prove or establish mathematical statements, propositions, theorems, or formulas for all natural numbers ‘n ≥1.’ principle. it involves two steps: base step: it proves whether a statement is true for the initial value (n), usually the smallest natural number in.
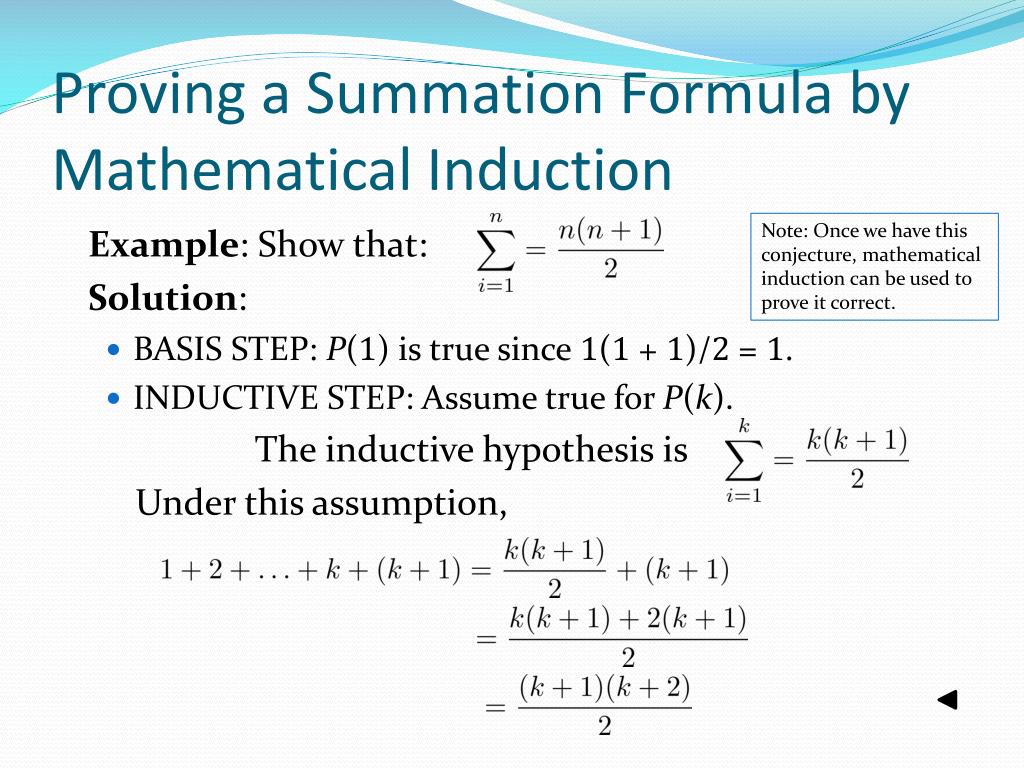
Ppt Mathematical Induction Powerpoint Presentation Free Download The primary use of the principle of mathematical induction is to prove statements of the form. (∀n ∈ n)(p(n)). where p(n) is some open sentence. recall that a universally quantified statement like the preceding one is true if and only if the truth set t of the open sentence p(n) is the set n. The principle of mathematical induction is used to prove mathematical statements suppose we have to prove a statement p (n) then the steps applied are, step 1: prove p (k) is true for k =1. step 2: let p (k) is true for all k in n and k > 1. step 3: prove p (k 1) is true using basic mathematical properties. thus, if p (k 1) is true then we say. Process of proof by induction. there are two types of induction: regular and strong. the steps start the same but vary at the end. here are the steps. in mathematics, we start with a statement of our assumptions and intent: let p(n), ∀n ≥ n0, n, n0 ∈ z be a statement. we would show that p (n) is true for all possible values of n. That is how mathematical induction works. in the world of numbers we say: step 1. show it is true for first case, usually n=1; step 2. show that if n=k is true then n=k 1 is also true; how to do it. step 1 is usually easy, we just have to prove it is true for n=1. step 2 is best done this way: assume it is true for n=k.

Mathematical Induction Examples Solutions Youtube Process of proof by induction. there are two types of induction: regular and strong. the steps start the same but vary at the end. here are the steps. in mathematics, we start with a statement of our assumptions and intent: let p(n), ∀n ≥ n0, n, n0 ∈ z be a statement. we would show that p (n) is true for all possible values of n. That is how mathematical induction works. in the world of numbers we say: step 1. show it is true for first case, usually n=1; step 2. show that if n=k is true then n=k 1 is also true; how to do it. step 1 is usually easy, we just have to prove it is true for n=1. step 2 is best done this way: assume it is true for n=k. The proof by mathematical induction (simply known as induction) is a fundamental proof technique that is as important as the direct proof, proof by contraposition, and proof by contradiction. it is usually useful in proving that a statement is true for all the natural numbers . in this case, we are going to prove summation statements that. General, a proof using the weak induction principle above will look as follows: mathematical induction to prove a statement of the form 8n a; p(n) using mathematical induction, we do the following. 1.prove that p(a) is true. this is called the \base case." 2.prove that p(n) )p(n 1) using any proof method. what is commonly done here is to use.
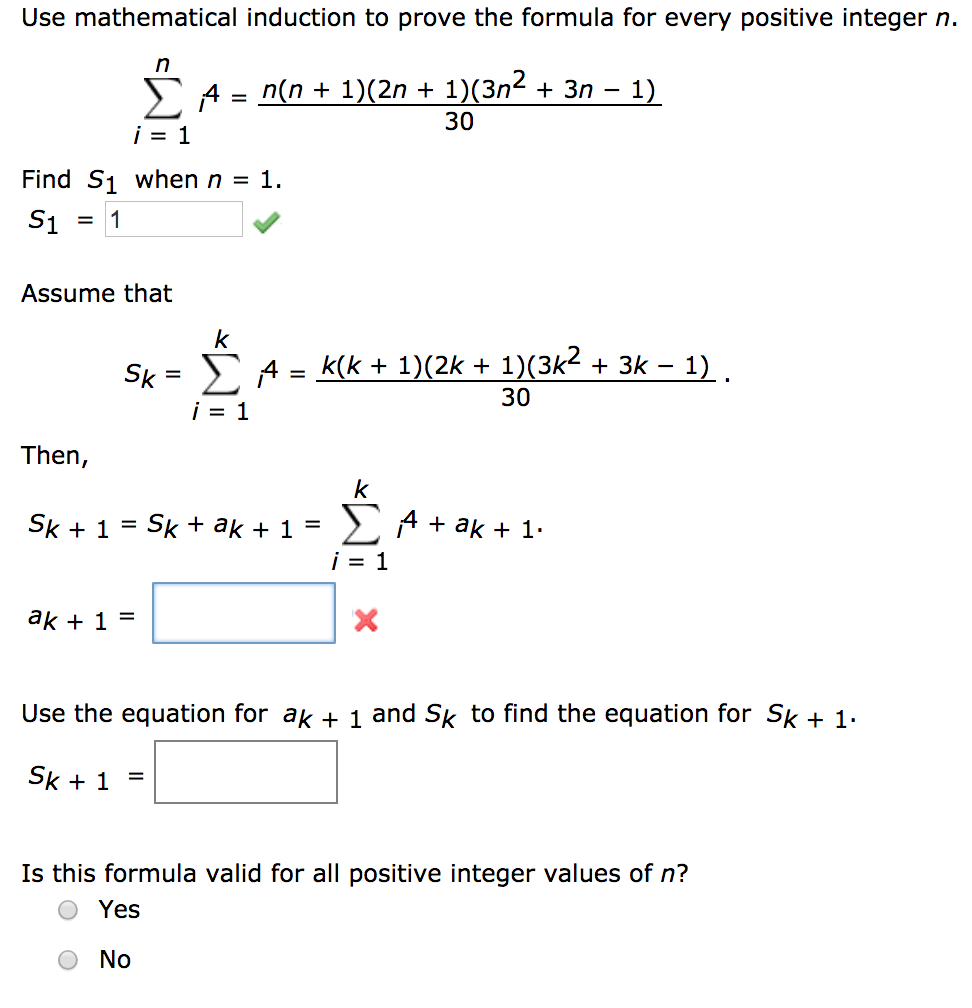
Solved Use Mathematical Induction To Prove The Formula For Chegg The proof by mathematical induction (simply known as induction) is a fundamental proof technique that is as important as the direct proof, proof by contraposition, and proof by contradiction. it is usually useful in proving that a statement is true for all the natural numbers . in this case, we are going to prove summation statements that. General, a proof using the weak induction principle above will look as follows: mathematical induction to prove a statement of the form 8n a; p(n) using mathematical induction, we do the following. 1.prove that p(a) is true. this is called the \base case." 2.prove that p(n) )p(n 1) using any proof method. what is commonly done here is to use.

Solved Use Mathematical Induction To Prove The Formula Fo Chegg

Comments are closed.