Trigonometric Functions And Angle Measures Studocu
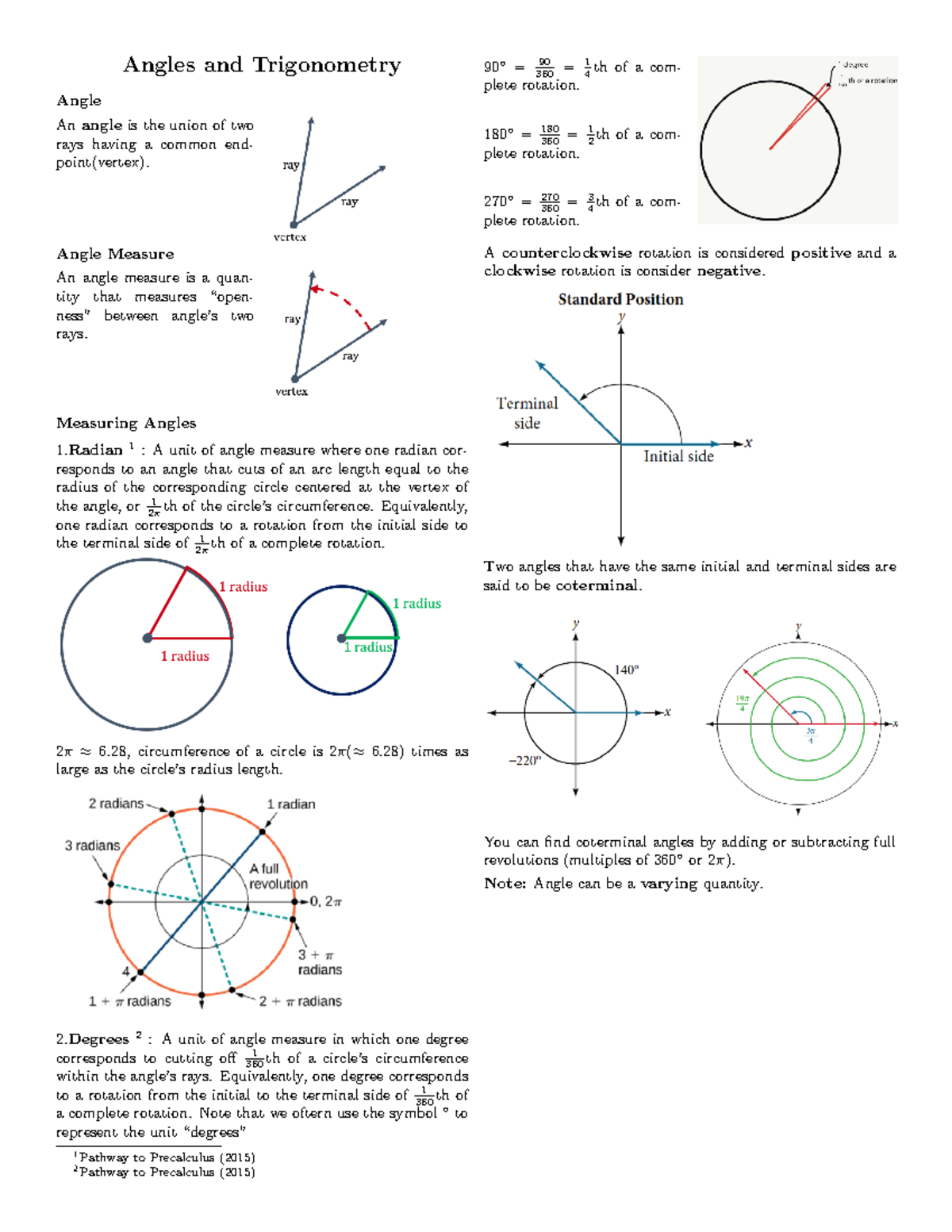
M2 Angle Measure And Trig Functions Overview Notes Angles And If the rotation is clockwise, the angle is negative as shown on the right below. r1 o a b r2 r2 b o r1 a angle measure there are two commonly used measures for angles, degrees and radians. in calculus, we will almost exclusively use radian measure since the trigonometric functions are defined in terms of this measure. Chapter 6: trigonometric functions – right triangle approach. 6 – angle measure. a. warm up: label the special numbers and terminal points in the first quadrant. then use reference numbers to fill in the remaining special numbers and terminal points for the other quadrants. sketch the parent graphs for all 6 trig functions: b. angles.

Chapter 3 Trigonometric Functions Maths Class 11 Chapter 3 Thus, the terminal side is in the same exact position as the initial side. in trigonometry, angles can have a measure of many revolutions there is no limit to the magnitude of a given angle. a revolution can be abbreviated "rev". degrees. a more common way to measure angles is in degrees. there are 360 degrees in one revolution. The six basic trigonometric functions. trigonometric functions allow us to use angle measures, in radians or degrees, to find the coordinates of a point on any circle—not only on a unit circle—or to find an angle given a point on a circle. they also define the relationship among the sides and angles of a triangle. Radian. a unit of angle measure such that an angle with center at the center of a unit circle, with measure one radian produces an arc with arc length one. law of cosines. in a triangle with vertices a, b, and c and sides of lengths a, b, and c opposite the angle with the same letter name, c^2 = a^2 b^2 2abcosc. Definition: trigonometric functions. let p = (x, y) be a point on the unit circle centered at the origin o. let θ be an angle with an initial side along the positive x axis and a terminal side given by the line segment op. the trigonometric functions are then defined as. sinθ = y. cscθ = 1 y. cosθ = x. secθ = 1 x.
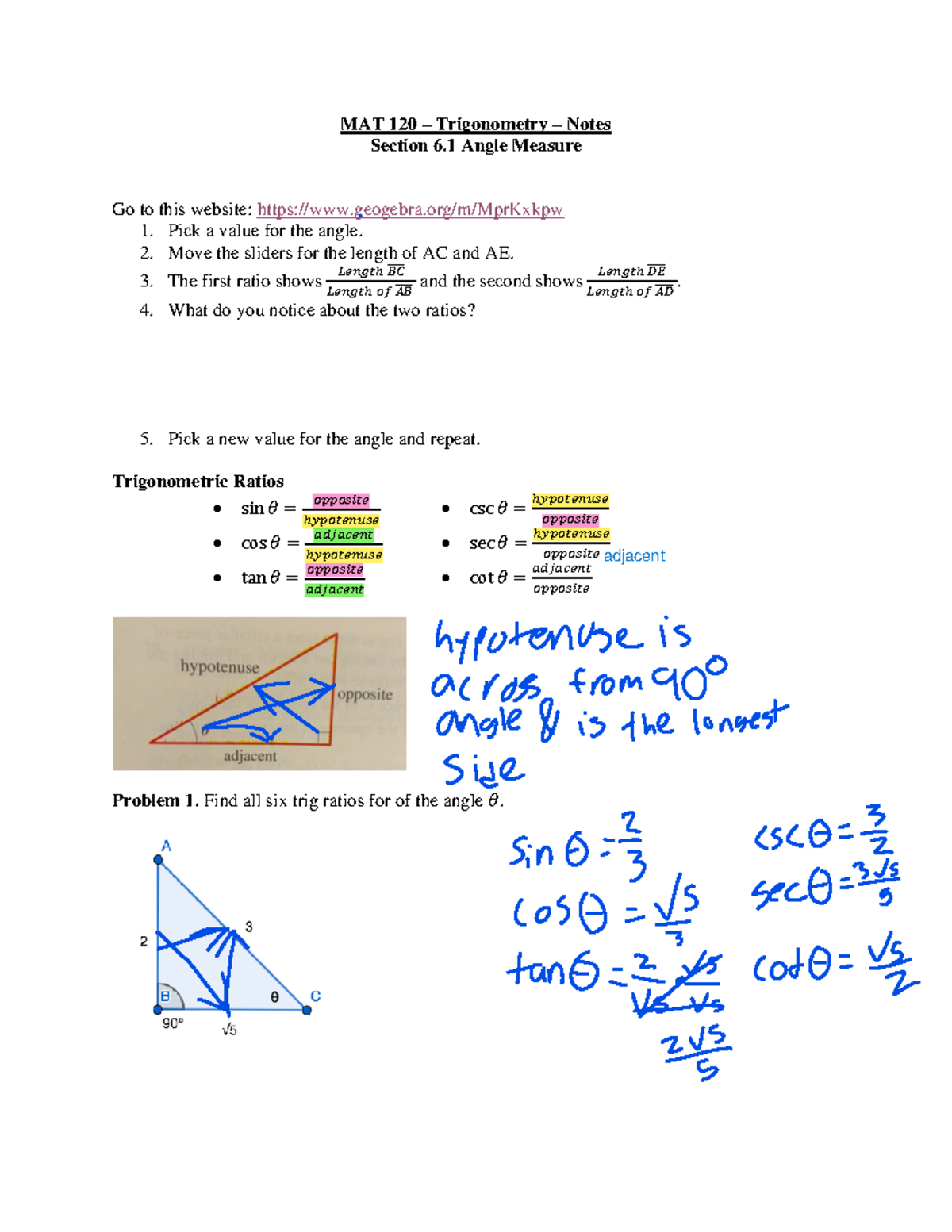
Mat 120 Notes 6 From Mat120 2023 24 Mat 120 Trigonometry Radian. a unit of angle measure such that an angle with center at the center of a unit circle, with measure one radian produces an arc with arc length one. law of cosines. in a triangle with vertices a, b, and c and sides of lengths a, b, and c opposite the angle with the same letter name, c^2 = a^2 b^2 2abcosc. Definition: trigonometric functions. let p = (x, y) be a point on the unit circle centered at the origin o. let θ be an angle with an initial side along the positive x axis and a terminal side given by the line segment op. the trigonometric functions are then defined as. sinθ = y. cscθ = 1 y. cosθ = x. secθ = 1 x. If one angle is an reference angle of another, the trigonometric functions of these two angles have the same values in magnitude, and we just need to pay attention to their signs based on the quadrant that the terminal side of the angle lies in. for example, 30° is the reference angle of 210° with sine values sin(30°)= and sin(210°)=. Trigonometric functions of an angle. with the notation in figure 3.1, we see that cos(t) = x and sin(t) = y. in this context, we often the cosine and sine circular functions because they are defined by points on the unit circle. now we want to focus on the perspective the cosine and sine as functions of angles.
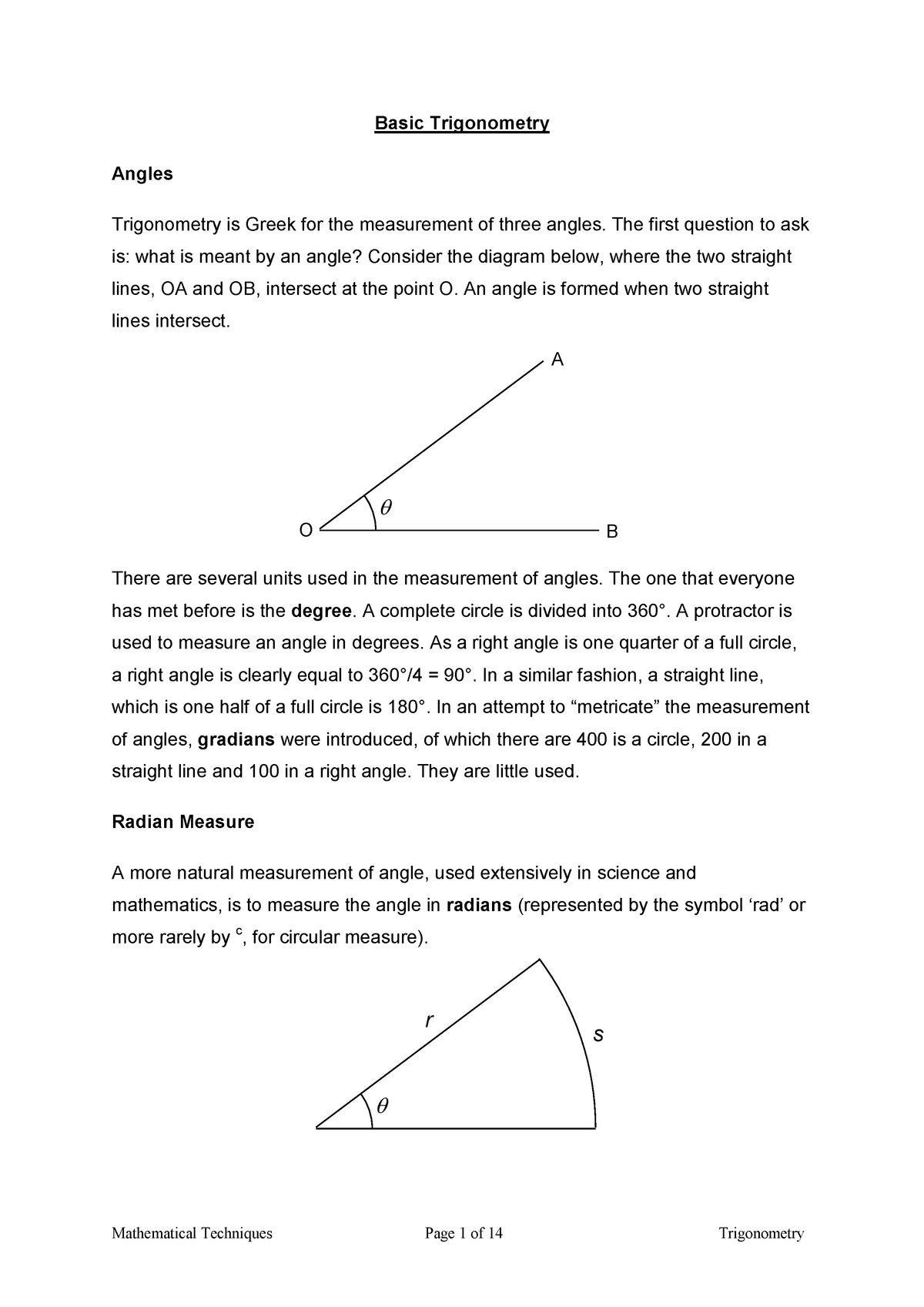
Lecture 1 Trigonometry Basic Trigonometry Angles Trigonometry Is If one angle is an reference angle of another, the trigonometric functions of these two angles have the same values in magnitude, and we just need to pay attention to their signs based on the quadrant that the terminal side of the angle lies in. for example, 30° is the reference angle of 210° with sine values sin(30°)= and sin(210°)=. Trigonometric functions of an angle. with the notation in figure 3.1, we see that cos(t) = x and sin(t) = y. in this context, we often the cosine and sine circular functions because they are defined by points on the unit circle. now we want to focus on the perspective the cosine and sine as functions of angles.

Comments are closed.