Geometry Three Externally Touching Circles Have Their Centers On The Download scientific diagram | three externally touching circles with radii í µí² , í µí² & í µí² are inscribed by the circle with center o and r from publication: mathematical analysis. Learn to apply systems of equations in solving geometry problems. how to find the radii of three circles touching each other externally or externally tangent.
Three Circles Touch Each Other Externally Find The Radii Of The Elementary solution : the top two right triangles in your diagram are similar. so $$ \frac{height}{base} = \frac{b a}{b a} = \frac{c b}{c b} $$ by componendo and. This means that one of the angles of the parallelogram is right. therefore, based on the rectangle attribute, АОО 1 В is a rectangle. the distance between side АВ and ОО 1 is equal to the radius of the circle—that is, it is equal to r. proof of the property of the circles that touch externally. step 3. Property of external touching circles with different radii. if two circles with different radii touch externally, their centers and crossing point lie on the bisector of an angle formed by common external tangent lines. c is the common tangent line of two circles that touch externally. the centers of the circles lie on different sides of c. Suppose ab = 3cm, bc = 3cm and ac = 4cm. find the radii of the circles. when two circles touch externally, the distance between their center is the sum of their radii. b c = 3. (3) where a, b and c are the radii of the three circles with the centers a, b and c respectively. to solve the system, first add all the three equations.
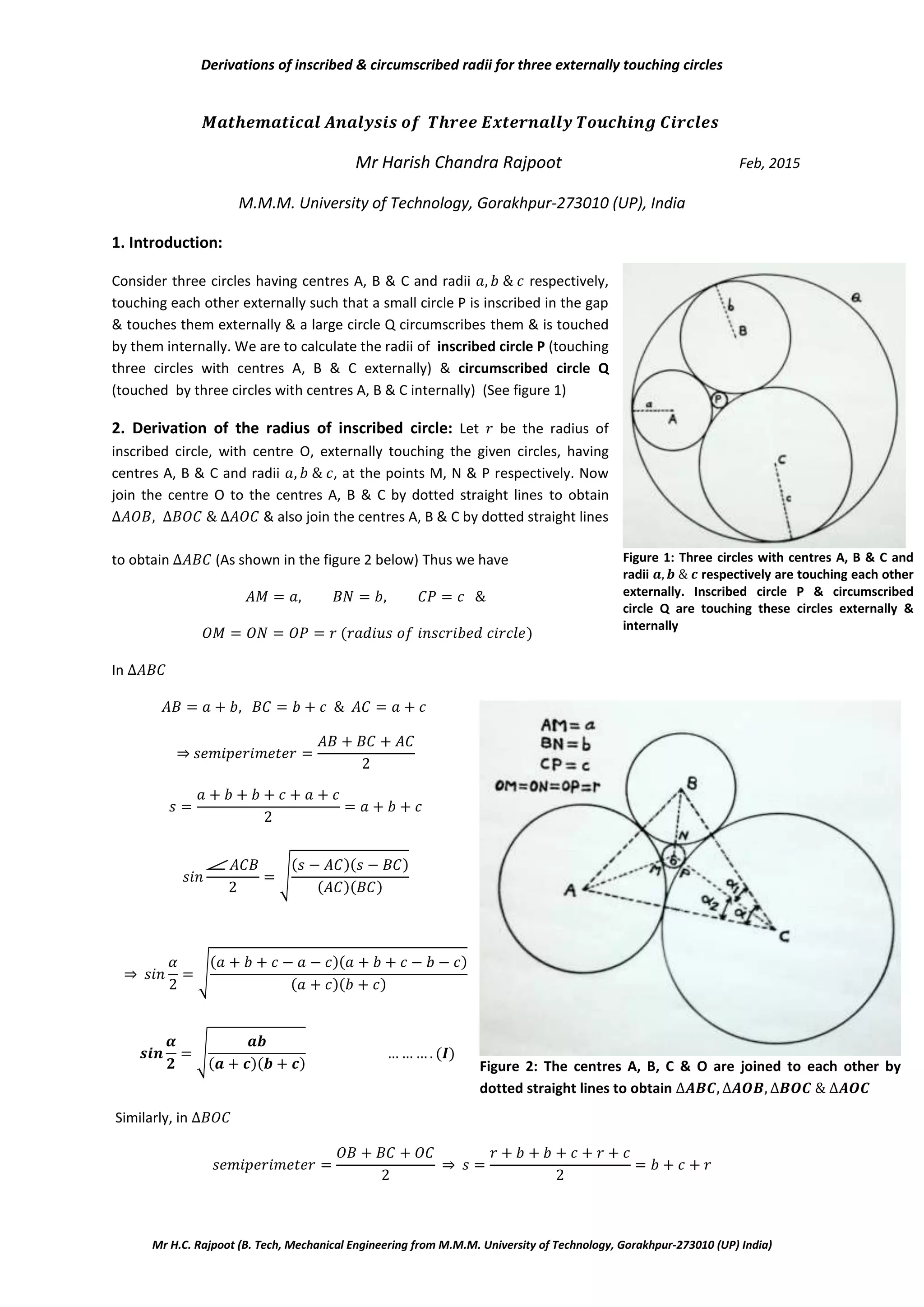
Derivations Of Inscribed Circumscribed Radii For Three Externally Property of external touching circles with different radii. if two circles with different radii touch externally, their centers and crossing point lie on the bisector of an angle formed by common external tangent lines. c is the common tangent line of two circles that touch externally. the centers of the circles lie on different sides of c. Suppose ab = 3cm, bc = 3cm and ac = 4cm. find the radii of the circles. when two circles touch externally, the distance between their center is the sum of their radii. b c = 3. (3) where a, b and c are the radii of the three circles with the centers a, b and c respectively. to solve the system, first add all the three equations. Step 1. consider two circles with centers at points o and О 1 and radii r and r: let’s assume these circles touch externally in point c. let’s draw tangent line a to these circles. let’s designate the touching points of the circle and tangent line as a and b. let’s prove that the formed angle acb is right. Test yourself. finding the centre and radius of a circle when given the equation of a circle of form (x h)^2 (y k)^2 = r^2 | questions. tutorial. finding the equation of a circle with centre (h,k) and having a line as a tangent. test yourself.
Comments are closed.