Theory Of Relativity Lecture Notes 3 Special Theory Of Relativity

Theory Of Relativity Lecture Notes 3 Special Theory Of Relativity Relativistic dynamics and particle physics. relativistic momentum inferred from gedanken experiment with inelastic collisions. relativistic relations between force and acceleration. relativistic version of work energy theorem. kinetic energy, rest energy, equivalence of mass energy. e 2 p 2 invariant. 3 frames of reference and newton’s laws the cornerstone of the theory of special relativity is the principle of relativity: the laws of physics are the same in all inertial frames of reference. we shall see that many surprising consequences follow from this innocuous looking statement.

Relativity Postulates Of Special Theory Of Relativity Relativity The theory of special relativity, originally proposed by albert einstein in his famous 1905 paper, has had profound consequences on our view of physics, space, and time. this course will introduce you to the concepts behind special relativity including, but not limited to, length contraction, time dilation, the lorentz transformation, relativistic kinematics, doppler shifts, and even so called. This latter theory deals with the extension of the special theory to the case of the law of gravitation; we shall not discuss the general theory here. the principle of relativity was first stated by newton, in one of his corollaries to the laws of motion: “the motions of bodies included in a given space are the same among themselves, whether. To do this we start with a known four vector. recall that the four veolcity of any object in its own reference frame is given by (c; 0, 0, 0). thus, let us look at the four velocity of the frame s′ in the two frames s and s′. let v be the velocity (say, along the x direction for simplicity) of s′ with respect to s. Of relativity. in a fixed inertial frame, s,wedrawonedirectionofspace—sayx — along the horizontal axis and time on the vertical axis. but things look much nicer if we rescale time and plot ct on the vertical instead. in the context of special relativity, space and time is called minkowski space. (although the true definition of minkowski.

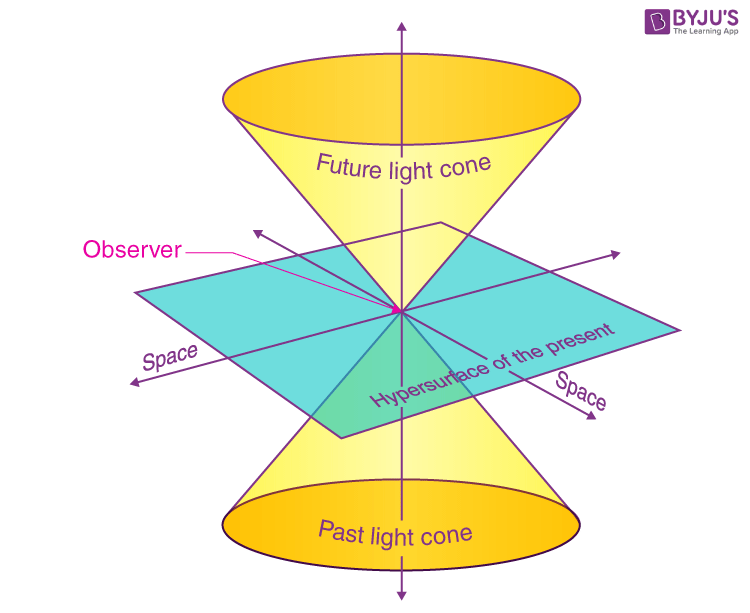
Special Theory Of Relativity Einstein S Theory Of Relativity Str To do this we start with a known four vector. recall that the four veolcity of any object in its own reference frame is given by (c; 0, 0, 0). thus, let us look at the four velocity of the frame s′ in the two frames s and s′. let v be the velocity (say, along the x direction for simplicity) of s′ with respect to s. Of relativity. in a fixed inertial frame, s,wedrawonedirectionofspace—sayx — along the horizontal axis and time on the vertical axis. but things look much nicer if we rescale time and plot ct on the vertical instead. in the context of special relativity, space and time is called minkowski space. (although the true definition of minkowski. S, from the theory, and this theory must be the same in all irfs, then it must be that the speed of light, c, is the same in all irfs. this latter result is sufficient to effect the change of all kinematics and dynamics. [n3] [kin] [pgr] 1905 [psr] [max] [c] special relativity fundamental conflict 3 special relativistic kinematics. Step 2: if an object of a physical theory turns out to be invariant under a group g then the theory is said to be invariant under this ’symmetry group’. this is the most successful way in the history of physics to build a theory. why some groups are ’realised’ in nature and (most) others are not, is not known.