The Vectorial Part Of The Wave Equation Derived From The Maxwell

The Vectorial Part Of The Wave Equation Derived From The Maxwell Equation [6] is known as the wave equation it is actually 3 equations, since we have an x , y and z component for the e field. to break down and understand equation [6], let's imagine we have an e field that exists in source free region. suppose we only have an e field that is polarized in the x direction, which means that ey=ez=0 (the y and. The wave equations as stated in eqs. (2.1) and (2.2) do not impose any conditions on the media and hence are generally valid. 2.1 homogeneous solution in free space we first consider the solution of the wave equations in free space, in absence of matter and sources. for this case the right hand sides of the wave equations are 19.

The Vectorial Part Of The Wave Equation Derived From The Maxwell 0 kz − ω t ) ˆ i. the equation above contains the complete information about the electromagnetic wave: 1. direction of wave propagation: the argument of the sine form in the electric field can be rewritten as ( kz − ω t ) = k ( z − vt ) , which indicates that the wave is propagating in the z direction. 2. The electromagnetic wave equation is a second order partial differential equation that describes the propagation of electromagnetic waves through a medium or in a vacuum. it is a three dimensional form of the wave equation. the homogeneous form of the equation, written in terms of either the electric field e or the magnetic field b, takes the form:. Light is a pair of 3d vector fields. xx ˆ yy ˆ zz ˆ in 3d space. a light wave has both electric and magnetic 3d vector fields. in this illustration, the vectors of the two fields are only shown at a few selected locations, equally spaced along a line. but the fields are defined at every point (x,y,z). the equations of optics are maxwell’s. The scalar wave equation can then be written as. 2u −n2e0μ0∂2u ∂t2 = 0. 2 u − n 2 e 0 μ 0 ∂ 2 u ∂ t 2 = 0. the speed of light in matter is. c n = 1 (eμ0)1 2. c n = 1 (e μ 0) 1 2. this page titled 1.5: the scalar and vector wave equation is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by.

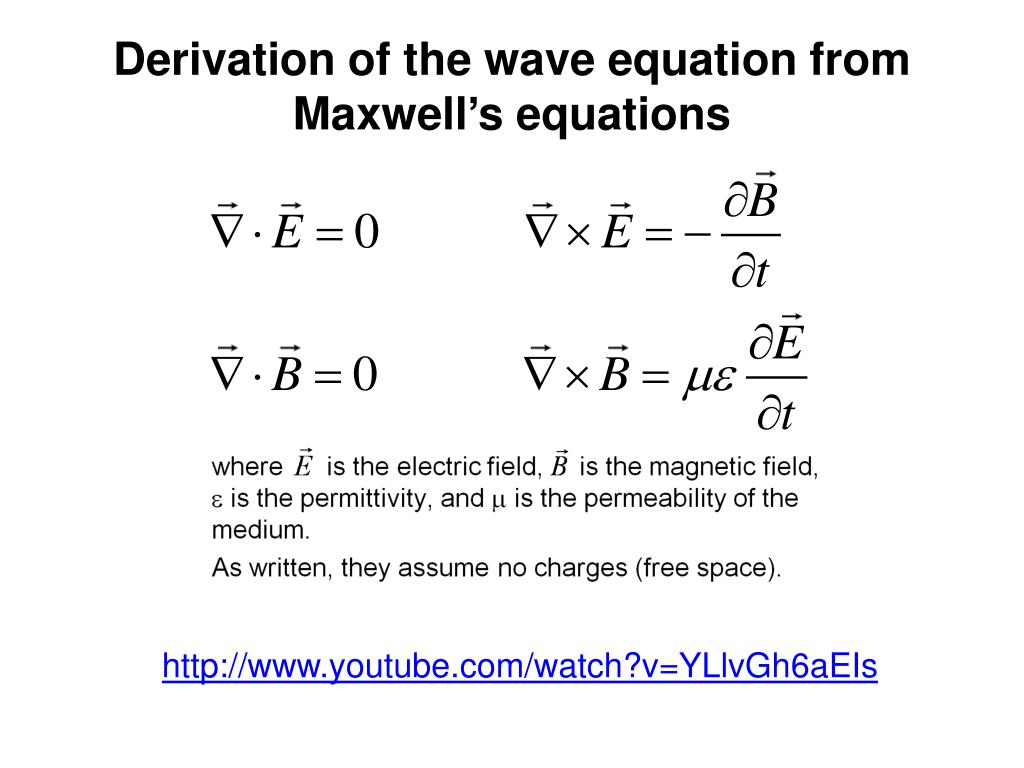
Ppt Chapter 4 Wave Equations Powerpoint Presentation Free Download Light is a pair of 3d vector fields. xx ˆ yy ˆ zz ˆ in 3d space. a light wave has both electric and magnetic 3d vector fields. in this illustration, the vectors of the two fields are only shown at a few selected locations, equally spaced along a line. but the fields are defined at every point (x,y,z). the equations of optics are maxwell’s. The scalar wave equation can then be written as. 2u −n2e0μ0∂2u ∂t2 = 0. 2 u − n 2 e 0 μ 0 ∂ 2 u ∂ t 2 = 0. the speed of light in matter is. c n = 1 (eμ0)1 2. c n = 1 (e μ 0) 1 2. this page titled 1.5: the scalar and vector wave equation is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by. Derivation of electromagnetic wave equation now let’s see how we can combine the differential forms of maxwell’s equations to derive a set of differential equations (wave equations) for the electric and magnetic fields. let’s assume we solve these equations in a region without any electric charges present (ρ=0) or any currents (j=0). 1 the dot product of a and b can be defined as a • b = ax bx ayb y azb z = a b cos θ , where θ is the angle between the two vectors. 2 the cross product of zˆ (a x by − ayb x ) ; its magnitude is a. and b can be defined as a × b = xˆ (ay bz − a z by ) yˆ (a z bx − axb z ) . b sin θ .

The Vectorial Part Of The Wave Equation Derived From The Maxwell Derivation of electromagnetic wave equation now let’s see how we can combine the differential forms of maxwell’s equations to derive a set of differential equations (wave equations) for the electric and magnetic fields. let’s assume we solve these equations in a region without any electric charges present (ρ=0) or any currents (j=0). 1 the dot product of a and b can be defined as a • b = ax bx ayb y azb z = a b cos θ , where θ is the angle between the two vectors. 2 the cross product of zˆ (a x by − ayb x ) ; its magnitude is a. and b can be defined as a × b = xˆ (ay bz − a z by ) yˆ (a z bx − axb z ) . b sin θ .

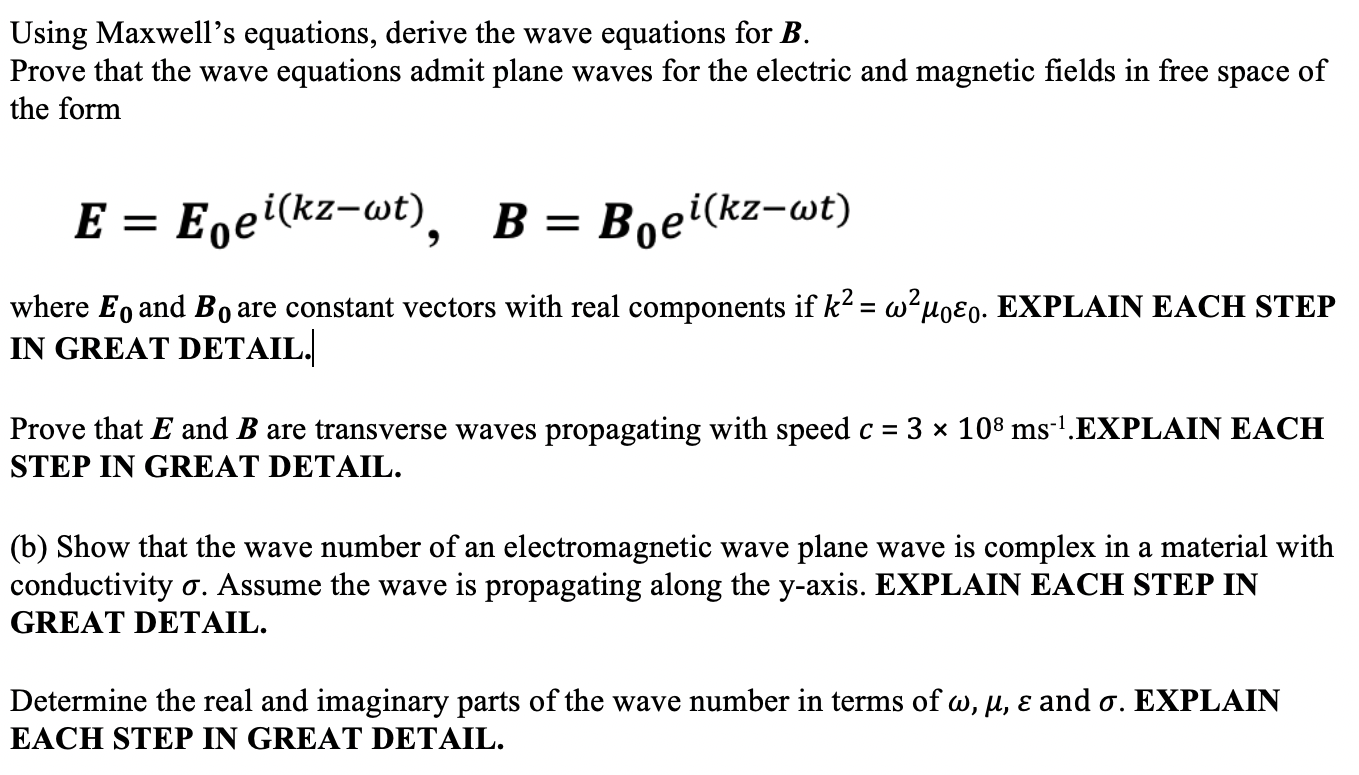
Solved Using Maxwell S Equations Derive The Wave Equations Chegg

Ppt Maxwell S Equations Powerpoint Presentation Free Download Id