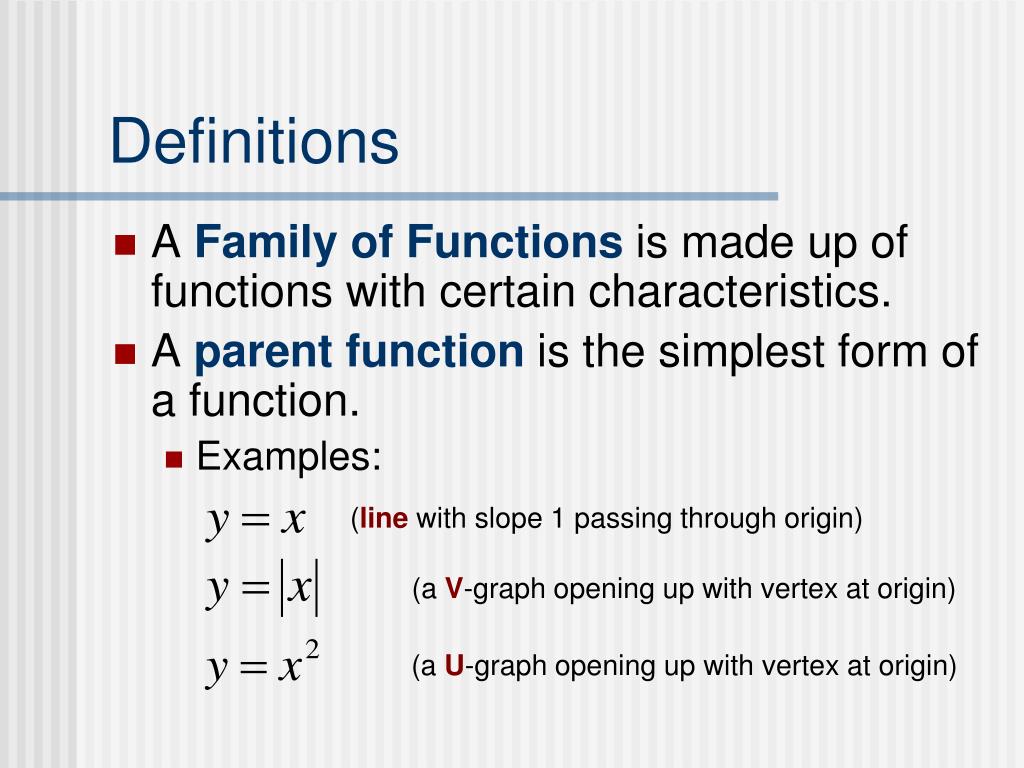
The Family Of Functions

How To Identify Families Of Functions Study Our first family of functions is called linear functions. the "parent" function for this family is. f(x) = x. as you may have guessed, these are the type of functions whose graphs are a straight line. the graph of f(x) = x looks like. graphs in this family may have different slants or be in a different location on the coordinate plane, but what. The power function family can be extended to create polynomials and rational functions. the exponential function family: \(f(x)=e^{x}\) the exponential function family is one of the first functions you see where \(x\) is not the base of the exponent. this function eventually grows much faster than any power function. \(f(x)=2^{x}\) is a very.

Families Of Functions Transformations And Symmetry Part 3 Families A function family is a group of functions with the same degree and that look the same, even with different numbers. the most common function families are: linear (degree of 1,. A ll the functions listed so far can be grouped together into an even larger function family called the power function family. the power function family: f (x) = c x a. the power function family has two parameters. the parameter c is a vertical scale factor. the parameter a controls everything about the shape. the reason why all the functions. And suppose that we're asked to find what functions in the family have a local minimum at (1, 1). a good place to start with this type of question is to graph the function for some values of the parameters. this is done in the figures to the right. what do you notice? are we going to get a local minimum at (1, 1) with these parameter choices? nope!. Activity 2.3.3. consider the two parameter family of functions of the form h(x) = a(1 − e − bx), where a and b are positive real numbers. find the first derivative and the critical numbers of h. use these to construct a first derivative sign chart and determine for which values of x the function h is increasing and decreasing.
Ppt 2 6 Families Of Functions P 93 Powerpoint Presentation Free And suppose that we're asked to find what functions in the family have a local minimum at (1, 1). a good place to start with this type of question is to graph the function for some values of the parameters. this is done in the figures to the right. what do you notice? are we going to get a local minimum at (1, 1) with these parameter choices? nope!. Activity 2.3.3. consider the two parameter family of functions of the form h(x) = a(1 − e − bx), where a and b are positive real numbers. find the first derivative and the critical numbers of h. use these to construct a first derivative sign chart and determine for which values of x the function h is increasing and decreasing. A family of functions is a function that changes depending on the particular values of certain parame ters. an example of such a family of functions would be something like f(x) = a(x b)2 c. depending on the values of a, b, and c, this function could take multiple forms. the collection of all such functions is referred to as a family. Example 3.2.1 3.2. 1. consider the two parameter family of functions given by g (x) = axe−bx , where a and b are positive real numbers. fully describe the behavior of a typical member of the family in terms of a and b, including the location of all critical numbers, where g is increasing, decreasing, concave up, and concave down, and the long.

Parent Functions A family of functions is a function that changes depending on the particular values of certain parame ters. an example of such a family of functions would be something like f(x) = a(x b)2 c. depending on the values of a, b, and c, this function could take multiple forms. the collection of all such functions is referred to as a family. Example 3.2.1 3.2. 1. consider the two parameter family of functions given by g (x) = axe−bx , where a and b are positive real numbers. fully describe the behavior of a typical member of the family in terms of a and b, including the location of all critical numbers, where g is increasing, decreasing, concave up, and concave down, and the long.

Comments are closed.