The Arithmetic Series Part 1 14

The Arithmetic Series Part 1 14 Youtube More lessons: mathandscience twitter: twitter jasongibsonmath in this lesson, we will learn about the arithmetic series and how i. Once we find the value for \large {n}, we will substitute that into the arithmetic series formula together with the first and last terms to find the sum of the given arithmetic series. finally, we have all the values that we need to calculate the sum of the given series which are \large {n=37} 37 \large {a 1} = 7 8 \large {a n} = 187 187.

Arithmetic Series Solutions Examples Videos Worksheets Games Our arithmetic sequence calculator can also find the sum of the sequence (called the arithmetic series) for you. trust us, you can do it by yourself — it's not that hard! look at the first example of an arithmetic sequence: 3, 5, 7, 9, 11, 13, 15, 17, 19, 21. we could sum all of the terms by hand, but it is not necessary. 13 used when referring to an arithmetic sequence. 14 the constant \(d\) that is obtained from subtracting any two successive terms of an arithmetic sequence; \(a {n} a {n 1}=d\). 15 the terms between given terms of an arithmetic sequence. 16 the sum of the terms of an arithmetic sequence. Definition 12.3.1. an arithmetic sequence is a sequence where the difference between consecutive terms is always the same. the difference between consecutive terms, a {n} a {n 1}, is d, the common difference, for n greater than or equal to two. figure 12.2.1. This means that $3 8 13 … 68 73 = 570$ and we’ve demonstrated how to use the two important formulas for the arithmetic series. example 1. in the arithmetic series, $ 4 2 0 2 4 …$, find the sum of the first $40$ terms. solution.
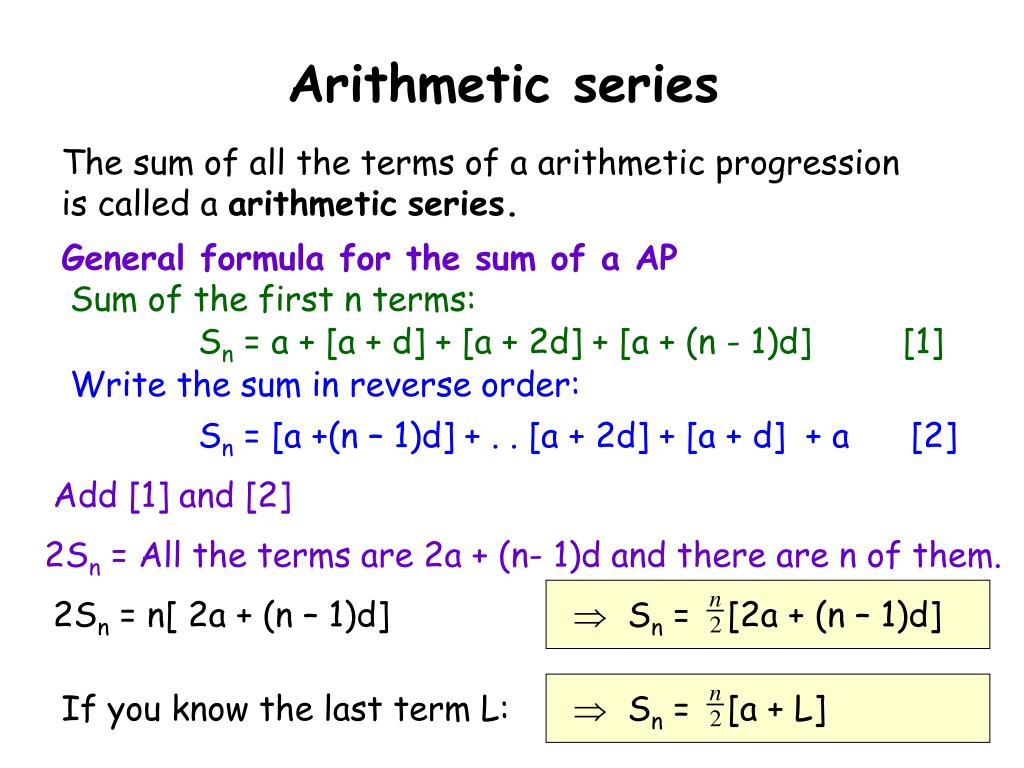
Ppt Arithmetic Series Powerpoint Presentation Free Download Id 5250363 Definition 12.3.1. an arithmetic sequence is a sequence where the difference between consecutive terms is always the same. the difference between consecutive terms, a {n} a {n 1}, is d, the common difference, for n greater than or equal to two. figure 12.2.1. This means that $3 8 13 … 68 73 = 570$ and we’ve demonstrated how to use the two important formulas for the arithmetic series. example 1. in the arithmetic series, $ 4 2 0 2 4 …$, find the sum of the first $40$ terms. solution. The problem tells us that there is an arithmetic sequence with two known terms which are 8 {a 5} = – 8 –8 {a {25}} = 72 25 72. the first step is to use the information of each term and substitute its value in the arithmetic formula. we have two terms so we will do it twice. this is wonderful because we have two equations and two unknown. This sequence is the same as the one that is given in example 2. there we found that a = 3, d = 5, and n = 50. so we have to find the sum of the 50 terms of the given arithmetic series. s n = n 2 [a 1 a n] s 50 = [50 ( 3 248)] 2 = 6275. answer: the sum of the given arithmetic sequence is 6275.

Arithmetic Series Ppt The problem tells us that there is an arithmetic sequence with two known terms which are 8 {a 5} = – 8 –8 {a {25}} = 72 25 72. the first step is to use the information of each term and substitute its value in the arithmetic formula. we have two terms so we will do it twice. this is wonderful because we have two equations and two unknown. This sequence is the same as the one that is given in example 2. there we found that a = 3, d = 5, and n = 50. so we have to find the sum of the 50 terms of the given arithmetic series. s n = n 2 [a 1 a n] s 50 = [50 ( 3 248)] 2 = 6275. answer: the sum of the given arithmetic sequence is 6275.

Arithmetic Series Part 1 Youtube

Arithmetic Series By Maths Support Centre Tpt

Comments are closed.