Structure Function Analysis Of Annular Zernike Polynomials Ppt Download 13 finding the structure functions for each obscuration ratio and for each zernike polynomial create the surface using a matrix of points (>100 x 100) for each point on the surface find a second point a distance r away for a number of different angles (>15) then figure out if the second position also lies in the pupil if the second point is also in the pupil, then add the squared difference of. Conclusion • zernike polynomials can be used to describe the residual surface errors in telescope mirror due to polishing and support errors • using the conversions to structure functions from zernike polynomials developed here, a structure function for the mirror can be found • structure functions are useful specifications for.
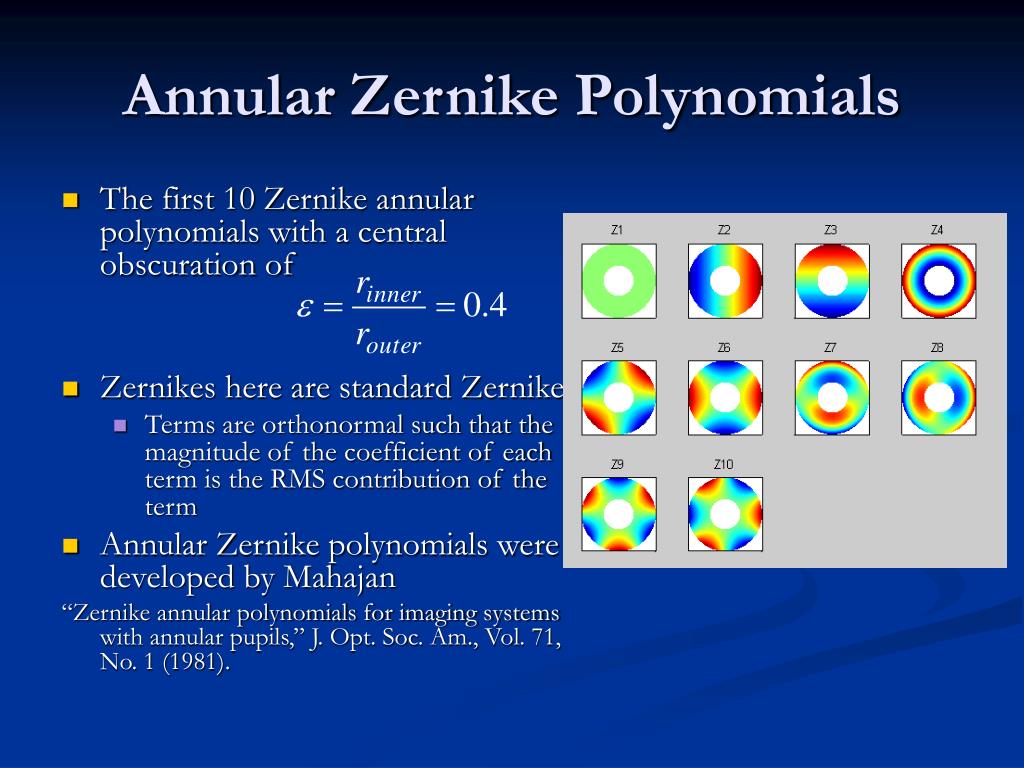
Ppt Structure Function Analysis Of Annular Zernike Polynomials Download presentation structure function analysis of annular zernike polynomials anastacia m. hvisc*, james h. burge college of optical sciences the university of arizona * amh 21@email. arizona. edu. Structure function analysis of annular zernike polynomials. structure function analysis of annular zernike polynomials. anastacia m. hvisc*, james h. burge college of optical sciences the university of arizona * [email protected]. outline. my work describes a method of converting annular zernike polynomials into structure functions. 5. what are zernike polynomials? set of basis shapes or topographies of a surface similar to modes of a circular drum head real surface is constructed of linear combination of basis shapes or modes polynomials are a product of a radial and azimuthal part radial orders are positive, integers (n), 0, 1,2, 3, 4, azimuthal indices (m) go from n to. The second property of zernike polynomials is that the radial function must be a polynomial in r of degree 2n and contain no power of r less than m. the third property is that r[r] must be even if m is even, and odd if m is odd. the radial polynomials can be derived as a special case of jacobi polynomials, and tabulated as r@n, m, rd. their.
Ppt Structure Function Analysis Of Annular Zernike Polynomials 5. what are zernike polynomials? set of basis shapes or topographies of a surface similar to modes of a circular drum head real surface is constructed of linear combination of basis shapes or modes polynomials are a product of a radial and azimuthal part radial orders are positive, integers (n), 0, 1,2, 3, 4, azimuthal indices (m) go from n to. The second property of zernike polynomials is that the radial function must be a polynomial in r of degree 2n and contain no power of r less than m. the third property is that r[r] must be even if m is even, and odd if m is odd. the radial polynomials can be derived as a special case of jacobi polynomials, and tabulated as r@n, m, rd. their. For a given total power, the central value of the image of a point object is maximum for uniform amplitude and phase of the wave at the exit pupil. strehl ratio is the ratio of central irradiances with and without phase amplitude variations. s =. aberrated central irraidance aberration free central irraidance. i ( 0 ). Zernike polynomials are a useful set of functions for representing surface form and wavefronts on circular domains. the normalized version of the zernikes gives a direct quality metric in the form of variance. many different schemes and definitions exists, so be careful when comparing results from different sources.
Comments are closed.