Specific Internal Energy Thermodynamic Properties

Specific Internal Energy Thermodynamic Properties The specific internal energy (u) of a substance is its internal energy per unit mass. it equals the total internal energy (u) divided by the total mass (m). example: determine the specific internal energy of 12 lbm of steam if the total internal energy is 23,000 btu. solution:. The internal energy and specific internal energy are important properties for the analysis of closed systems, which will be explained in detail in chapters 4 6. 2.2.5 enthalpy and specific enthalpy enthalpy is an important thermodynamic property for the analysis of open systems or control volumes, where mass transfer is always accompanied by.
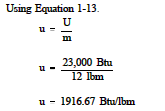
Specific Internal Energy Thermodynamic Properties Pressure , temperature , specific volume , specific internal energy , specific enthalpy , and specific entropy are intensive properties. let us consider a container of air at 101 kpa and 20 o c. if the container is divided into two compartments and all other conditions remain unchanged, see figure 1.3.1 , the air in each compartment is still at. The total energy of a system may consist of internal energy, kinetic energy, potential energy, and other forms of energy. for a system free of magnetic, electric, and surface tension effects, its total energy and corresponding specific energy can be expressed as. e = u ke pe. e = u ke pe. where , and represent the total energy, internal. The internal energy of an ideal gas is proportional to its amount of substance (number of moles) and to its temperature. where is the isochoric (at constant volume) molar heat capacity of the gas; is constant for an ideal gas. the internal energy of any gas (ideal or not) may be written as a function of the three extensive properties. Chapter 4. the first law for closed systems. we have already encountered work and heat as two forms of energy. in order to complete the picture for closed systems, another type of energy, internal energy, must be taken into consideration. these three forms of energy will later be combined in the first law of thermodynamics.

Change In Internal Energy Using Specific Heat In 3 Minutes Youtube The internal energy of an ideal gas is proportional to its amount of substance (number of moles) and to its temperature. where is the isochoric (at constant volume) molar heat capacity of the gas; is constant for an ideal gas. the internal energy of any gas (ideal or not) may be written as a function of the three extensive properties. Chapter 4. the first law for closed systems. we have already encountered work and heat as two forms of energy. in order to complete the picture for closed systems, another type of energy, internal energy, must be taken into consideration. these three forms of energy will later be combined in the first law of thermodynamics. The internal energy and specific internal energy are important properties for the analysis of closed systems, which will be explained in detail in chapters 4 6. 2.2.5 enthalpy and specific enthalpy enthalpy is an important thermodynamic property for the analysis of open systems or control volumes, where mass transfer is always accompanied by. 4.1.1 using thermodynamic tables to determine specific internal energy u. for pure substances with available thermodynamic tables, the specific internal energy can be read from the thermodynamic tables, then the internal energy can be found from [latex]u = mu[ latex] where [latex]m[ latex]: mass of a system, in kg [latex]u[ latex]: internal.

Comments are closed.