Solving Polynomial Equations Of Higher Degree Part 1
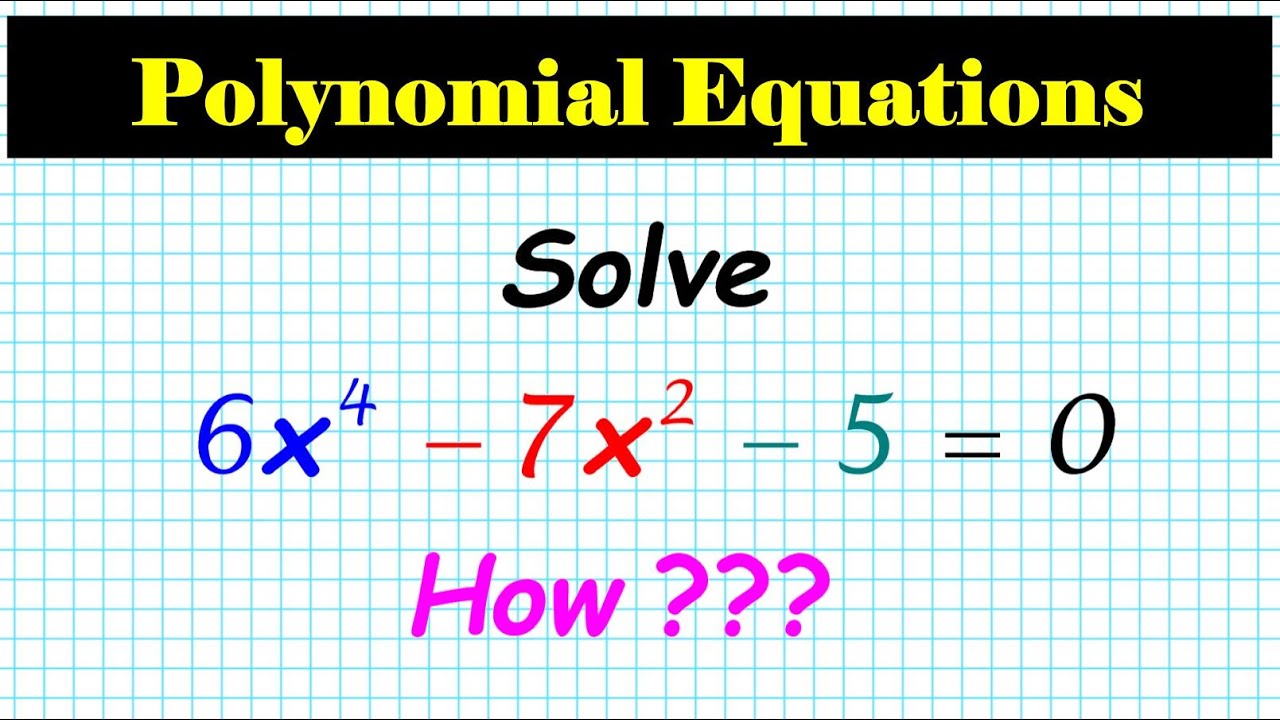
How To Solve Polynomial Equations Of Higher Degree Precalculus Vtext The solutions are x=0, x= 4, and x= 2. identify polynomials that act like a quadratic. you likely already know how to solve second degree polynomials, in the form . you can solve some higher degree polynomials the same way, if they're in the form . here are a couple examples: x = ±√ (2 3). To solve a polynomial equation write it in standard form (variables and canstants on one side and zero on the other side of the equation). factor it and set each factor to zero. solve each factor. the solutions are the solutions of the polynomial equation. a polynomial equation is an equation formed with variables, exponents and coefficients.

Solving Polynomial Equations Of Higher Degree Part 1 Youtube In fact, it is known that only a very small part of polynomials of degree $\ge 5$ admit a solution formula using the operations listed above. nevertheless, finding solutions to polynomial formulas is quite easy using numerical methods, e.g., newton's method. these methods are independent of the degree of the polynomial. These are called the roots (or zeros) of the polynomial equation f(x) = 0. things to do. use the "degree" and − buttons below the graph to change the degree of the polynomial. use the "a n slider" below the graph to move the graph up and down. observe that: a degree 1 polynomial has at most 1 root; a degree 2 polynomial has at most 2 roots. The techniques used to solve polynomial equations of degree 3 or higher are not as straightforward as those used to solve linear equations and quadratic equations. the next example shows how the factor theorem can be used to solve a third degree polynomial equation. example 5 solving a third degree equation suppose the equationx3 4x2 17x 60 0. We may be able to solve using basic algebra: example: 2x 1. 2x 1 is a linear polynomial: the graph of y = 2x 1 is a straight line. it is linear so there is one root. use algebra to solve: a "root" is when y is zero: 2x 1 = 0. subtract 1 from both sides: 2x = −1. divide both sides by 2: x = −1 2.

How To Solve Higher Degree Polynomials With Pictures Wikihow The techniques used to solve polynomial equations of degree 3 or higher are not as straightforward as those used to solve linear equations and quadratic equations. the next example shows how the factor theorem can be used to solve a third degree polynomial equation. example 5 solving a third degree equation suppose the equationx3 4x2 17x 60 0. We may be able to solve using basic algebra: example: 2x 1. 2x 1 is a linear polynomial: the graph of y = 2x 1 is a straight line. it is linear so there is one root. use algebra to solve: a "root" is when y is zero: 2x 1 = 0. subtract 1 from both sides: 2x = −1. divide both sides by 2: x = −1 2. If you’re solving an equation, you can throw away any common constant factor. (technically, you’re dividing left and right sides by that constant factor.) but if you’re factoring a polynomial, you must keep the common factor. example: to solve 8 x ² 16 x 8 = 0, you can divide left and right by the common factor 8. By m. bourne. this chapter discusses methods for solving higher degree polynomial equations. in the study of polynomial equations, the most important thing is to understand what "solution of an equation" means. for equations of higher degree, allow for many solutions. the maximum number of solutions you can get is the degree of the polynomial.

Solving Polynomials Equations Of Higher Degree A Plus Topper If you’re solving an equation, you can throw away any common constant factor. (technically, you’re dividing left and right sides by that constant factor.) but if you’re factoring a polynomial, you must keep the common factor. example: to solve 8 x ² 16 x 8 = 0, you can divide left and right by the common factor 8. By m. bourne. this chapter discusses methods for solving higher degree polynomial equations. in the study of polynomial equations, the most important thing is to understand what "solution of an equation" means. for equations of higher degree, allow for many solutions. the maximum number of solutions you can get is the degree of the polynomial.

Solving High Degree Polynomial Equations Real Roots Mathgotserved

Comments are closed.