Solved Prove That 1 2 2 3 Is A Basis For The Crazy Chegg

Solved Prove That 1 2 2 3 Is A Basis For The Crazy Chegg Here’s the best way to solve it. prove that { (1, 2), (2, 3)} is a basis for the crazy vector space c. recall: the crazy vector space is defined as c = { {x 1, x 2)|x 1, x 2 c} with vector addition and scalar multiplication defined, respectively, by (x 1, x 2) (y 1, y 2) = (x 1 y 1 1, x 2 y 2 1) and a (x 1, x 2) = (alpha x 1 alpha. Here’s the best way to solve it. please …. c80. prove that { (1, 2), (2, 3)} is a basis for the crazy vector space c ). (example cvs. example cvs. the crazy vector space. set c = { (x1, x2) | 21, 22 e c}. equality (x1, x2) = (y1, y2) if and only if x1 = yı and x2 = 42 vector addition (21, 22) (y1, y2) = (21 41 1, 22 y2 1). a (x1, x2.

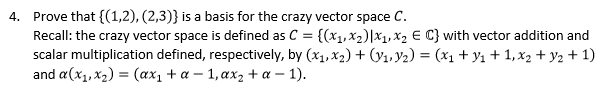
Solved Prove That 1 2 2 3 Is A Basis For The Crazy Chegg So they are linearly independent. since you have 3 vectors in r3 which are linearly independent then they form a basis. – maesumi. jun 16, 2013 at 2:26. a better way of stating the problem: a1(1,0.−1) a2(2,5,1) a3(0,−4,3)=0 if and only if a1, a2, and a3 are 0 this means linear independence of those vectors. – benkoshy. To prove the implication. p(k) ⇒ p(k 1) (3.6.3) (3.6.3) p (k) ⇒ p (k 1) in the inductive step, we need to carry out two steps: assuming that p(k) p (k) is true, then using it to prove p(k 1) p (k 1) is also true. so we can refine an induction proof into a 3 step procedure: verify that p(a) p (a) is true. Example 4.7.3 determine the components of the vector v = (1,7) relative to the ordered basis b = {(1,2),(3,1)}. solution: if we let v1 = (1,2)and 2 = 3 , then since these vectors are not collinear, b ={v1,v2} is a basis for r2. we must determine constants c1,c2 such that c1v1 c2v2 = v. we write c1(1,2) c2(3,1) = (1,7). this requires that c1. 2 1 21 10 = − s, so we solve this inhomogeneous system as 2 1 1 10 2 21 7 01 3 1 0 2 00 0 − −→ − . therefore [ ] 2 3 = − x b, i.e. x v v= −23 12, and you can easily verify that this is the case. it’s worth noting that had we chosen x∉v, the system would have been inconsistent.

Solved 1 2 3 Chegg Example 4.7.3 determine the components of the vector v = (1,7) relative to the ordered basis b = {(1,2),(3,1)}. solution: if we let v1 = (1,2)and 2 = 3 , then since these vectors are not collinear, b ={v1,v2} is a basis for r2. we must determine constants c1,c2 such that c1v1 c2v2 = v. we write c1(1,2) c2(3,1) = (1,7). this requires that c1. 2 1 21 10 = − s, so we solve this inhomogeneous system as 2 1 1 10 2 21 7 01 3 1 0 2 00 0 − −→ − . therefore [ ] 2 3 = − x b, i.e. x v v= −23 12, and you can easily verify that this is the case. it’s worth noting that had we chosen x∉v, the system would have been inconsistent. For example, $(1 x)^2 = 1 2x 1 x^2$, and so the coefficients $1, 2, 1$ translate into the column vector $ \begin{bmatrix} 1 \\ 2 \\ 1 \end{bmatrix}$. because $\dim \mathbf{p} 2 = 3$, this set is a basis if and only if these three vectors are linearly independent. Prove that { (1, 2), (2, 3) } is a basis for the crazy vector space c. your solution’s ready to go! our expert help has broken down your problem into an easy to learn solution you can count on.