Solved Let A 2 1 1 1 0 2 1 0 1 B 2 2 0 1 2 2 4 Chegg

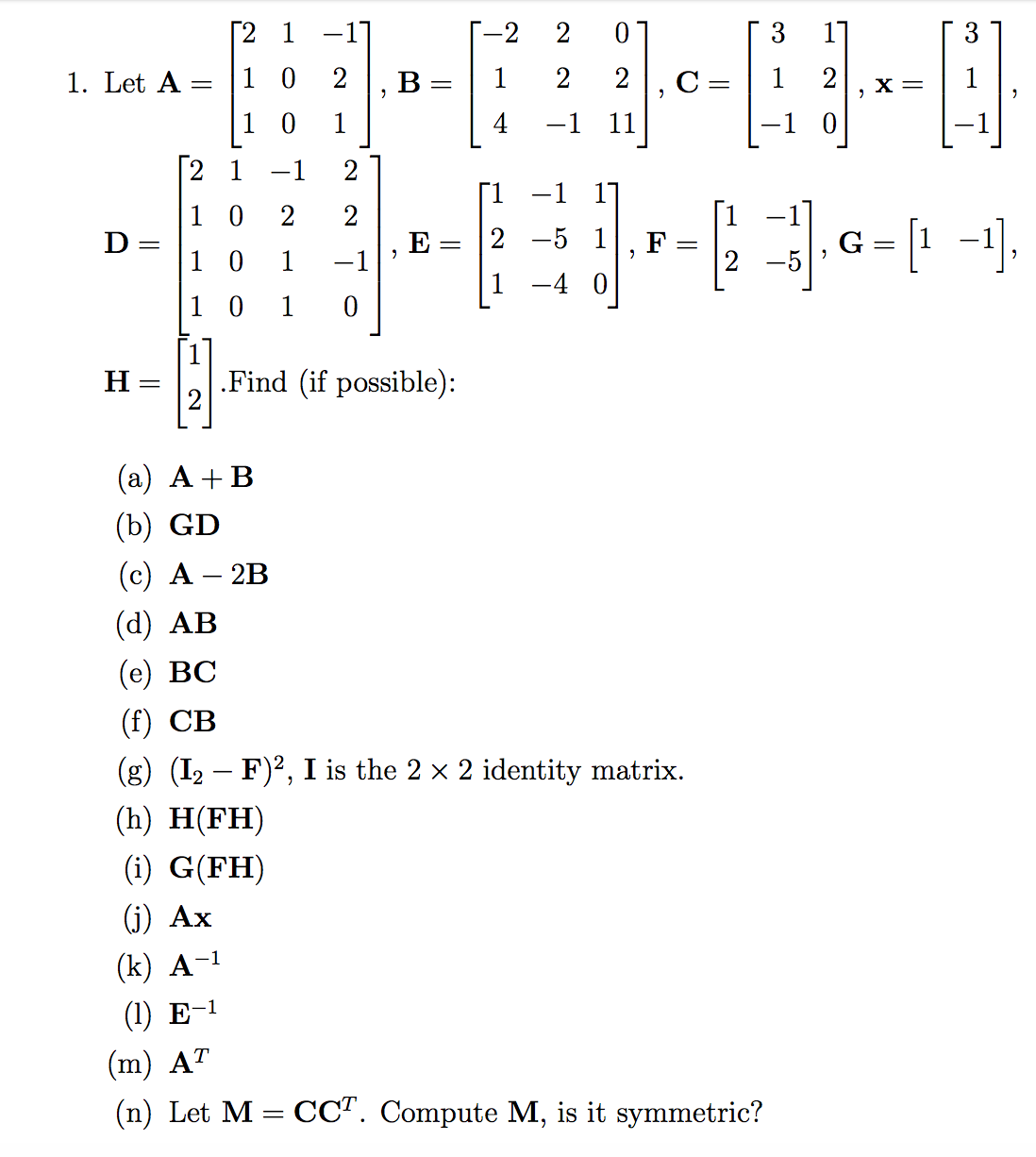
Solved Let A 2 1 1 1 0 2 1 0 1 B 2 2 0 1 2 2 4 Chegg Step 1. (1) if the vector w can be expressed as a linear combination of the vectors v 1, v 2, v 3 then we let v 1 = [1 0 1], v 2 = [2 1 3], v 3 = [4 3 0], and w = [4 2 6]. (1) is w in span {v 1, v 2, v 3}; (2) find a basis for span {v 1, v 2, v 3}. let a = [ 3 1 2 6 2 4 1 2 5 1 3 8], w = [1 1 2 1] and v = [ 1 0 2]. Free math problem solver answers your algebra homework questions with step by step explanations.

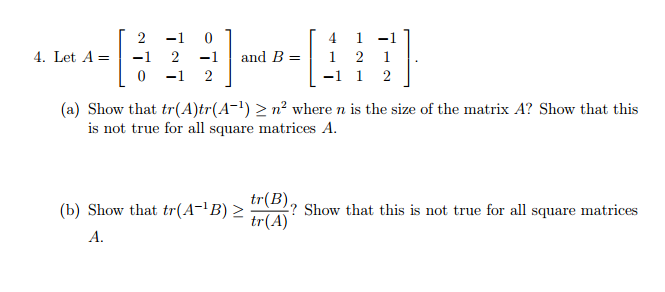
Solved Let A 2 1 0 1 2 1 0 0 1 2 And B 4 1 1 Chegg Muhammad gave you a nice way using partial derivative so i'll just throw out here another way without using derivatives. the shortest distance to a plane create angle of $\pi 2$, so let's create the vector $\vec v=\begin{bmatrix}1 a\\2 a\\3 b\\4 b\end{bmatrix}$, this vector is the vector from the point $(1,2,3,4)$ to an arbitrary point on the plane. Explore math with our beautiful, free online graphing calculator. graph functions, plot points, visualize algebraic equations, add sliders, animate graphs, and more. 3. (b) the only solution of bx =. 1 0 is x = 2. .31 0 0 solution: (a) a = , (b) impossible because b would have to be a 2 by 3 matrix 1 2 3 without free variables, i.e., f rank 3. however, the rank of a matrix can not be greater than the number of rows, in this example it could be. t m. Key idea 2.5.1: solving ax = b. let a be an n × n matrix, where the reduced row echelon form of a is i. to solve the matrix equation ax = b for x, form the augmented matrix [a b]. put this matrix into reduced row echelon form. it will be of the form [i x], where x appears in the columns where b once was.

Solved Let A And B Find Each Chegg 3. (b) the only solution of bx =. 1 0 is x = 2. .31 0 0 solution: (a) a = , (b) impossible because b would have to be a 2 by 3 matrix 1 2 3 without free variables, i.e., f rank 3. however, the rank of a matrix can not be greater than the number of rows, in this example it could be. t m. Key idea 2.5.1: solving ax = b. let a be an n × n matrix, where the reduced row echelon form of a is i. to solve the matrix equation ax = b for x, form the augmented matrix [a b]. put this matrix into reduced row echelon form. it will be of the form [i x], where x appears in the columns where b once was. Question: problem 5. in this problem, we practice change o f order of integration in triple integrals. let f be a continuous function. show the following relation:∫0x∫0y∫0zf (t)dtdzdy=12∫0x (x t)2f (t)dtwhere x≥0,y≥0, and z≥0.problem 2. let f (x,y) be defined on a bounded domain d in the xy plane. the graph of f. To find the transition matrix p from b ′ to b, express the vectors in b ′ as linear combinations let b = {0, 1, 1), (1, 0, 1), (1, 1, 0)) and b' = { (1, 0, 0), (0, 1, 0), (0, 0, 1)) be bases for r, and let 1 1 a 2 ninun ninn 2 1 be the matrix for t: rr relative to b. (a) find the transition matrix p from b' to b. p dll 100 it (b.