Solve Higher Order Polynomial Equations

Solving Higher Order Polynomial Equations Youtube The solutions are x=0, x= 4, and x= 2. identify polynomials that act like a quadratic. you likely already know how to solve second degree polynomials, in the form . you can solve some higher degree polynomials the same way, if they're in the form . here are a couple examples: x = ±√ (2 3). To solve a polynomial equation write it in standard form (variables and canstants on one side and zero on the other side of the equation). factor it and set each factor to zero. solve each factor. the solutions are the solutions of the polynomial equation. a polynomial equation is an equation formed with variables, exponents and coefficients.
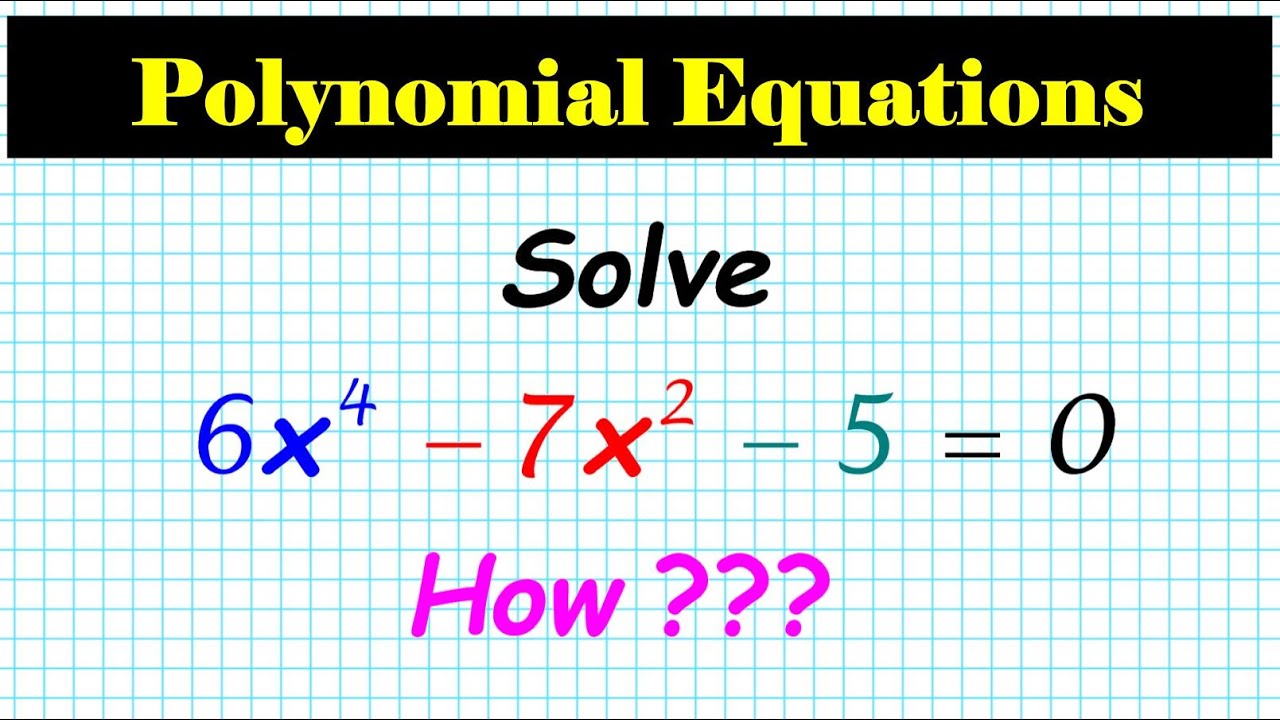
How To Solve Polynomial Equations Of Higher Degree Precalculus Vtext The question was: how to solve a polynomial of degree n. knowing to where to find the solution is an answer to the question cited. in general, there are no exact solutions for solving polynomials in terms of radicals, that is in terms of square roots, cube roots , etc., for polynomials of degree five or greater, and the solutions are. By m. bourne. this chapter discusses methods for solving higher degree polynomial equations. in the study of polynomial equations, the most important thing is to understand what "solution of an equation" means. for equations of higher degree, allow for many solutions. the maximum number of solutions you can get is the degree of the polynomial. 1. set up the division. draw an inverted division bracket as shown below. outside the bracket, write the value of the zero; inside the bracket, write the coefficients of the polynomial that you are factoring in order from higher order terms to lower order terms (include zero valued coefficients also). 2. It has degree of 2 since the quadratic polynomial has degree 2 (i.e. highest exponent of all monomials in the polynomial is 2: \(x^2\)). recall the methods we can use to solve quadratic equations such as factoring or using the quadratic formula (review these on the solving quadratic equations page). these only work for solving quadratic.

How To Solve Higher Degree Polynomials With Pictures Wikihow 1. set up the division. draw an inverted division bracket as shown below. outside the bracket, write the value of the zero; inside the bracket, write the coefficients of the polynomial that you are factoring in order from higher order terms to lower order terms (include zero valued coefficients also). 2. It has degree of 2 since the quadratic polynomial has degree 2 (i.e. highest exponent of all monomials in the polynomial is 2: \(x^2\)). recall the methods we can use to solve quadratic equations such as factoring or using the quadratic formula (review these on the solving quadratic equations page). these only work for solving quadratic. If you’re solving an equation, you can throw away any common constant factor. (technically, you’re dividing left and right sides by that constant factor.) but if you’re factoring a polynomial, you must keep the common factor. example: to solve 8 x ² 16 x 8 = 0, you can divide left and right by the common factor 8. Polynomials. recall our definitions of polynomials from chapter 1. a polynomial is function that can be written as. each of the constants are called coefficients and can be positive, negative, or zero, and be whole numbers, decimals, or fractions. a term of the polynomial is any one piece of the sum, that is any .

Solve Higher Order Polynomial Equations By Scientific Calculator Youtube If you’re solving an equation, you can throw away any common constant factor. (technically, you’re dividing left and right sides by that constant factor.) but if you’re factoring a polynomial, you must keep the common factor. example: to solve 8 x ² 16 x 8 = 0, you can divide left and right by the common factor 8. Polynomials. recall our definitions of polynomials from chapter 1. a polynomial is function that can be written as. each of the constants are called coefficients and can be positive, negative, or zero, and be whole numbers, decimals, or fractions. a term of the polynomial is any one piece of the sum, that is any .

Comments are closed.