Solutions Of First Order Odes Study Notes Differential Equations

Solution Differential Equations For Engineering Chapter 1 First Order First order odes 5 intuitively, the ode wants to push solutions towards the line y= 1=t:note that y ‘ is not a solution to the ode, unlike the equilibrium points of the rst example. 0 2 4 6 0 0.5 1 1.5 2 0 2 4 6 0 0.5 1 1.5 2 3. separable equations even rst order odes are complicated enough that exact solutions are not easy to obtain. Here is a set of notes used by paul dawkins to teach his differential equations course at lamar university. included are most of the standard topics in 1st and 2nd order differential equations, laplace transforms, systems of differential eqauations, series solutions as well as a brief introduction to boundary value problems, fourier series and partial differntial equations.
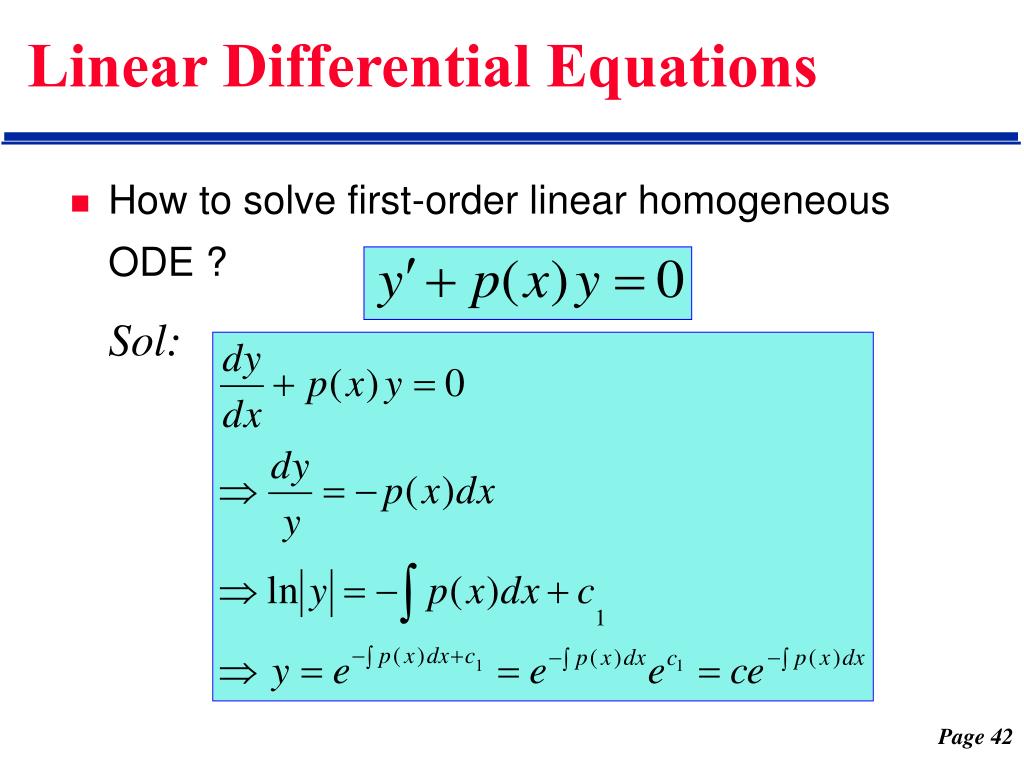
Ppt Chap 1 First Order Differential Equations Powerpoint Presentation Equivalence of (1.4) and (1.7) means starting from a solution of either of these ode we can produce a solution of the other. this is a simple calculation and is left as an exercise. note that the first order system for z consists of n equations. this n is the order of (1.4). exercise 1.10 define higher order systems of ordinary differential. First order ode s we will now discuss different methods of solutions of first order odes. the first type of such odes that we will consider is the following: definition separable variables: a first order differential equation of the form dy dx = g(x)h(y) is called separable or to have separable variables. such odes can be solved by direct. 1.1 ordinary differential equation (ode) 1 1.2 solution 1 1.3 order n of the de 2 1.4 linear equation: 2 1.5 homogeneous linear equation: 3 1.6 partial differential equation (pde) 3 1.7 general solution of a linear differential equation 3 1.8 a system of ode’s 4 2 the approaches of finding solutions of ode 5 2.1 analytical approaches 5. 18.03 differential equations, supplementary notes ch. 3. 3. solutions of first order linear odes. 3.1. homogeneous and inhomogeneous; superposition. a first order linear equation is homogeneous if the right hand side is zero: (1) x ̇ p(t)x = 0 . homogeneous linear equations are separable, and so the solution can be expressed in terms of an.

Solutions Of First Order Odes Study Notes Differential Equations 1.1 ordinary differential equation (ode) 1 1.2 solution 1 1.3 order n of the de 2 1.4 linear equation: 2 1.5 homogeneous linear equation: 3 1.6 partial differential equation (pde) 3 1.7 general solution of a linear differential equation 3 1.8 a system of ode’s 4 2 the approaches of finding solutions of ode 5 2.1 analytical approaches 5. 18.03 differential equations, supplementary notes ch. 3. 3. solutions of first order linear odes. 3.1. homogeneous and inhomogeneous; superposition. a first order linear equation is homogeneous if the right hand side is zero: (1) x ̇ p(t)x = 0 . homogeneous linear equations are separable, and so the solution can be expressed in terms of an. Session overview. in this session we will start our study of linear equations, which is probably the most important class of differential equations. we will introduce the ideas and terminology of superposition, systems, input and response which will be used for the rest of the course. After that we will focus on first order differential equations. we’ll start by defining differential equations and seeing a few well known ones from science and engineering. very quickly we will learn about the three main ways of approaching ode’s: analytic (in symbols), geometric (with pictures and graphs), and numerical (with the computer).

First Order Differential Equations Teaching Resources Session overview. in this session we will start our study of linear equations, which is probably the most important class of differential equations. we will introduce the ideas and terminology of superposition, systems, input and response which will be used for the rest of the course. After that we will focus on first order differential equations. we’ll start by defining differential equations and seeing a few well known ones from science and engineering. very quickly we will learn about the three main ways of approaching ode’s: analytic (in symbols), geometric (with pictures and graphs), and numerical (with the computer).

First Order Differential Equation Solutions Types Examples

Comments are closed.