Solution Differential Equations For Engineering Chapter 1 First Order

Solution Differential Equations For Engineering Chapter 1 First Order 2 chapter 1. introduction and first order equations and the the combination 2f(x) = 2cexp(2x) appearing on the right hand side, and checking that they are indeed equal for each value of x. the equation(1.1)is adifferential equation. thefunction f(x) = cexp(2x) satisfying it will be referred to as a solution of the given differential equation. We should be particularly careful about existence and uniqueness of the solution of the initial value problem if we solve the problem numerically using computers. example 1: ode of the 1st order: ’ – ’ 0 initial condition: general solution: –– 2, family of straight lines. let’s find c: 0 2 2.

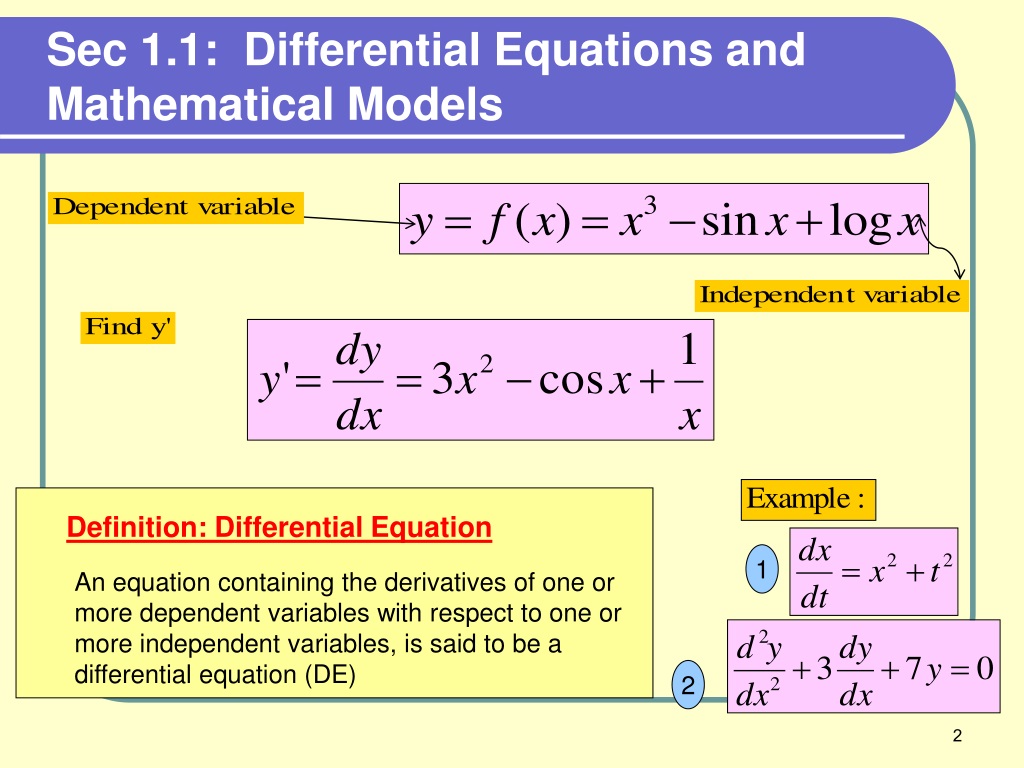
Ppt Chapter 1 First Order Differential Equations Powerpoint 1.4 linear equation: 2 1.5 homogeneous linear equation: 3 1.6 partial differential equation (pde) 3 1.7 general solution of a linear differential equation 3 1.8 a system of ode’s 4 2 the approaches of finding solutions of ode 5 2.1 analytical approaches 5 2.2 numerical approaches 5 2. first order differential equations 7 1 linear equation 7. Typically, the first differential equations encountered are first order equa first order differential equation tions. a first order differential equation takes the form f(y0,y, x) = 0.(1.14) there are two common first order differential equations for which one can formally obtain a solution. the first is the separable case and the second is. De class no tes 1 a collection of han douts on first order ordinary differential equations (ode's) chapter 1 introduction to differential equations and mathematical modeling, and a technique for solving first order linear ode’s 1. introduction to differential equations and mathematical modeling 2. useful characteristics of ode's 3. We consider the general first order differential equation: τdy(t) dt y(t) = x(t) the general solution is given by: y(t) = y0e − (t − t0) τ e − (t − t0) τ τ ∫t t0x(t′)e(t − t0) τdt′. where y0 = y(t − t0). note that t′ is used to be distinguished from the upper limit t of the integral. to obtain the general.

Solution Chapter 1first Order Differential Equations With Examples By De class no tes 1 a collection of han douts on first order ordinary differential equations (ode's) chapter 1 introduction to differential equations and mathematical modeling, and a technique for solving first order linear ode’s 1. introduction to differential equations and mathematical modeling 2. useful characteristics of ode's 3. We consider the general first order differential equation: τdy(t) dt y(t) = x(t) the general solution is given by: y(t) = y0e − (t − t0) τ e − (t − t0) τ τ ∫t t0x(t′)e(t − t0) τdt′. where y0 = y(t − t0). note that t′ is used to be distinguished from the upper limit t of the integral. to obtain the general. One of our main goals in this chapter is to find solutions to first order differential equa tions of the form (1.12.1). there are various ways in which we can seek these solutions: 1. geometrically: the function f(x,y)gives the slope of the tangent line to the solution curves of the differential equation (1.12.1) at the point (x,y). thus, by. Chapter 1 first‐order differential equations 6 solutions a function that satisfies the de is a solution to that de. seeking such functions is the main objective of the book while composing the des, which may excite engineering majors more, is the secondary objective of this book.

Solution Differential Equations For Engineering Chapter 1 First Order One of our main goals in this chapter is to find solutions to first order differential equa tions of the form (1.12.1). there are various ways in which we can seek these solutions: 1. geometrically: the function f(x,y)gives the slope of the tangent line to the solution curves of the differential equation (1.12.1) at the point (x,y). thus, by. Chapter 1 first‐order differential equations 6 solutions a function that satisfies the de is a solution to that de. seeking such functions is the main objective of the book while composing the des, which may excite engineering majors more, is the secondary objective of this book.