Slope Formula What Is Slope Formula Equation Examples
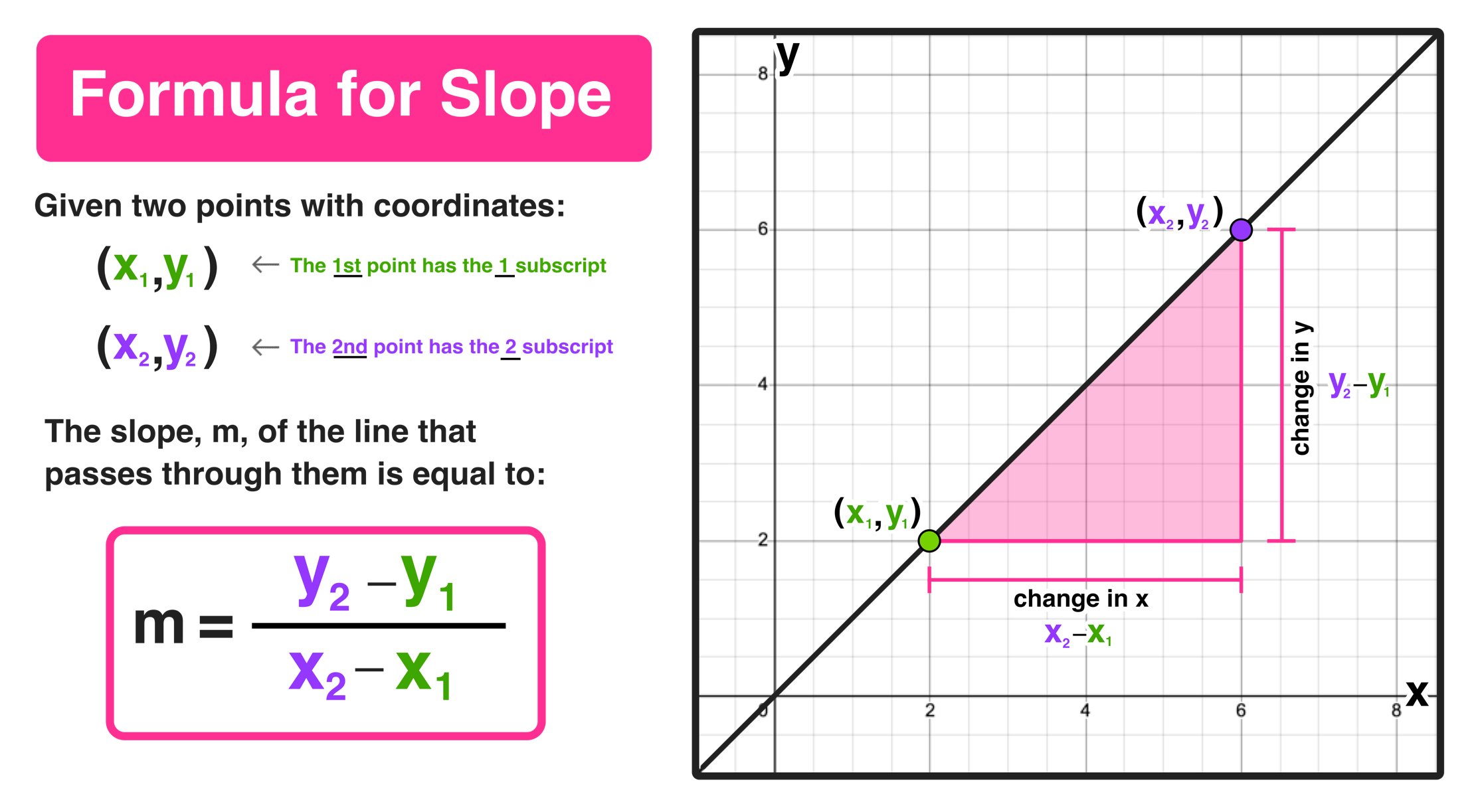
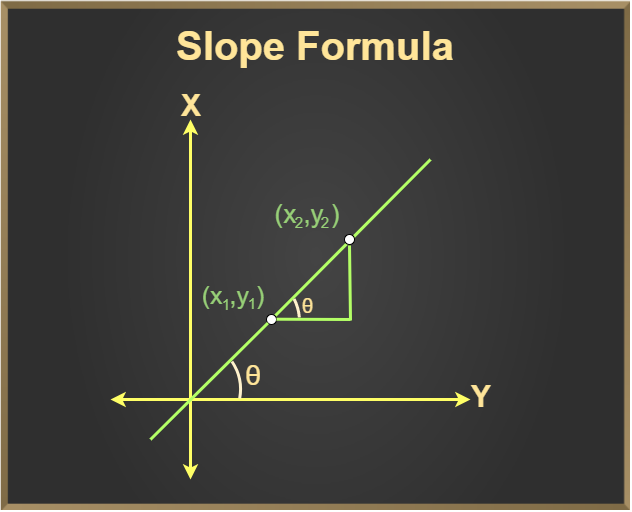
Formula For Slope Overview Equation Examples Mashup Math Thus, the slope equation is, m = tanθ = Δy Δx. from the graph, we observe: Δy = (y 2 y 1) Δx = (x 2 x 1) thus, the slope formula is given as: slope = m = (y 2 y 1) (x 2 x 1) slope equation. as we discussed in the previous section, the slope formula can be used to determine the slope of any line. In this first example, you have to find the slope of the line that passes through the points (2,4) and (4,3). to find the slope, simply identify the values of x1, x2, y1, and y2 and the plug these values into the formula for slope and solve (be sure to express your answer in reduced form if possible).
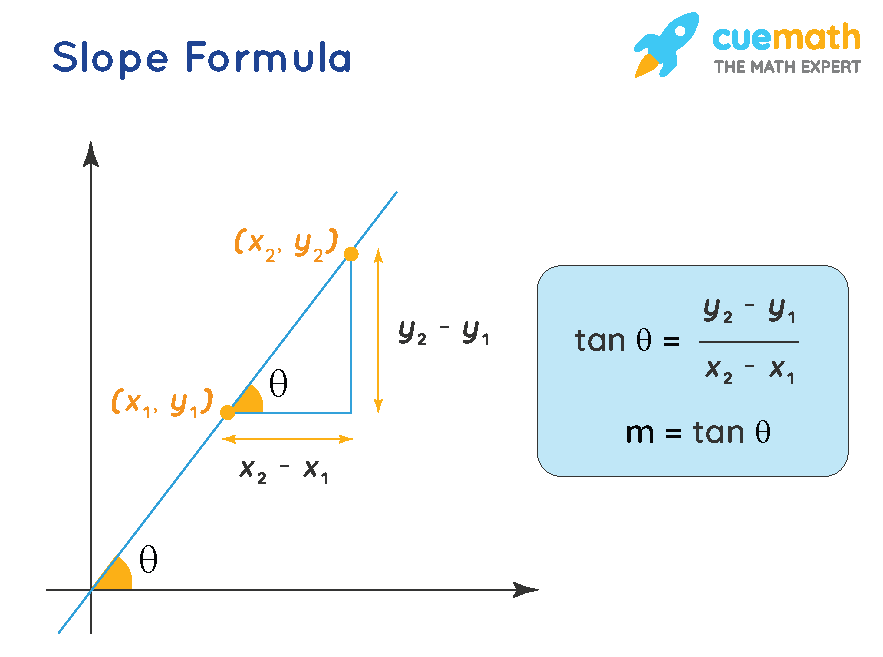
Slope Formula What Is Slope Formula Equation Examples Slope of a is the change in y coordinate with respect to the change in x coordinate. visit byju's to learn the slope formulas, equations and many solved examples in detail. Examples of how to find the slope of a line using the slope formula. example 1: determine the slope of the line passing through the points [latex]\left( {3,5} \right)[ latex] and [latex]\left( {7,13} \right)[ latex]. start by assigning which ones will be our first and second points. Example. given two points, (1, 3) and (4, 7), we can plug them into the formula to find the slope: the slope is which means that for every increase of 4 units in y, there is an increase of 3 units in x. change in y is sometimes referred to as "rise" while change in x is referred to as "run.". In the example to the right, we are asked to determine the slope of the line that passes through the ordered pairs ( 3,8) and (2, 11). we first identify the components of both ordered pairs by noticing which numbers are the x values and which are the y values. then we substitute these numbers into the slope formula and calculate the slope.
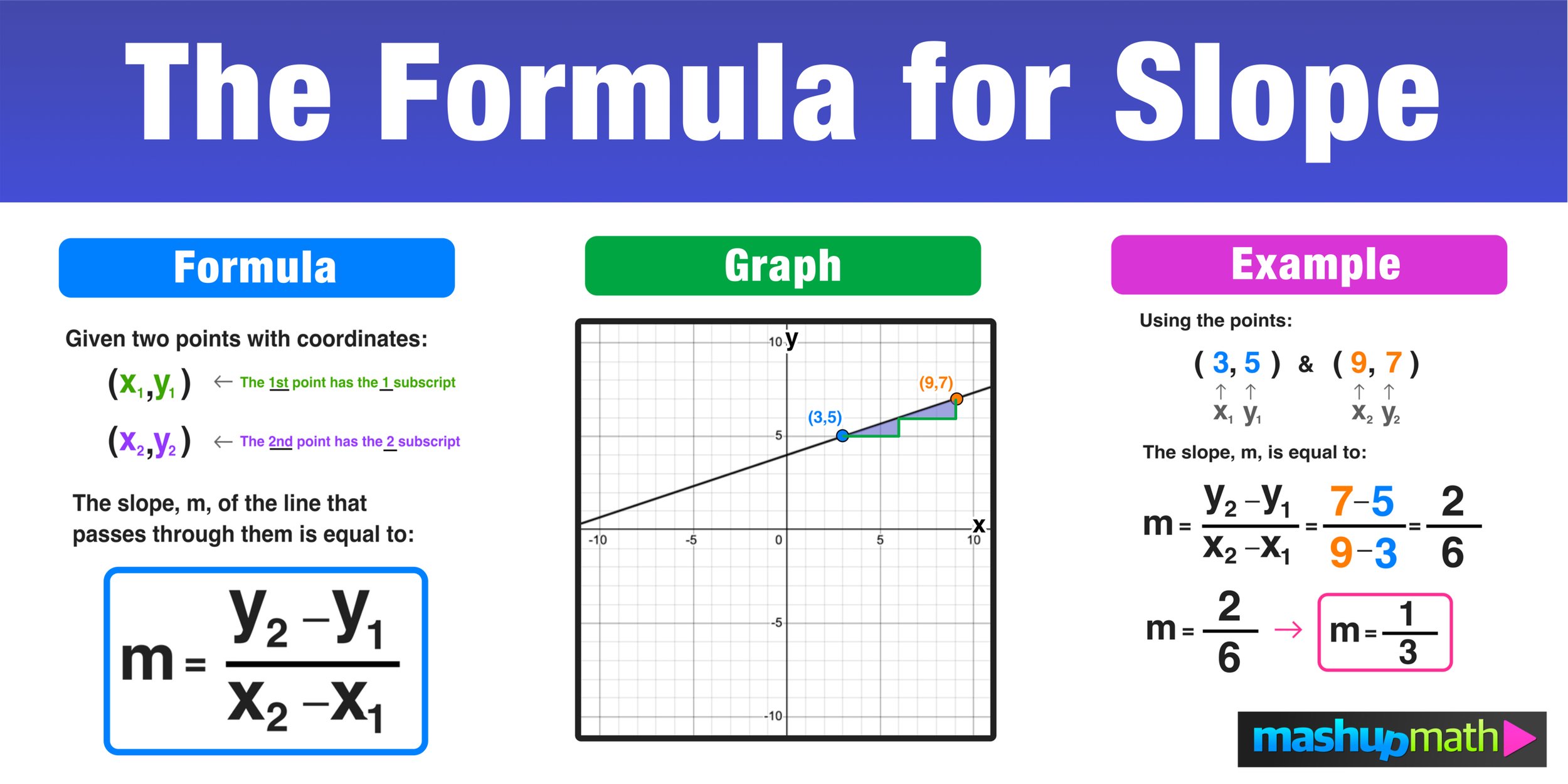
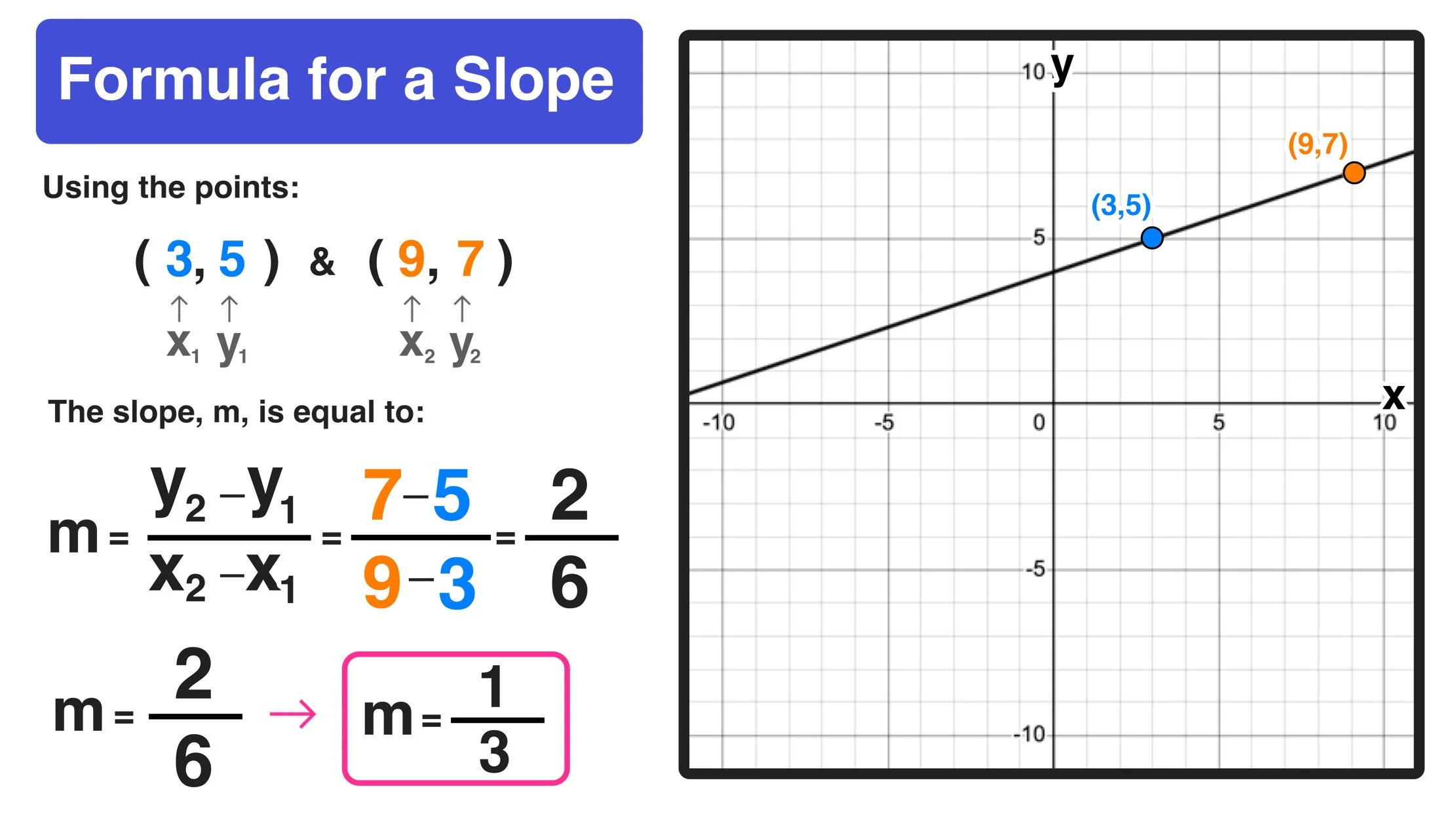
Formula For Slope Overview Equation Examples Mashup Math Example. given two points, (1, 3) and (4, 7), we can plug them into the formula to find the slope: the slope is which means that for every increase of 4 units in y, there is an increase of 3 units in x. change in y is sometimes referred to as "rise" while change in x is referred to as "run.". In the example to the right, we are asked to determine the slope of the line that passes through the ordered pairs ( 3,8) and (2, 11). we first identify the components of both ordered pairs by noticing which numbers are the x values and which are the y values. then we substitute these numbers into the slope formula and calculate the slope. Example one. the slope of a line going through the point (1, 2) and the point (4, 3) is $$ \frac{1}{3}$$ remember: difference in the y values goes in the numerator of formula, and the difference in the x values goes in denominator of the formula. Example 1: given a line with the equation, 2y = 8x 9, find its slope. solution: we know that the general formula of the slope is given as, y = mx b. hence, we try to bring the equation to this form. we make the coefficient of y = 1, and hence we get, y = 4x 4.5. clearly, the coefficient of x is found to be 4.

Slope Formula Explained W 15 Step By Step Examples Example one. the slope of a line going through the point (1, 2) and the point (4, 3) is $$ \frac{1}{3}$$ remember: difference in the y values goes in the numerator of formula, and the difference in the x values goes in denominator of the formula. Example 1: given a line with the equation, 2y = 8x 9, find its slope. solution: we know that the general formula of the slope is given as, y = mx b. hence, we try to bring the equation to this form. we make the coefficient of y = 1, and hence we get, y = 4x 4.5. clearly, the coefficient of x is found to be 4.
Formula For Slope Overview Equation Examples Mashup Math
Slope Formula What Is Slope Formula Equation Examples Faqs

Comments are closed.