Section 5 Factoring Higher Degree Polynomials

Section 5 Factoring Higher Degree Polynomials Youtube The solutions are x=0, x= 4, and x= 2. identify polynomials that act like a quadratic. you likely already know how to solve second degree polynomials, in the form . you can solve some higher degree polynomials the same way, if they're in the form . here are a couple examples: x = ±√ (2 3). Section 1.5 : factoring polynomials. of all the topics covered in this chapter factoring polynomials is probably the most important topic. there are many sections in later chapters where the first step will be to factor a polynomial. so, if you can’t factor the polynomial then you won’t be able to even start the problem let alone finish it.
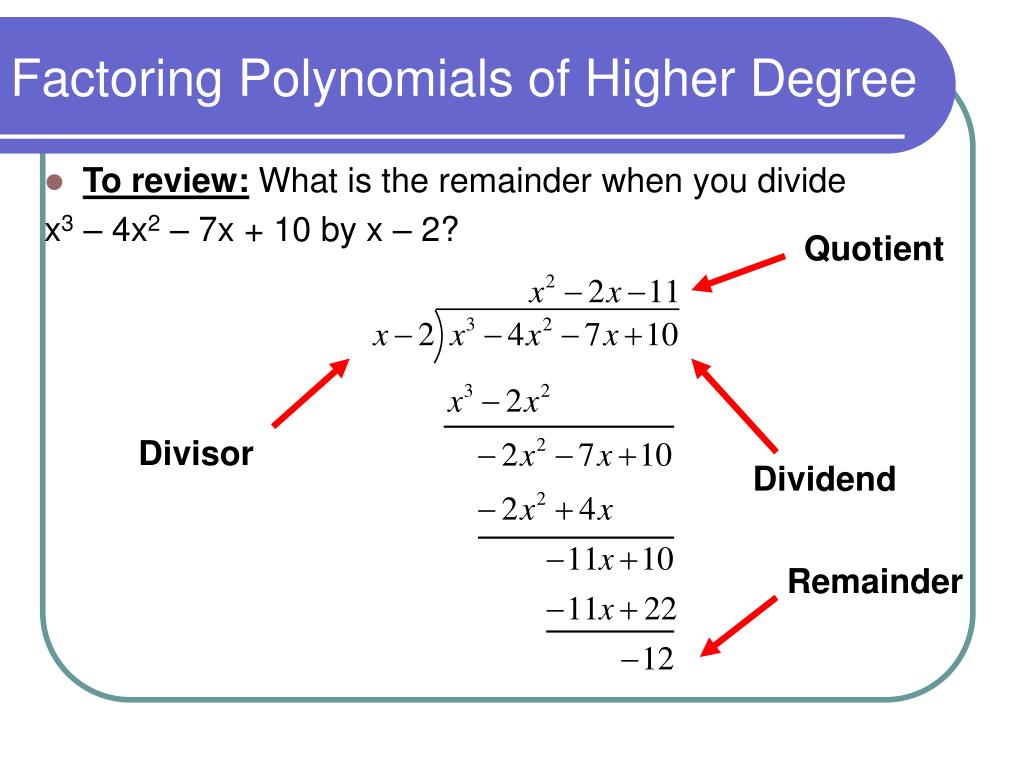
Ppt Factoring Polynomials Of Higher Degree Powerpoint Presentation Section 1.5 : factoring polynomials. for problems 1 – 4 factor out the greatest common factor from each polynomial. 6x7 3x4 −9x3 6 x 7 3 x 4 − 9 x 3 solution. a3b8 −7a10b4 2a5b2 a 3 b 8 − 7 a 10 b 4 2 a 5 b 2 solution. 2x(x2 1)3 −16(x2 1)5 2 x (x 2 1) 3 − 16 (x 2 1) 5 solution. x2(2−6x) 4x(4−12x) x 2 (2 − 6 x) 4. Factor polynomials by taking common factors, grouping, and using known quadratic methods. Higher degree equations name directions: solve each polynomial equation for all values ofx. show all work, your answers can be found in the "answer chart" beware as there are "extra" answers. when finished, create equations for the four un used "extra" answers, o. Example 1. an example of a polynomial (with degree 3) is: p(x) = 4x 3 − 3x 2 − 25x − 6. the factors of this polynomial are: (x − 3), (4x 1), and (x 2) note there are 3 factors for a degree 3 polynomial. when we multiply those 3 terms in brackets, we'll end up with the polynomial p(x).
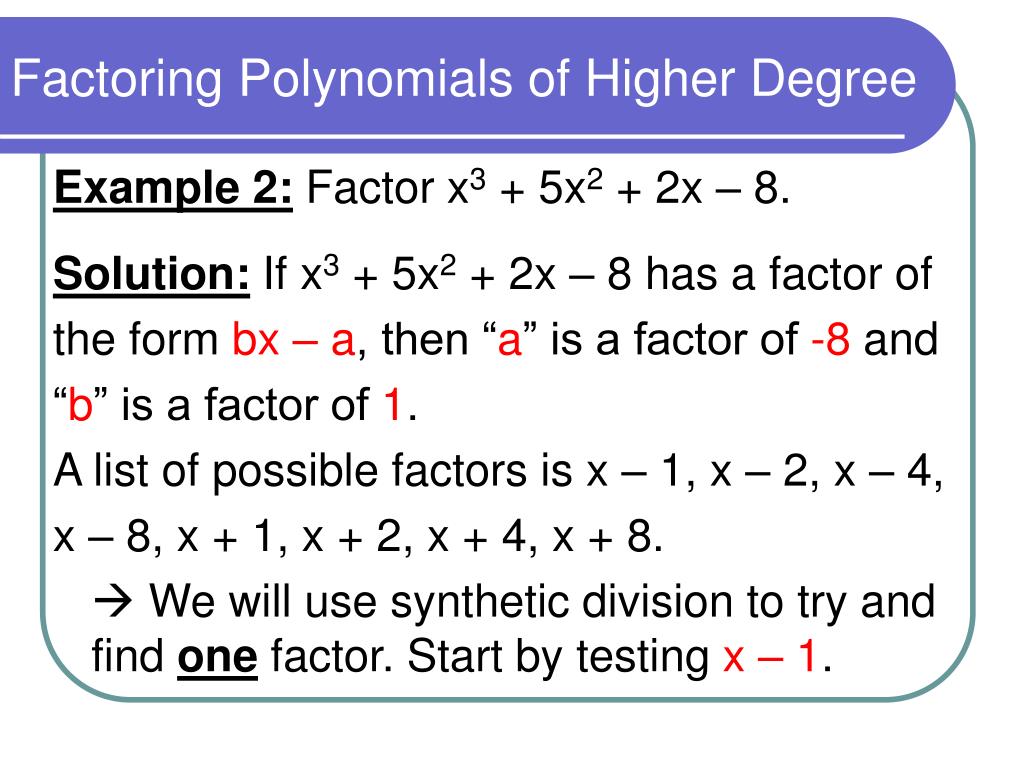
Ppt Factoring Polynomials Of Higher Degree Powerpoint Presentation Higher degree equations name directions: solve each polynomial equation for all values ofx. show all work, your answers can be found in the "answer chart" beware as there are "extra" answers. when finished, create equations for the four un used "extra" answers, o. Example 1. an example of a polynomial (with degree 3) is: p(x) = 4x 3 − 3x 2 − 25x − 6. the factors of this polynomial are: (x − 3), (4x 1), and (x 2) note there are 3 factors for a degree 3 polynomial. when we multiply those 3 terms in brackets, we'll end up with the polynomial p(x). To factor a higher degree polynomial, remove factors using synthetic or long division until you have a quadratic which can be factored or there are no more factors that can be taken out. i f p x − q is a factor of a polynomial with integer coefficient, p is a factor of the leading coefficient, and q is a factor of the constant. Course: algebra (all content) > unit 10. lesson 27: advanced polynomial factorization methods. factoring higher degree polynomials. factoring sum of cubes. factoring difference of cubes.

Unit 5 Factoring Higher Degree Polynomials Youtube To factor a higher degree polynomial, remove factors using synthetic or long division until you have a quadratic which can be factored or there are no more factors that can be taken out. i f p x − q is a factor of a polynomial with integer coefficient, p is a factor of the leading coefficient, and q is a factor of the constant. Course: algebra (all content) > unit 10. lesson 27: advanced polynomial factorization methods. factoring higher degree polynomials. factoring sum of cubes. factoring difference of cubes.

Comments are closed.