Relations Discrete Mathematics
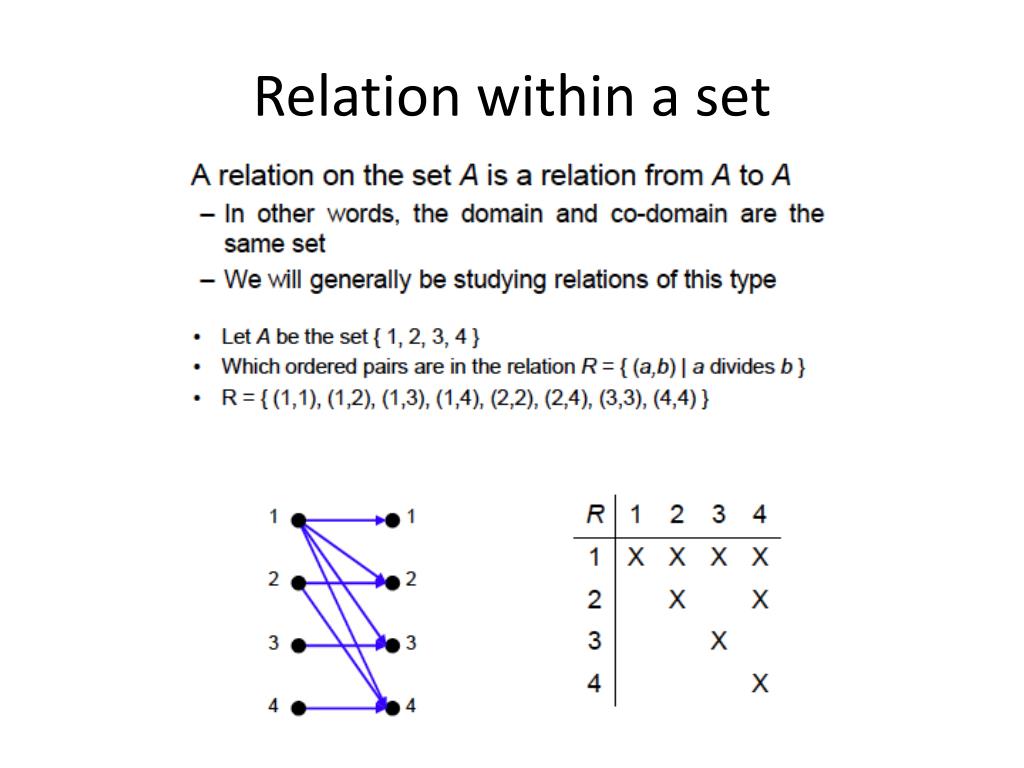
Ppt 22c 19 Discrete Math Relations Powerpoint Presentation Free Summary and review. relations are generalizations of functions. a relation merely states that the elements from two sets a and b are related in a certain way. more formally, a relation is defined as a subset of a × b. the domain is the set of elements in a and the codomain is the set of elements in b. the range of a relation is the set of. Relations are used in mathematics, statistics, and data sciences. it is an integral part of rdbms (relational database management systems) used to search, store, and sort large data. in this article, we will learn about, relation in mathematics, example of relation, types of relation, and others in detail.
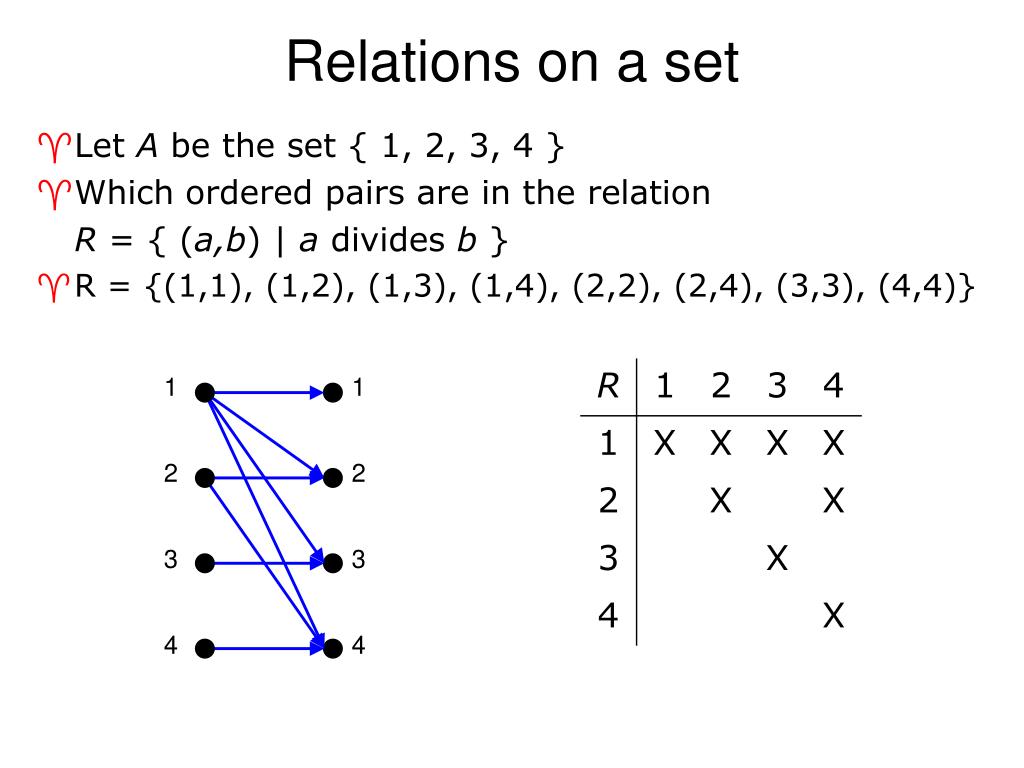
Ppt Discrete Mathematics Relations Powerpoint Presentation Free More formally, a relation is defined as a subset of. a × b. . the domain of a relation is the set of elements in. a. that appear in the first coordinates of some ordered pairs, and the image or range is the set of elements in. b. that appear in the second coordinates of some ordered pairs. for brevity and for clarity, we often write. Looking for paid tutoring or online courses with practice exercises, text lectures, solutions, and exam practice? trevtutor has you covered!we int. A relation from a set \(a\) to itself is called a relation on set \(a\). given any relation \(r\) on a set \(a\), we are interested in three properties that \(r\) may or may not have. the relation \(r\) is said to be reflexive if every element is related to itself, that is, if \(x\,r\,x\) for every \(x\in a\). Discrete mathematics relations whenever sets are being discussed, the relationship between the elements of the sets is the next thing that comes up. relations may exist between objects of the same set or between objects of two or more sets.

Discrete Mathematics Introduction To Relations Youtube A relation from a set \(a\) to itself is called a relation on set \(a\). given any relation \(r\) on a set \(a\), we are interested in three properties that \(r\) may or may not have. the relation \(r\) is said to be reflexive if every element is related to itself, that is, if \(x\,r\,x\) for every \(x\in a\). Discrete mathematics relations whenever sets are being discussed, the relationship between the elements of the sets is the next thing that comes up. relations may exist between objects of the same set or between objects of two or more sets. Definition if a and b are sets, then a binary relation from a to b is a subset of a × b. we say that x is related to y by r, written x r y, if, and only if, (x, y) ∈ r. denoted as x r y ⇔ (x, y) ∈ r . relationship. set of all functions is a proper subset of the set of all relations. Introduction to video: relations discrete math 00:00:34. relation properties: reflexive, irreflexive, symmetric, antisymmetric, and transitive 00:18:55 decide which of the five properties is illustrated for relations in roster form (examples #1 5) 00:42:10 which of the five properties is specified for: x and y are born on the same day (example #6a).

Discrete Math Relations Illustrated W 15 Examples Definition if a and b are sets, then a binary relation from a to b is a subset of a × b. we say that x is related to y by r, written x r y, if, and only if, (x, y) ∈ r. denoted as x r y ⇔ (x, y) ∈ r . relationship. set of all functions is a proper subset of the set of all relations. Introduction to video: relations discrete math 00:00:34. relation properties: reflexive, irreflexive, symmetric, antisymmetric, and transitive 00:18:55 decide which of the five properties is illustrated for relations in roster form (examples #1 5) 00:42:10 which of the five properties is specified for: x and y are born on the same day (example #6a).

Discrete Math Intro To Relations Youtube

Comments are closed.