Pythagoras Theorem Formula Proof Examples

How To Prove The Pythagorean Theorem 10 Steps With Pictures Pythagoras theorem (also called pythagorean theorem) is an important topic in mathematics, which explains the relation between the sides of a right angled triangle. the sides of the right triangle are also called pythagorean triples. the formula and proof of this theorem are explained here with examples. Example 1: the hypotenuse of a right angled triangle is 16 units and one of the sides of the triangle is 8 units. find the measure of the third side using the pythagoras theorem formula. solution: given: hypotenuse = 16 units. let us consider the given side of a triangle as the perpendicular height = 8 units.

Solution The Pythagoras Theorem Pythagorean Theorem Formula Proof The sum of the smaller squares (orange and yellow) is equal to the largest square (blue). the pythagorean theorem relates the three sides in a right triangle. to be specific, relating the two legs and the hypotenuse, the longest side. the pythagorean theorem can be summarized in a short and compact equation as shown below. Proof by rearrangement. pythagoras's proof. given any right triangle with legs a a and b b and hypotenuse c c like the above, use four of them to make a square with sides a b a b as shown below: this forms a square in the center with side length c c and thus an area of c^2. c2. however, if we rearrange the four triangles as follows, we can see. What is the pythagorean theorem? you can learn all about the pythagorean theorem, but here is a quick summary: the pythagorean theorem says that, in a right triangle, the square of a (which is a×a, and is written a 2) plus the square of b (b 2) is equal to the square of c (c 2): a 2 b 2 = c 2. proof of the pythagorean theorem using algebra. Use the pythagorean theorem to determine the length of x. step 1. identify the legs and the hypotenuse of the right triangle. the legs have length 6 and 8. x x is the hypotenuse because it is opposite the right angle. step 2. substitute values into the formula (remember 'c' is the hypotenuse). a2 b2 = c2 62 82 = x2 a 2 b 2 = c 2 6 2 8 2.
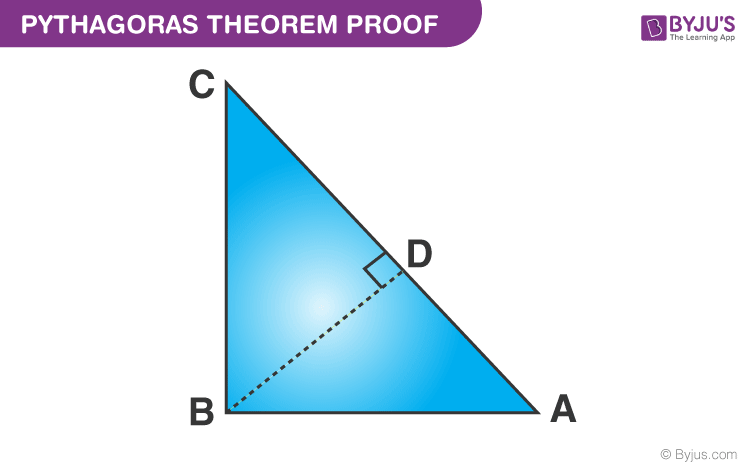
Pythagoras Theorem Formula Proof Examples Applications What is the pythagorean theorem? you can learn all about the pythagorean theorem, but here is a quick summary: the pythagorean theorem says that, in a right triangle, the square of a (which is a×a, and is written a 2) plus the square of b (b 2) is equal to the square of c (c 2): a 2 b 2 = c 2. proof of the pythagorean theorem using algebra. Use the pythagorean theorem to determine the length of x. step 1. identify the legs and the hypotenuse of the right triangle. the legs have length 6 and 8. x x is the hypotenuse because it is opposite the right angle. step 2. substitute values into the formula (remember 'c' is the hypotenuse). a2 b2 = c2 62 82 = x2 a 2 b 2 = c 2 6 2 8 2. It is called "pythagoras' theorem" and can be written in one short equation: a 2 b 2 = c 2. note: c is the longest side of the triangle; a and b are the other two sides; definition. the longest side of the triangle is called the "hypotenuse", so the formal definition is:. So, according to pythagoras theorem. in a right angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides. mathematically, base2 perpendicular2 = hypotenuse2. therefore, if “a” is the base, “b” is the perpendicular and “c” is the hypotenuse in a right angled triangle, then. c 2 = a 2.

Comments are closed.