Properties Of A Parallelogram Neurochispas

Properties Of A Parallelogram Neurochispas The fundamental properties of parallelograms are as follows: the opposite sides are parallel. the opposite sides are congruent (they have the same length). consecutive angles are supplementary. if one of the angles is a right angle, then the other angles will also be right angles. the two diagonals bisect each other. The perimeter of a parallelogram represents the length of the contour of the parallelogram. on the other hand, the area is a measure of the two dimensional space occupied by the figure. we can find the perimeter of the parallelogram by adding the lengths of its four sides, and we can find the area by multiplying the length of its base by its.
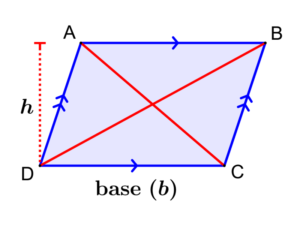
Properties Of A Parallelogram Neurochispas A parallelogram is a quadrilateral in which opposite sides are parallel and have the same length. having opposite sides that are parallel and of equal lengths, it makes the angles on the opposite sides equal as well. the diagonals of a parallelogram are the segments that connect the opposite corners of the figure. The opposite sides of a parallelogram are equal. the opposite angles of a parallelogram are equal. the diagonals of a parallelogram bisect each other. if one pair of opposite sides is equal and parallel in a quadrilateral then it is a parallelogram. theorem 1: in a parallelogram the opposite sides are equal. A parallelogram is a quadrilateral made from two pairs of intersecting parallel lines. there are several rules involving: the angles of a parallelogram. the sides of a parallelogram. the diagonals of a parallelogram. rule 1: opposite sides are parallel read more. rule 2: opposite sides are congruent read more. There are six important properties of parallelograms to know: opposite sides are congruent (ab = dc). opposite angels are congruent (d = b). consecutive angles are supplementary (a d = 180°). if one angle is right, then all angles are right. the diagonals of a parallelogram bisect each other. each diagonal of a parallelogram separates it.

Properties Of A Parallelogram Neurochispas A parallelogram is a quadrilateral made from two pairs of intersecting parallel lines. there are several rules involving: the angles of a parallelogram. the sides of a parallelogram. the diagonals of a parallelogram. rule 1: opposite sides are parallel read more. rule 2: opposite sides are congruent read more. There are six important properties of parallelograms to know: opposite sides are congruent (ab = dc). opposite angels are congruent (d = b). consecutive angles are supplementary (a d = 180°). if one angle is right, then all angles are right. the diagonals of a parallelogram bisect each other. each diagonal of a parallelogram separates it. Definition. a parallelogram is a quadrilateral that has opposite sides that are parallel. since a parallelogram is a quadrilateral, a parallelogram has all of the properties of a quadrilateral in addition to properties unique to itself. the sections below will address its unique properties. property: opposite sides. Formula of parallelogram perimeter in terms of sides: p = 2 a 2 b = 2 (a b) 2. formula of parallelogram perimeter in terms of one side and diagonals: p = 2 a √ 2d12 2d22 4a2. p = 2 b √ 2d12 2d22 4b2. 3. formula of parallelogram perimeter in terms of side, height and sine of an angle: p =.

Area And Perimeter Of A Parallelogram Formulas And Examples Definition. a parallelogram is a quadrilateral that has opposite sides that are parallel. since a parallelogram is a quadrilateral, a parallelogram has all of the properties of a quadrilateral in addition to properties unique to itself. the sections below will address its unique properties. property: opposite sides. Formula of parallelogram perimeter in terms of sides: p = 2 a 2 b = 2 (a b) 2. formula of parallelogram perimeter in terms of one side and diagonals: p = 2 a √ 2d12 2d22 4a2. p = 2 b √ 2d12 2d22 4b2. 3. formula of parallelogram perimeter in terms of side, height and sine of an angle: p =.
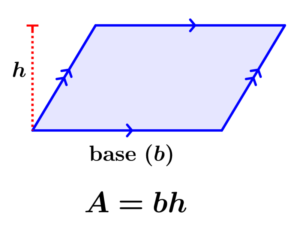
Area Of A Parallelogram Formulas And Examples Neurochispas

Diagonal Of A Parallelogram Formulas And Examples Neurochispas

Comments are closed.