Proof By Induction W 9 Step By Step Examples

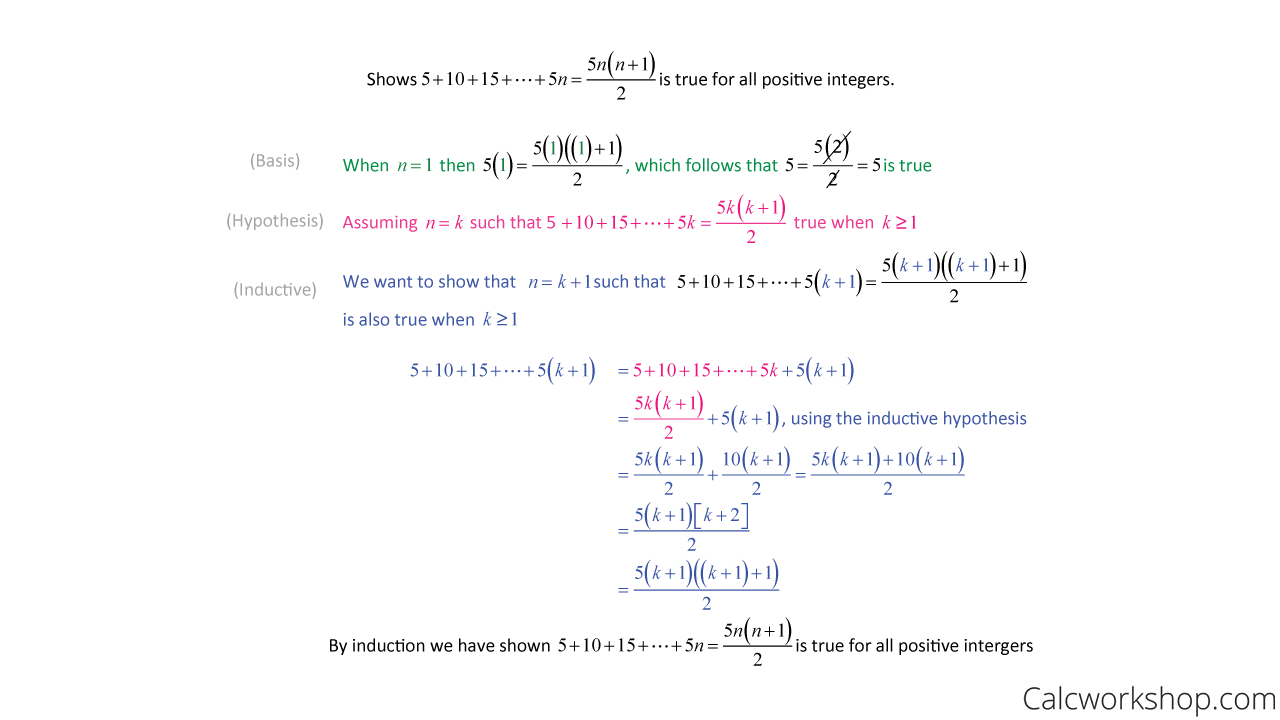
Proof By Induction W 9 Step By Step Examples Steps for proof by induction: the basis step. the hypothesis step. and the inductive step. where our basis step is to validate our statement by proving it is true when n equals 1. then we assume the statement is correct for n = k, and we want to show that it is also proper for when n = k 1. the idea behind inductive proofs is this: imagine. Process of proof by induction. there are two types of induction: regular and strong. the steps start the same but vary at the end. here are the steps. in mathematics, we start with a statement of our assumptions and intent: let p(n), ∀n ≥ n0, n, n0 ∈ z be a statement. we would show that p (n) is true for all possible values of n.

Proof By Induction W 9 Step By Step Examples Mathematical induction (or weak mathematical induction) is a method to prove or establish mathematical statements, propositions, theorems, or formulas for all natural numbers ‘n ≥1.’ principle. it involves two steps: base step: it proves whether a statement is true for the initial value (n), usually the smallest natural number in. Direct proof, so we assume p(n) is true, and derive p(n 1). this is called the \inductive step." the base case and inductive step are often labeled as such in a proof. the assumption that p(n) is true, made in the inductive step, is often referred to as the inductive hypothesis. let’s look at a few examples of proof by induction. Proof by induction: strong form. example 1. example 2. one of the most powerful methods of proof — and one of the most difficult to wrap your head around — is called mathematical induction, or just “induction" for short. i like to call it “proof by recursion," because this is exactly what it is. The principle of induction thus implies that n3 −n is indeed divisible by 3 for all n ≥ 2. 2) show by induction that n < 2n for all natural numbers n. step a) (check): for n = 1, since 21 = 2, it is true that 1 < 21. step b) (induction step): assume it is true for n = k, i.e., k < 2k.

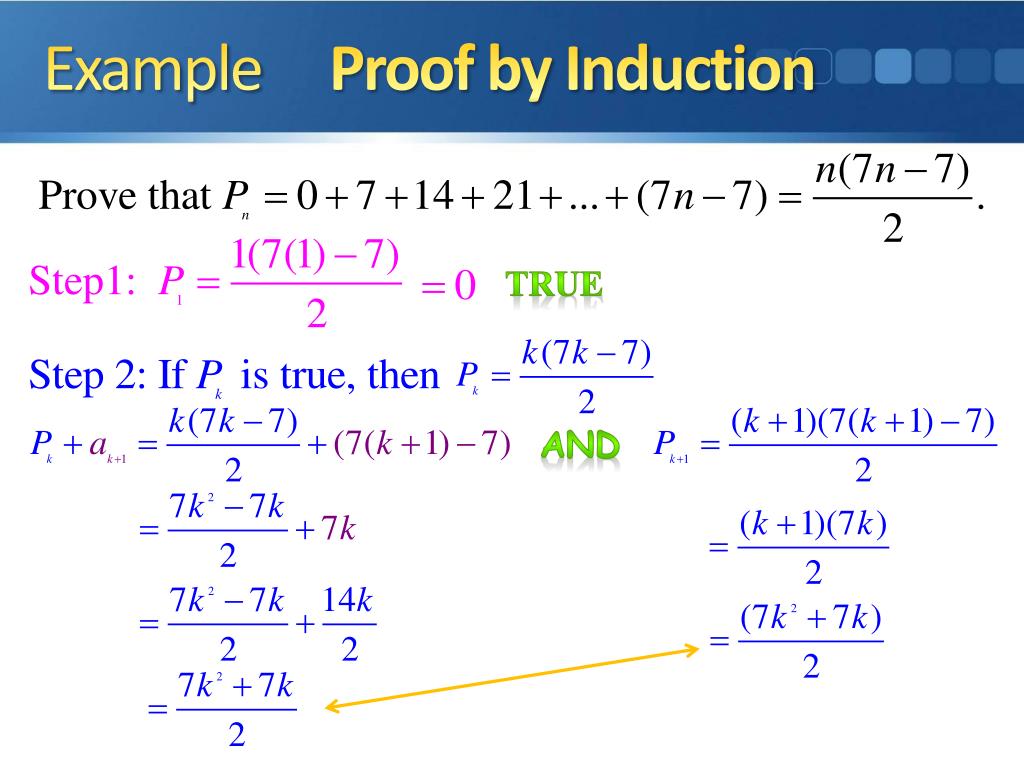
Ppt Mathematical Induction Powerpoint Presentation Free Download Proof by induction: strong form. example 1. example 2. one of the most powerful methods of proof — and one of the most difficult to wrap your head around — is called mathematical induction, or just “induction" for short. i like to call it “proof by recursion," because this is exactly what it is. The principle of induction thus implies that n3 −n is indeed divisible by 3 for all n ≥ 2. 2) show by induction that n < 2n for all natural numbers n. step a) (check): for n = 1, since 21 = 2, it is true that 1 < 21. step b) (induction step): assume it is true for n = k, i.e., k < 2k. Proof by induction examples. if you think you have the hang of it, here are two other mathematical induction problems to try: 1) the sum of the first n positive integers is equal to \frac {n (n 1)} {2} 2n(n 1) we are not going to give you every step, but here are some head starts: base case: p (1) = 1 (1 1) 2. Prove the inductive step, p (k) → p (k 1), by assuming that p (k) is true, called the inductive hypothesis, then prove that p (k 1) is also true. use the principle of mathematical induction to prove that p (n) is true for all integers n ≥ a. in many examples a = 1 or a = 0, but it is possible to start induction using any integer base a.

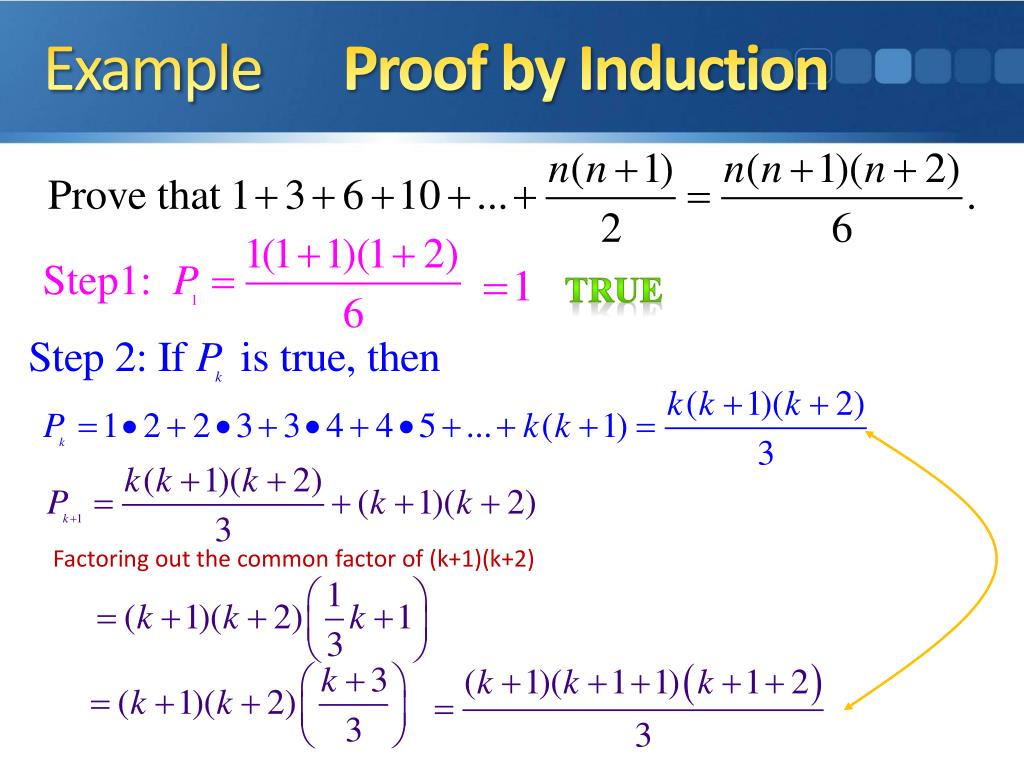
Ppt Mathematical Induction Powerpoint Presentation Free Download Proof by induction examples. if you think you have the hang of it, here are two other mathematical induction problems to try: 1) the sum of the first n positive integers is equal to \frac {n (n 1)} {2} 2n(n 1) we are not going to give you every step, but here are some head starts: base case: p (1) = 1 (1 1) 2. Prove the inductive step, p (k) → p (k 1), by assuming that p (k) is true, called the inductive hypothesis, then prove that p (k 1) is also true. use the principle of mathematical induction to prove that p (n) is true for all integers n ≥ a. in many examples a = 1 or a = 0, but it is possible to start induction using any integer base a.

Proof By Induction Definition Steps Examples Study

Proof By Induction Example 1 Youtube