Pdf 5 1 Elementary Theory Of Initial Value Problems Pdf Wissam M

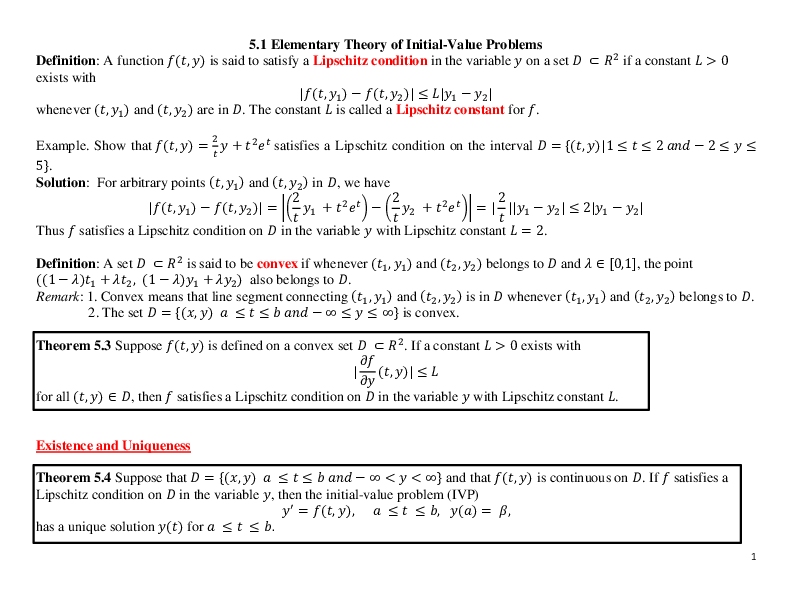
Pdf 5 1 Elementary Theory Of Initial Value Problems Pdf Wissam M 5.1 elementary theory of initial value problems is said to satisfy a lipschitz condition in the variable on a set definition: a function exists with are in . the constant and whenever example. show that . solution: for arbitrary points thus is called a lipschitz constant for . Definition 5.1. a function %(#, ") is said to satisfy a lipschitz condition. in the variable " on a set 0 ⊂ 23 if a constant 4 > 0 exists with |% #, "8 − % #, "3 | ≤ 4|"8 − "3| whenever #, "8 and #, "3 are in 0. the constant 4 is called a. lipschitz constant for %. definition 5.2 a set 0 ⊂ 23 is said to be convex if whenever and #3.

Pdf A New Approach For Solving Boundary And Initial Value Problems By 1. 5.1 elementary theory of initial value problems. 2. definition 5.1a function 𝑓𝑓(𝑡𝑡,𝑦𝑦) is said to satisfy a lipschitz condition. in the variable 𝑦𝑦 on a set 𝐷𝐷 ⊂𝑅𝑅2if a constant 𝐿𝐿> 0 exists with |𝑓𝑓(𝑡𝑡,𝑦𝑦1) −𝑓𝑓(𝑡𝑡,𝑦𝑦2)| ≤𝐿𝐿|𝑦𝑦1−𝑦𝑦2. 1 5.1 elementary theory of initial value problems definition: a function is said to satisfy a lipschitz condition in the variable on a set if a constant exists with whenever and . the constant are in is called a lipschitz constant for . example. show that. Chapter 5. initial value problems for odes 5.1 elementary theory of initial value problems 5.1 elementary theory of initial value problems in this chapter, we study numerical approximation of the initial value problem (ivp) of ordinary differential equations. we start from the ivp of a first order ode 8 <: dy dt = f(t;y); a t b y(a) = (5.1). Papers by wissam m tahir. 5.1 elementary theory of initial value problems.pdf. 5.1 elementary theory of initial value problems.pdf.

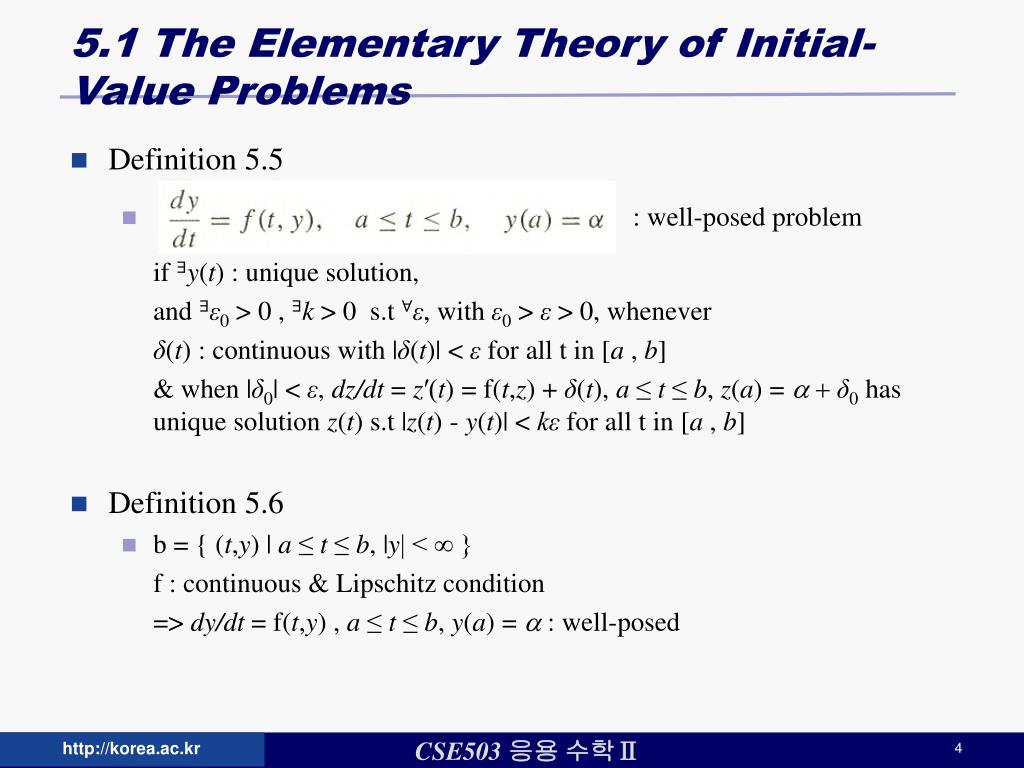
Ppt Chapter 5 Ordinary Differential Equation Powerpoint Presentation Chapter 5. initial value problems for odes 5.1 elementary theory of initial value problems 5.1 elementary theory of initial value problems in this chapter, we study numerical approximation of the initial value problem (ivp) of ordinary differential equations. we start from the ivp of a first order ode 8 <: dy dt = f(t;y); a t b y(a) = (5.1). Papers by wissam m tahir. 5.1 elementary theory of initial value problems.pdf. 5.1 elementary theory of initial value problems.pdf. We study numerical solution for initial value problem (ivp) of ordinary differential equations (ode). i a basic ivp: dy dt = f(t;y); for a t b with initial value y(a) = . remark i f is given and called the defining function of ivp. i is given and called the initial value. i y(t) is called the solution of the ivp if i y(a) = ;. Mat360 section summary: 5.1 elementary theory of initial value problems 1. summary first of all we need to say a little about what differential equations and initial value problems are, and the conditions under which they have unique solutions. then we need to realize that, because we’re solving these problems numerically, we’re not.

Pdf Semiclassical Theories As Initial Value Problems We study numerical solution for initial value problem (ivp) of ordinary differential equations (ode). i a basic ivp: dy dt = f(t;y); for a t b with initial value y(a) = . remark i f is given and called the defining function of ivp. i is given and called the initial value. i y(t) is called the solution of the ivp if i y(a) = ;. Mat360 section summary: 5.1 elementary theory of initial value problems 1. summary first of all we need to say a little about what differential equations and initial value problems are, and the conditions under which they have unique solutions. then we need to realize that, because we’re solving these problems numerically, we’re not.