Ordinary Differential Equation Formula Definition Examples

Ordinary Differential Equation Formula Definition Examples Answer: the order is 2. example 2: the rate of decay of the mass of a radio wave substance any time is k times its mass at that time, form the required ordinary differential equation satisfied by the mass of the substance. solution: the rate of decay of mass is dm dt. here dm dt is directly proportional to m. An n th order ordinary differential equations is linear if it can be written in the form; a 0 (x)y n a 1 (x)y n 1 … a n (x)y = r (x) the function a j (x), 0 ≤ j ≤ n are called the coefficients of the linear equation. the equation is said to be homogeneous if r (x) = 0. if r (x)≠0, it is said to be a non homogeneous equation.
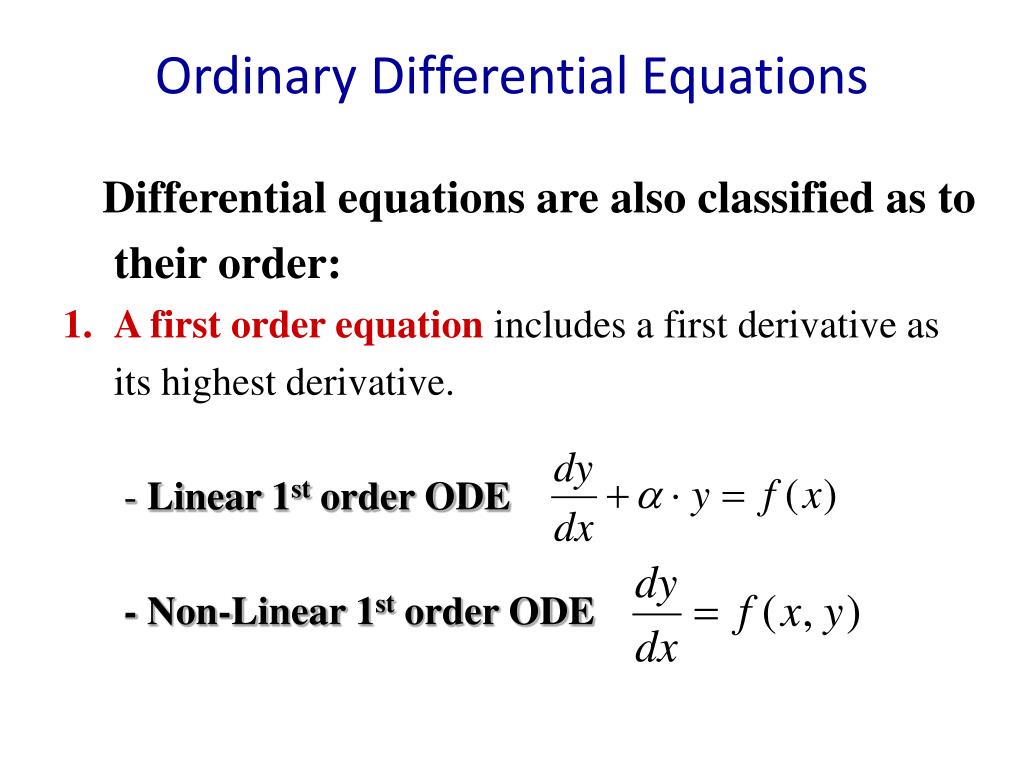
Ppt Part 7 Ordinary Differential Equations Odes Powerpoint An ordinary differential equation is a mathematical equation that involves the derivatives of an unknown function with respect to a single independent variable. it describes the relationship between function and its derivatives, commonly used to model various dynamic systems in physics, engineering, and other scientific fields. T. e. in mathematics, an ordinary differential equation (ode) is a differential equation (de) dependent on only a single independent variable. as with other de, its unknown (s) consists of one (or more) function (s) and involves the derivatives of those functions. [1]. Let's make things a little more complicated. consider the equation. dx dt = m sin t nt3, (2) (2) d x d t = m sin t n t 3, where m m and n n are just some real numbers. equation (2) (2) isn't much more complicated than equation (1) (1) because the right hand side does not depend on x x. it only depends on t t. Definition 1: ordinary differential equations. an ordinary differential equation (ode) is an equation for a function of one variable that involves (‘’ordinary”) derivatives of the function (and, possibly, known functions of the same variable). we give several examples below. d2x dt2 ω2x = 0 d 2 x d t 2 ω 2 x = 0.

Ordinary Differential Equations Intro Youtube Let's make things a little more complicated. consider the equation. dx dt = m sin t nt3, (2) (2) d x d t = m sin t n t 3, where m m and n n are just some real numbers. equation (2) (2) isn't much more complicated than equation (1) (1) because the right hand side does not depend on x x. it only depends on t t. Definition 1: ordinary differential equations. an ordinary differential equation (ode) is an equation for a function of one variable that involves (‘’ordinary”) derivatives of the function (and, possibly, known functions of the same variable). we give several examples below. d2x dt2 ω2x = 0 d 2 x d t 2 ω 2 x = 0. Ordinary differential equation. an ordinary differential equation (frequently called an "ode," "diff eq," or "diffy q") is an equality involving a function and its derivatives. an ode of order is an equation of the form. where is a function of , is the first derivative with respect to , and is the th derivative with respect to . Example 1. solve the ordinary differential equation (ode) dx dt = 5x − 3 d x d t = 5 x − 3. for x(t) x (t). solution: using the shortcut method outlined in the introduction to odes, we multiply through by dt d t and divide through by 5x − 3 5 x − 3: dx 5x − 3 = dt. d x 5 x − 3 = d t. we integrate both sides.
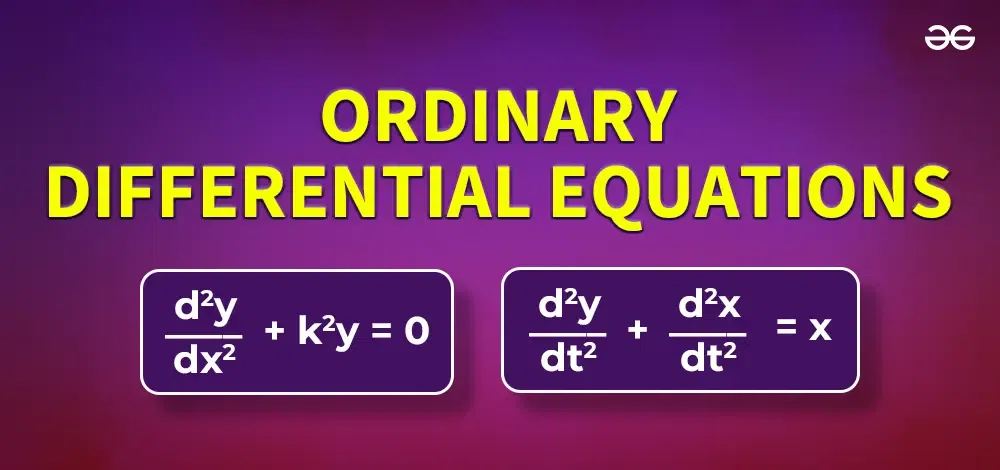
Ordinary Differential Equation Definition Types And Solutions Ordinary differential equation. an ordinary differential equation (frequently called an "ode," "diff eq," or "diffy q") is an equality involving a function and its derivatives. an ode of order is an equation of the form. where is a function of , is the first derivative with respect to , and is the th derivative with respect to . Example 1. solve the ordinary differential equation (ode) dx dt = 5x − 3 d x d t = 5 x − 3. for x(t) x (t). solution: using the shortcut method outlined in the introduction to odes, we multiply through by dt d t and divide through by 5x − 3 5 x − 3: dx 5x − 3 = dt. d x 5 x − 3 = d t. we integrate both sides.

Comments are closed.