Numerical Differentiation Examples
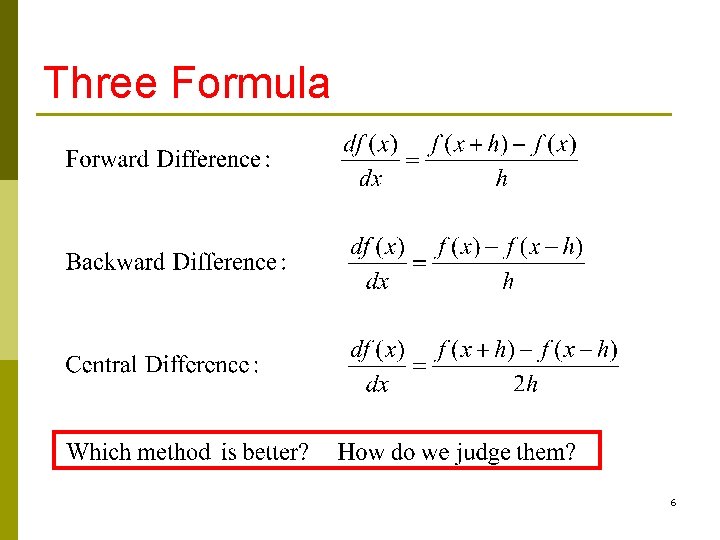
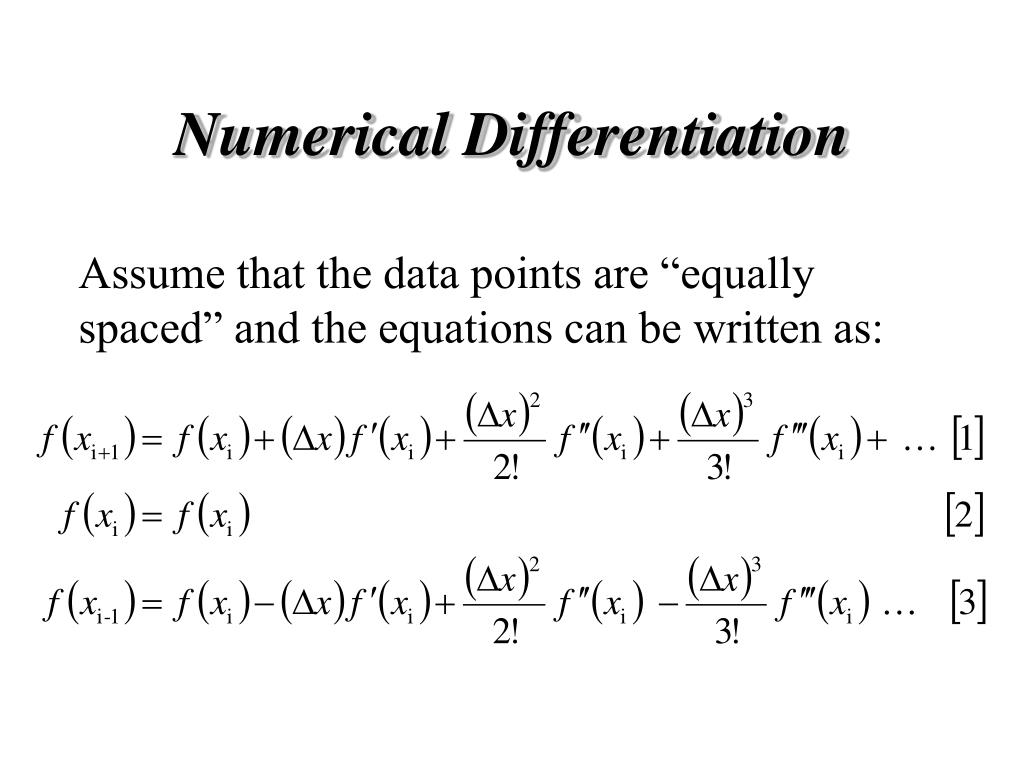
Numerical Differentiation 1 Numerical Differentiation First Order Example, a more accurate approximation for the first derivative that is based on the values of the function at the points f(x−h) and f(x h) is the centered differencing formula f0(x) ≈ f(x h)−f(x−h) 2h. (5.4) let’s verify that this is indeed a more accurate formula than (5.1). taylor expansions of the terms on the right hand side of. 178. chapter 9: numerical differentiation. numerical differentiation formulation of equations for physical problems often involve derivatives (rate of change quantities, such as v elocity and acceleration). numerical solution of such problems involves numerical evaluation of the derivatives. one method for numerically evaluating derivatives is.
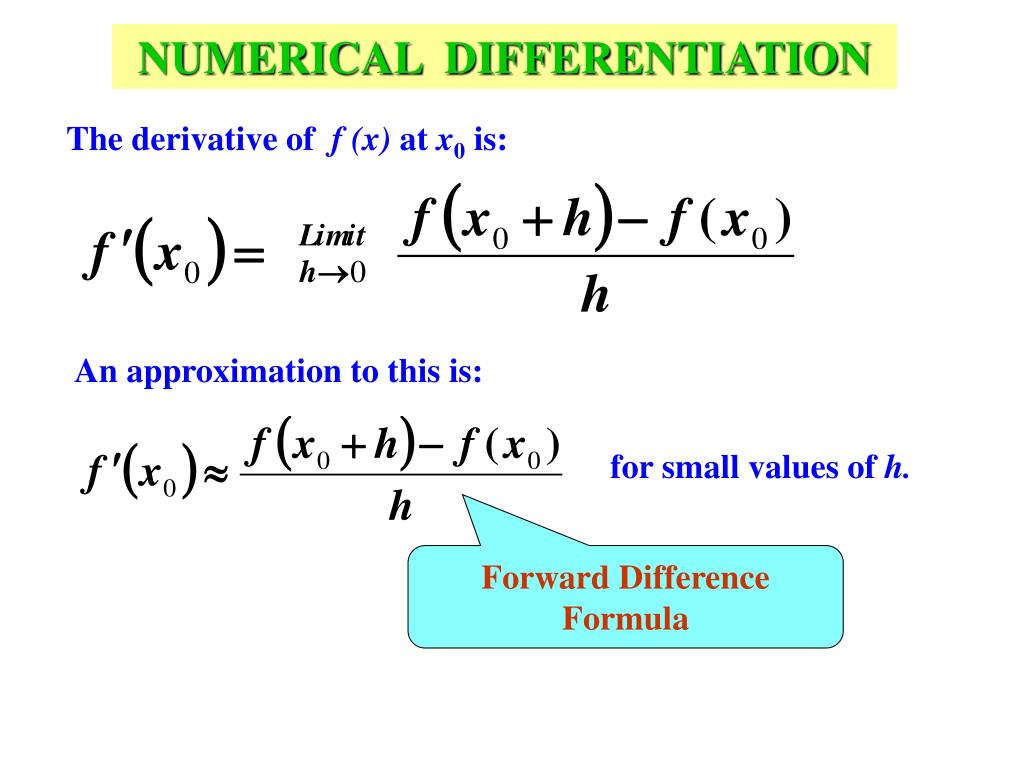
Ppt Lecture 18 Numerical Differentiation Powerpoint Presentation Numerical differentiation. use of numerical analysis to estimate derivatives of functions. finite difference estimation of derivative. in numerical analysis, numerical differentiation algorithms estimate the derivative of a mathematical function or function subroutine using values of the function and perhaps other knowledge about the function. Section 4.1 numerical differentiation . 2 . motivation. • consider to solve black scholes equation . example 4.4.1 use forward difference formula with ℎ= 0.1 to. Example 2.2.1.1. the velocity of a rocket is given by. v(t) = 2000ln[14 × 104 14 × 104 − 2100t] − 9.8t, 0 ≤ t ≤ 30. where v is given in m s and t is given in seconds. at t = 16 s, a) use the forward difference approximation of the first derivative of v(t) to calculate the acceleration. use a step size of h = 2 s. 9.1 numerical differentiation. how can we find a good approximation to the derivative of a function? the obvious approach is to pick a very small d d and calculate \frac {f (x d) f (x)} {d} df (x d)−f (x), which looks like the definition of the derivative. actually, this is not a great idea. why?.
Ppt Numerical Differentiation Powerpoint Presentation Free Download Example 2.2.1.1. the velocity of a rocket is given by. v(t) = 2000ln[14 × 104 14 × 104 − 2100t] − 9.8t, 0 ≤ t ≤ 30. where v is given in m s and t is given in seconds. at t = 16 s, a) use the forward difference approximation of the first derivative of v(t) to calculate the acceleration. use a step size of h = 2 s. 9.1 numerical differentiation. how can we find a good approximation to the derivative of a function? the obvious approach is to pick a very small d d and calculate \frac {f (x d) f (x)} {d} df (x d)−f (x), which looks like the definition of the derivative. actually, this is not a great idea. why?. Solution. a) to find the acceleration at t = 15 s with the forward divided difference method, a data point ahead of t = 15 s should be available. all these conditions are met, and we will use velocity values at t = 15 s and t = 20 s. a(ti) ≈ v(ti 1) − v(ti) ti 1 − ti. ti = 15. Numerical differentiation is the process of finding the numerical value of a derivative of a given function at a given point. in general, numerical differentiation is more difficult than numerical integration. this is because while numerical integration requires only good continuity properties of the function being integrated, numerical differentiation requires more complicated properties such.

Numerical Differentiation Examples Youtube Solution. a) to find the acceleration at t = 15 s with the forward divided difference method, a data point ahead of t = 15 s should be available. all these conditions are met, and we will use velocity values at t = 15 s and t = 20 s. a(ti) ≈ v(ti 1) − v(ti) ti 1 − ti. ti = 15. Numerical differentiation is the process of finding the numerical value of a derivative of a given function at a given point. in general, numerical differentiation is more difficult than numerical integration. this is because while numerical integration requires only good continuity properties of the function being integrated, numerical differentiation requires more complicated properties such.

Numerical Differentiation

Ppt Chapter 4 Numerical Differentiation And Integration Powerpoint

Comments are closed.