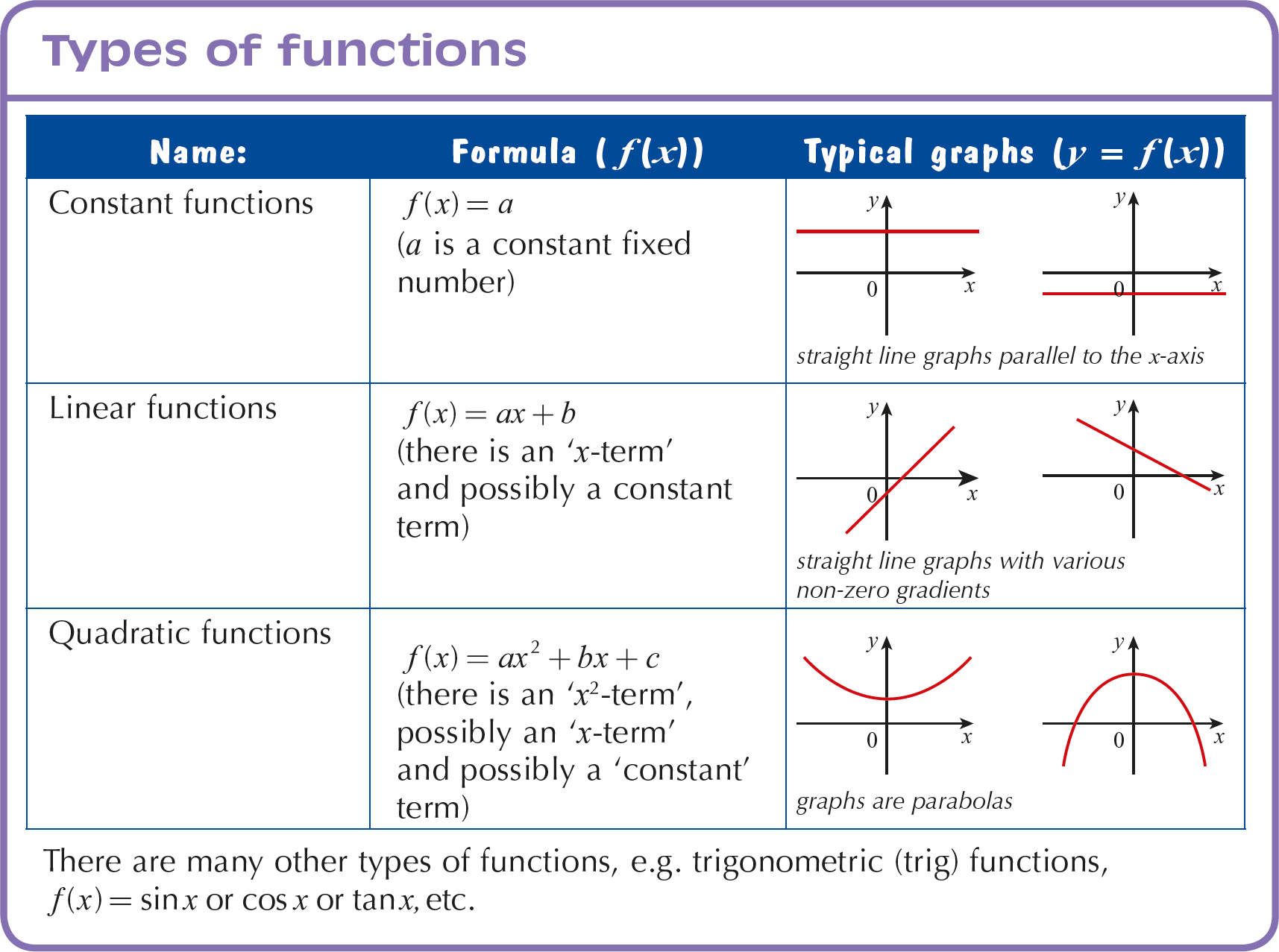
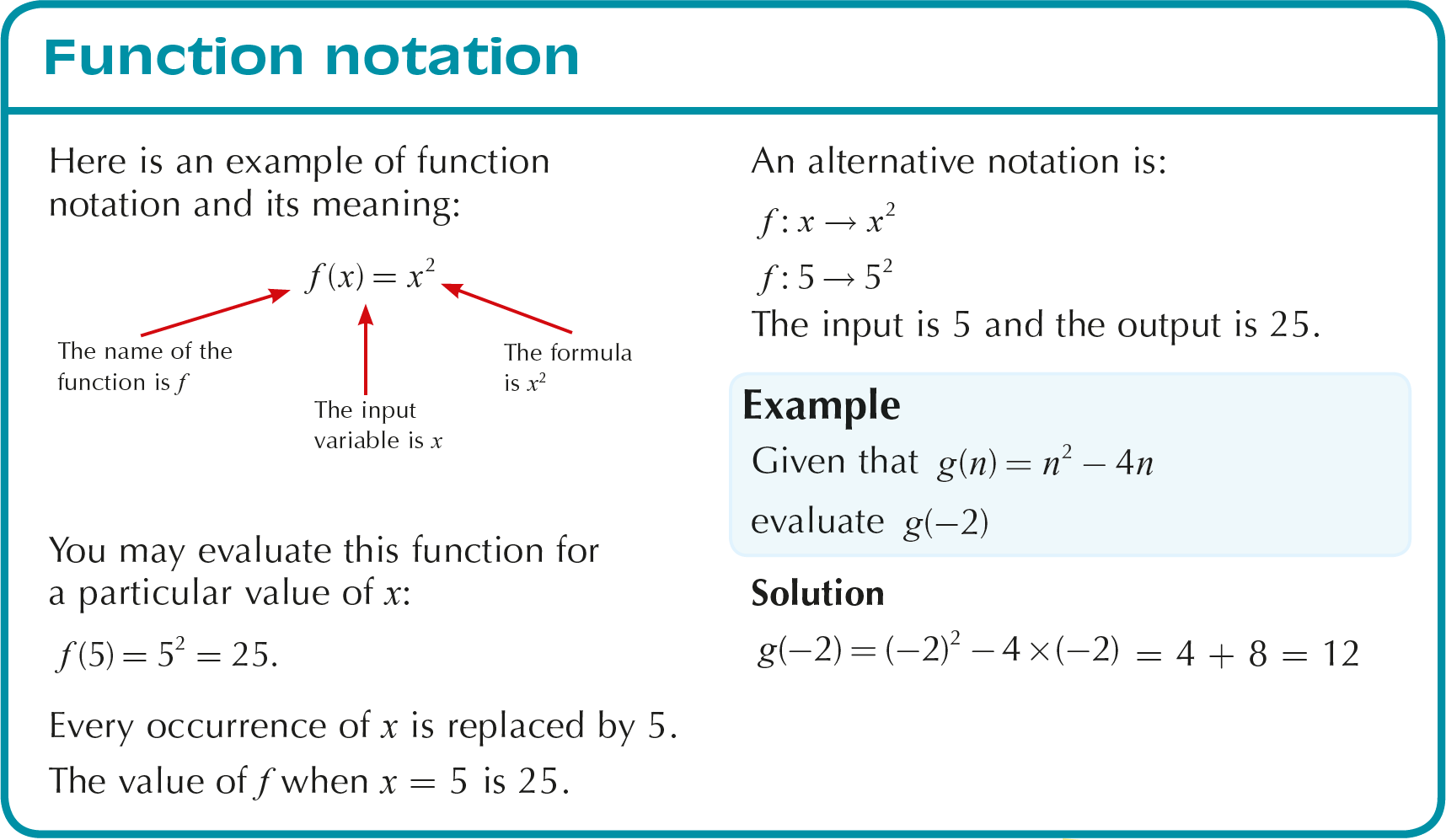
Notes On Functions
Working With Functions Including Function Notation Graph Of A F: a 2 5(a 2) − 3. using modern function notation, we would write. f(a 2) = 5(a 2) − 3. note that this is again a simple substitution, where we replace each occurrence of x in the formula f(x) = 5x − 3 with the expression a 2. finally, use the distributive property to first multiply by 5, then subtract 3. A function is a relation in which each member of the domain is paired with exactly one element of the range. all functions are relations, but not all relations are functions. a good example of a functional relation can be seen in the linear equation y = x 1, graphed in figure 3.
Working With Functions Including Function Notation Graph Of A This topic covers: evaluating functions domain & range of functions graphical features of functions average rate of change of functions function combination and composition function transformations (shift, reflect, stretch) piecewise functions inverse functions two variable functions. Also, in a function, there can’t be two pairs with the same first element. condition for a function. set a and set b should be non empty. in a function, a particular input is given to get a particular output. so, a function f: a >b denotes that f is a function from a to b, where a is a domain, and b is a co domain. Introduction to functions. mc ty introfns 2009 1. a function is a rule which operates on one number to give another number. however, not every rule describes a valid function. this unit explains how to see whether a given rule describes a valid function, and introduces some of the mathematical terms associated with functions. As with several of our previous topics, we have some special functions that will show up from time to time, and will be quite useful. de nition 3. the identity function on a set x, denoted by x, is the function x: x!xsuch that 8x2x; f(x) = x. the empty function is any function f: ;!x. note that there is no need, in the empty function, to.

Exponential Functions Notes And Worksheets Lindsay Bowden Introduction to functions. mc ty introfns 2009 1. a function is a rule which operates on one number to give another number. however, not every rule describes a valid function. this unit explains how to see whether a given rule describes a valid function, and introduces some of the mathematical terms associated with functions. As with several of our previous topics, we have some special functions that will show up from time to time, and will be quite useful. de nition 3. the identity function on a set x, denoted by x, is the function x: x!xsuch that 8x2x; f(x) = x. the empty function is any function f: ;!x. note that there is no need, in the empty function, to. All throughout a calculus course we will be finding roots of functions. a root of a function is nothing more than a number for which the function is zero. in other words, finding the roots of a function, g(x), is equivalent to solving. g(x) = 0. example 3 determine all the roots of f(t) = 9t3 − 18t2 6t. Figure 1.1.1 compares relations that are functions and not functions. figure 1.1.1: (a) this relationship is a function because each input is associated with a single output. note that input q and r both give output n. (b) this relationship is also a function. in this case, each input is associated with a single output.

Functions Relations Guided Notes By Mrstech Teachers Pay Teachers All throughout a calculus course we will be finding roots of functions. a root of a function is nothing more than a number for which the function is zero. in other words, finding the roots of a function, g(x), is equivalent to solving. g(x) = 0. example 3 determine all the roots of f(t) = 9t3 − 18t2 6t. Figure 1.1.1 compares relations that are functions and not functions. figure 1.1.1: (a) this relationship is a function because each input is associated with a single output. note that input q and r both give output n. (b) this relationship is also a function. in this case, each input is associated with a single output.

Fillable Online Intro To Functions Notes Pdf Fax Email Print Pdffiller

Comments are closed.