Multiplying Exponents Made Easy
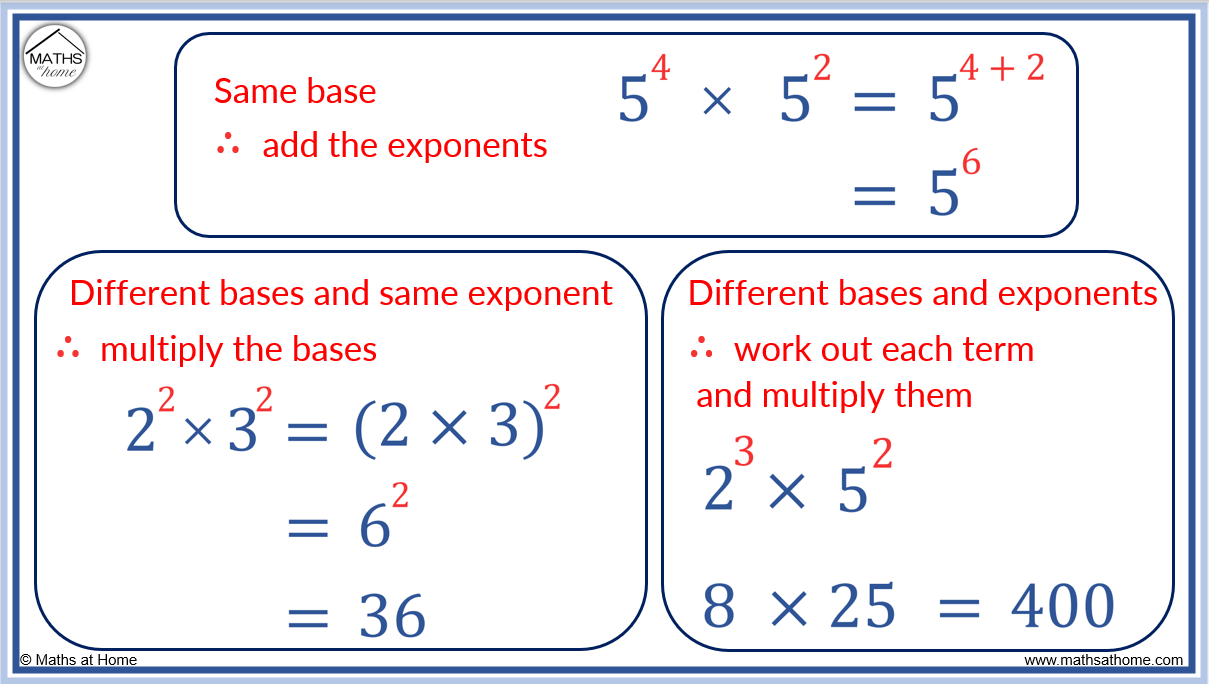
A Complete Guide To Multiplying Exponents Mathsathome Exponents. the exponent of a number says how many times to use the number in a multiplication. in words: 8 2 could be called "8 to the power 2" or "8 to the second power", or simply "8 squared". exponents make it easier to write and use many multiplications. example: 96 is easier to write and read than 9 × 9 × 9 × 9 × 9 × 9. There are two methods to multiply terms involving exponents, when the bases are the same, add the exponents. for example, a 3 × a 4 = a 3 4 = a 7a3 × a4 = a3 4 = a7. these questions usually ask to ‘simplify’ the calculation. when the bases are different, multiply the coefficients and keep the exponent the same.
A Complete Guide To Multiplying Exponents Mathsathome Laws of exponents. exponents are also called powers or indices. the exponent of a number says how many times to use the number in a multiplication. in this example: 82 = 8 × 8 = 64. in words: 8 2 could be called "8 to the second power", "8 to the power 2" or simply "8 squared". try it yourself:. According to the rules of multiplying exponents, when the bases are the same, we add the powers. 5 3 × 5 2 = 5 2 3 = 5 5 = 3125. example 3: state true or false with reference to the multiplication of exponents. a.) when the terms with the same base are multiplied, the powers are added. b.) 4 2 × 4 5 = 4 10. Community answer. 10^4 = 10 x 10 x 10 x 10 = 10,000, so you are really multiplying 3.5 x 10,000. the shortcut is that, when 10 is raised to a certain power, the exponent tells you how many zeros. 10^4 = 1 followed by 4 zeros = 10,000. thus, you can just move the decimal point to the right 4 spaces: 3.5 x 10^4 = 35,000. Multiplying exponents with the same base. when we multiply two expressions with the same base, we apply the rule, a m × a n = a (m n), in which 'a' is the common base and 'm' and 'n' are the exponents. for example, let us multiply 2 2 × 2 3. using the rule, 2 2 × 2 3 = 2 (2 3) = 2 5. multiplying exponents with different base and same power.
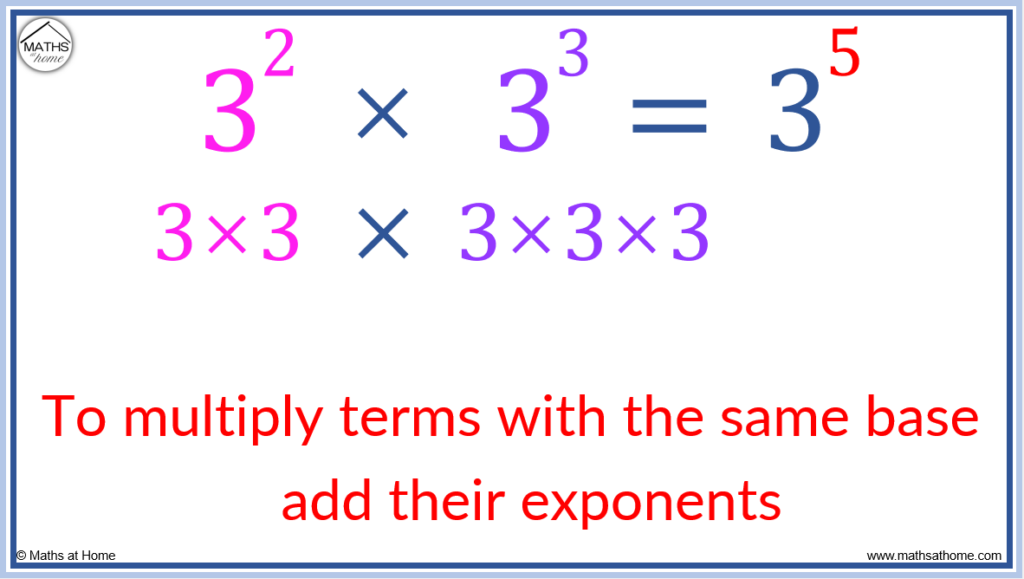
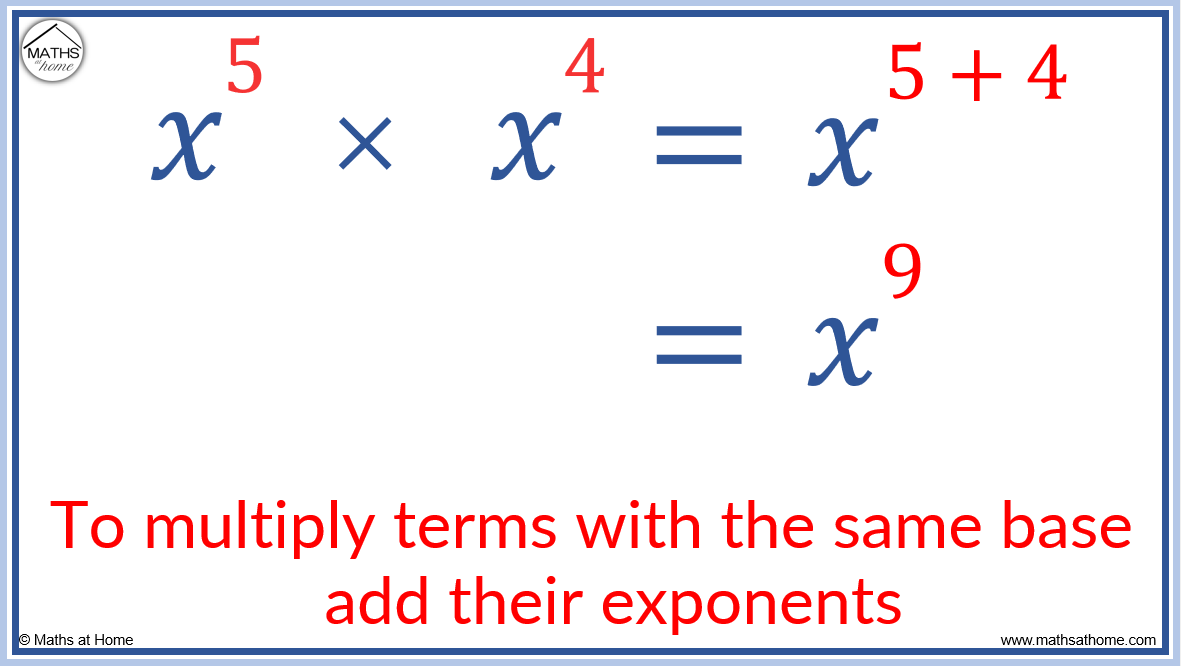
A Complete Guide To Multiplying Exponents Mathsathome Community answer. 10^4 = 10 x 10 x 10 x 10 = 10,000, so you are really multiplying 3.5 x 10,000. the shortcut is that, when 10 is raised to a certain power, the exponent tells you how many zeros. 10^4 = 1 followed by 4 zeros = 10,000. thus, you can just move the decimal point to the right 4 spaces: 3.5 x 10^4 = 35,000. Multiplying exponents with the same base. when we multiply two expressions with the same base, we apply the rule, a m × a n = a (m n), in which 'a' is the common base and 'm' and 'n' are the exponents. for example, let us multiply 2 2 × 2 3. using the rule, 2 2 × 2 3 = 2 (2 3) = 2 5. multiplying exponents with different base and same power. To multiply terms with the same base, add their exponents. for example, 𝑥5 × 𝑥4 = 𝑥9, since 5 4 = 9. which simplifies as . to multiply exponents with the same base, keep the base the same and only add the exponents. for example, 32 × 33 = 35. notice that we add the exponents of 2 3 = 5 but we keep the base number as 3. In this video, we're going to teach you how to multiply exponents an essential skill for math students. we're going to walk you through the steps, step by.

Comments are closed.