More Product And Quotient Rule Practice And Higher Order Derivatives

More Product And Quotient Rule Practice And Higher Order Derivatives 3. derivatives. 3.1 the definition of the derivative; 3.2 interpretation of the derivative; 3.3 differentiation formulas; 3.4 product and quotient rule; 3.5 derivatives of trig functions; 3.6 derivatives of exponential and logarithm functions; 3.7 derivatives of inverse trig functions; 3.8 derivatives of hyperbolic functions; 3.9 chain rule. Use the product rule to compute the derivative of y = 5x2sinx. evaluate the derivative at x = π 2. solution. to make our use of the product rule explicit, let's set f(x) = 5x2 and g(x) = sinx. we easily compute recall that f′(x) = 10x and g′(x) = cosx. employing the rule, we have d dx(5x2sinx) = 5x2cosx 10xsinx.

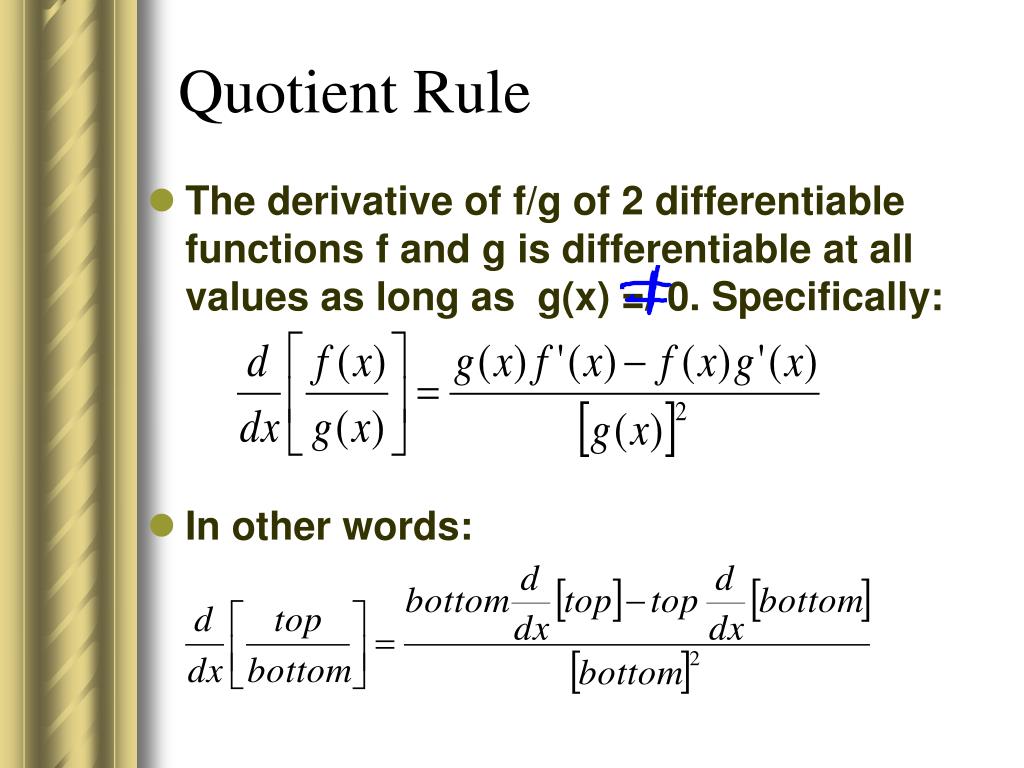
Ppt 2 3 The Product Rule Quotient Rule And Higher Order Derivatives When applying the product or quotient rule to find higher order derivatives, use the previously found lower order derivatives in the formula; common applications. the product and quotient rules are essential for finding derivatives of more complex functions. Quotient rule. if the two functions f (x) f (x) and g(x) g (x) are differentiable (i.e. the derivative exist) then the quotient is differentiable and, (f g)′ = f ′g −f g′ g2 (f g) ′ = f ′ g − f g ′ g 2. note that the numerator of the quotient rule is very similar to the product rule so be careful to not mix the two up!. The quotient rule. having developed and practiced the product rule, we now consider differentiating quotients of functions. as we see in the following theorem, the derivative of the quotient is not the quotient of the derivatives; rather, it is the derivative of the function in the numerator times the function in the denominator minus the derivative of the function in the denominator times the. Higher order derivatives the nth derivative for any positive integer n, the nth derivative of a function is obtained from the function by differentiating successively n times. if the original function is y =f(x), the nth derivative is denoted by dny dxn or f(n)(x) example find f(4)(x)if f(x)=x6 −2x5 x4 −3x3 5x −6.

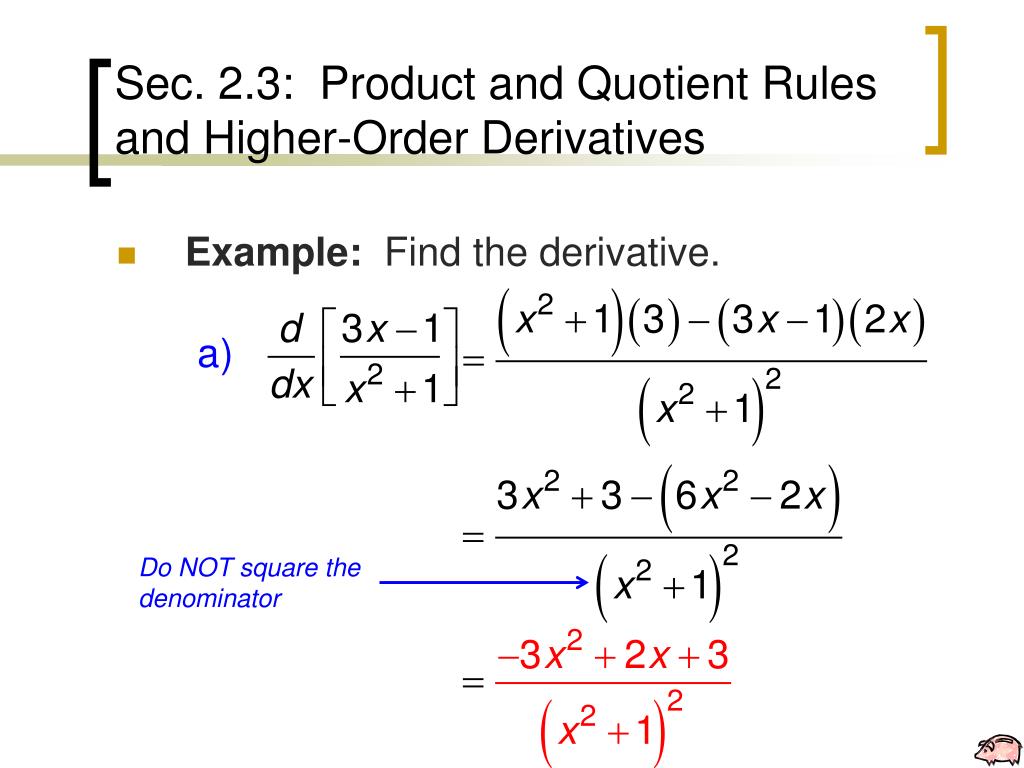
Ppt Sec 2 3 Product And Quotient Rules And Higher Order Derivatives The quotient rule. having developed and practiced the product rule, we now consider differentiating quotients of functions. as we see in the following theorem, the derivative of the quotient is not the quotient of the derivatives; rather, it is the derivative of the function in the numerator times the function in the denominator minus the derivative of the function in the denominator times the. Higher order derivatives the nth derivative for any positive integer n, the nth derivative of a function is obtained from the function by differentiating successively n times. if the original function is y =f(x), the nth derivative is denoted by dny dxn or f(n)(x) example find f(4)(x)if f(x)=x6 −2x5 x4 −3x3 5x −6. The second derivative the second derivative of a function is the derivative of its derivative. if y = f(x), the second derivative is denoted by d2y dx2 or f00(x) the second derivative gives the rate of change of the rate of change of the original function. example find the second derivative of f(x) = x10 4x6 27x 4. The quotient rule. having developed and practiced the product rule, we now consider differentiating quotients of functions. as we see in the following theorem, the derivative of the quotient is not the quotient of the derivatives; rather, it is the derivative of the function in the numerator times the function in the denominator minus the derivative of the function in the denominator times the.

Product And Quotient Rule Derivatives Worksheet Day 2 By Sarah Miller The second derivative the second derivative of a function is the derivative of its derivative. if y = f(x), the second derivative is denoted by d2y dx2 or f00(x) the second derivative gives the rate of change of the rate of change of the original function. example find the second derivative of f(x) = x10 4x6 27x 4. The quotient rule. having developed and practiced the product rule, we now consider differentiating quotients of functions. as we see in the following theorem, the derivative of the quotient is not the quotient of the derivatives; rather, it is the derivative of the function in the numerator times the function in the denominator minus the derivative of the function in the denominator times the.