Modelling With First Order Differential Equations Ordinary Differential Equations Lecture 5

Modelling With First Order Differential Equations Ordinary In this lecture we demonstrate how to derive a differential equation that describes the amount of salt in a tank of water. the problem is carefully explained. Differential equations. most of our models will be initial value problems. additional required mathematics after first order ode’s (and solution of second order ode’s by first order techniques) is linear algebra. all of these must be mastered in order to understand the development and solution of mathematical models in science and engineering.

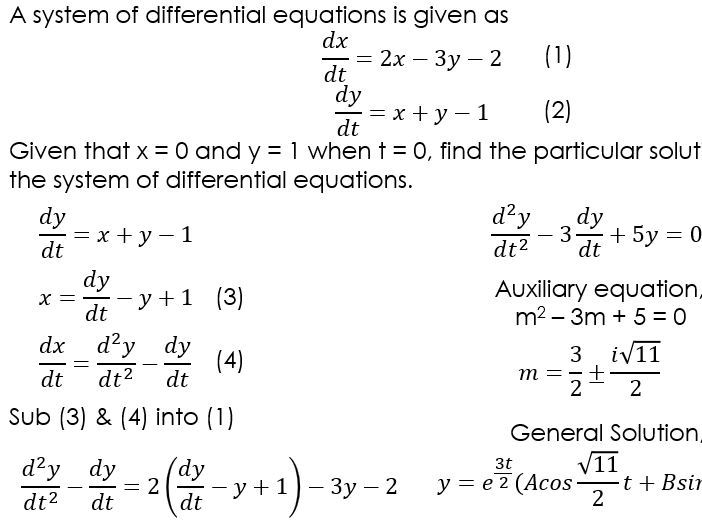
Modelling With Differential Equations Teaching Resources In this section we will use first order differential equations to model physical situations. in particular we will look at mixing problems (modeling the amount of a substance dissolved in a liquid and liquid both enters and exits), population problems (modeling a population under a variety of situations in which the population can enter or exit) and falling objects (modeling the velocity of a. First order odes 5 intuitively, the ode wants to push solutions towards the line y= 1=t:note that y ‘ is not a solution to the ode, unlike the equilibrium points of the rst example. 0 2 4 6 0 0.5 1 1.5 2 0 2 4 6 0 0.5 1 1.5 2 3. separable equations even rst order odes are complicated enough that exact solutions are not easy to obtain. 2 chapter 1. first order single differential equations (ii)how to solve the corresponding differential equations, (iii)how to interpret the solutions, and (iv)how to develop general theory. 1.2 relaxation and equilibria the most simplest and important example which can be modeled by ode is a relaxation process. Introduction to differential equations and mathematical modeling, and a technique for solving first order linear ode’s 1. introduction to differential equations and mathematical modeling 2. useful characteristics of ode's 3. technique for solving first order linear ode’s using an integrating factor sr 1. brief historical remarks on.

First Order Differential Equations Teaching Resources 2 chapter 1. first order single differential equations (ii)how to solve the corresponding differential equations, (iii)how to interpret the solutions, and (iv)how to develop general theory. 1.2 relaxation and equilibria the most simplest and important example which can be modeled by ode is a relaxation process. Introduction to differential equations and mathematical modeling, and a technique for solving first order linear ode’s 1. introduction to differential equations and mathematical modeling 2. useful characteristics of ode's 3. technique for solving first order linear ode’s using an integrating factor sr 1. brief historical remarks on. Section 1.2: solutions of some differential equations. section 1.3: linear versus nonlinear ordinary differential equations. section 2.1: method of integrating factors to solve first order liner ode. section 2.2: separable equations and homegeneous equations. section 2.3: modeling with first order differential equations. 1.1.3 ordinary differential equations an ordinary differential equation (ode) is an equation that involves the derivatives of a dependent variable y(x) with respect to a single independent variable x, e.g. y0= dy=dx, y00= d2y=dx2. the equation may also involve yitself and some given functions of x.

Ordinary Differential Equations Used In The First Model Download Table Section 1.2: solutions of some differential equations. section 1.3: linear versus nonlinear ordinary differential equations. section 2.1: method of integrating factors to solve first order liner ode. section 2.2: separable equations and homegeneous equations. section 2.3: modeling with first order differential equations. 1.1.3 ordinary differential equations an ordinary differential equation (ode) is an equation that involves the derivatives of a dependent variable y(x) with respect to a single independent variable x, e.g. y0= dy=dx, y00= d2y=dx2. the equation may also involve yitself and some given functions of x.