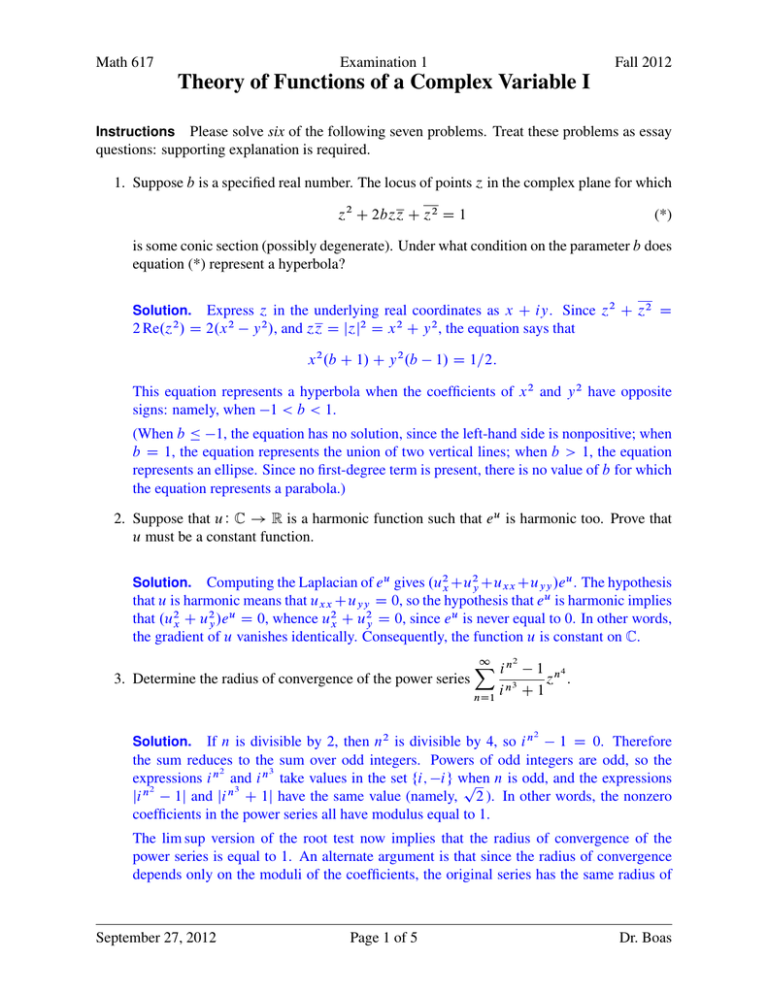
Librarika Functions Of A Complex Variable Library Of Mathematics

Librarika Functions Of A Complex Variable Library Of Mathematics A function ƒ () of a complex variable is said to have a derivative (be differentiable) at the point if lim ξ→z ƒ (ξ)−ƒ (z) ξ−z 2 1 exists and has the same value for any mode of approach of ξ to . this limit is called the derivative, and it is denoted by ƒ′ () or df dz. the formalism of (2 1) is the same as in the real. Functions of a complex variable are used to solve applications in various branches of mathematics, science, and engineering. functions of a complex variable: theory and technique is a book in a special category of influential classics because it is based on the authors' extensive experience in modeling complicated situations and providing analytic solutions.
Theory Of Functions Of A Complex Variable I Many common functions fof the real variable xare defined by giving a rule for calculating f through algebraic operations on x, e.g. f(x) = x2 −1, or f(x) = ex≡ p nx n n!. replacing x with the complex variable zwe obtain from each such rule a complex function f(z). if f(x) is differentiable, this complex extension is analytic: f(z) = u iv. This chapter contains sections titled: elementary properties of analytic functions. integration in the complex plane. analytic functions. calculus of residues: applications. periodic functions; fourier series. summary this chapter contains sections titled: elementary properties of analytic functions integration in the complex plane analytic. Functions of a complex variable provides an elementary introduction to the theory of differentiation and integration of complex functions and forms a sequel to complex numbers by walter ledermann. the book includes the standard topics to be found in a first university course in complex variable theory. Complex variable theory. d. crighton a. dowling j. williams m. heckl f. g. leppington. mathematics, physics. 1992. some techniques of complex variable theory, particularly contour integration, provide useful methods for dealing with certain problems of wave theory and are outlined in the present chapter. it is….
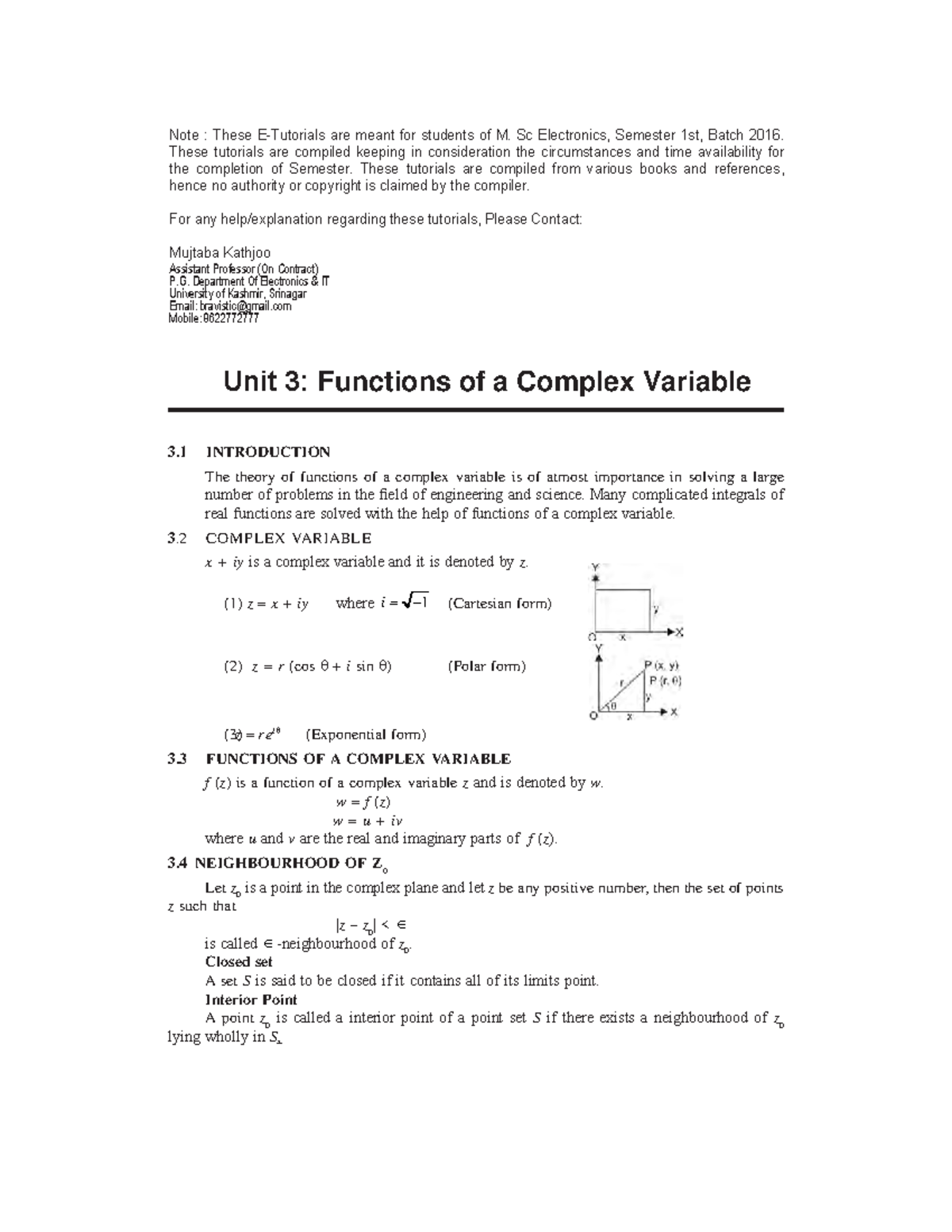
Functions Of A Complex Variable 1 Introduction The Theory Of Functions of a complex variable provides an elementary introduction to the theory of differentiation and integration of complex functions and forms a sequel to complex numbers by walter ledermann. the book includes the standard topics to be found in a first university course in complex variable theory. Complex variable theory. d. crighton a. dowling j. williams m. heckl f. g. leppington. mathematics, physics. 1992. some techniques of complex variable theory, particularly contour integration, provide useful methods for dealing with certain problems of wave theory and are outlined in the present chapter. it is…. Chapters 5 through 7 cover special functions such as the gamma function, laplace's method and other asymptotic methods, and transform methods such as the fourier transform, with the final chapter presenting some special topics such as the wiener hopf technique (extremely useful in many engineering and mathematical physics applications). A₁ absolutely convergent analytic continuation analytic function arg ƒ bilinear transformation bounded c₁ cauchy riemann equations cauchy's theorem centre change in arg circle of convergence complex numbers constant contour corresponds cosh defined denote domain entire function equating real essential singularity example finite number.

Functions Of A Complex Variable 1st Edition Hemant Kumar Pathak Chapters 5 through 7 cover special functions such as the gamma function, laplace's method and other asymptotic methods, and transform methods such as the fourier transform, with the final chapter presenting some special topics such as the wiener hopf technique (extremely useful in many engineering and mathematical physics applications). A₁ absolutely convergent analytic continuation analytic function arg ƒ bilinear transformation bounded c₁ cauchy riemann equations cauchy's theorem centre change in arg circle of convergence complex numbers constant contour corresponds cosh defined denote domain entire function equating real essential singularity example finite number.

Theory Of Functions Of A Complex Variable I

Librarika Functions Of A Complex Variable Library Of Mathematics

Comments are closed.