Learn The Associative Property Of Addition And Multiplication Youtube

Learn The Associative Property Of Addition And Multiplication Youtube Learn the associative property of addition and multiplication. this property indicates that you can add or multiply regardless of how the numbers are grouped. In this lesson, you will learn about the associate property of multiplication by exploring an example of the associative property. free practice worksheet:.

How To Use The Associative Property Of Addition And Multiplication Rootmath.org | algebrathe associative property tells us that is doesn't matter how we group addition or multiplication. the property can be writt. Identity property (or zero property) of addition. when you add 0 to any a number, the sum is that number. for example: 325 0 = 325. identity property (or one property) of multiplication. when you multiply any number by 1, the product is that number. for example: 65, 148 × 1 = 65, 148. zero property of multiplication. Example 2: simple associative property with multiplication. use the associative property to solve 7 × 4 × 5.7 × 4 × 5. check to see that the operation is addition or multiplication. show step. all the numbers are being multiplied, so the associative property can be used. change the grouping of the numbers and solve. Associative comes from the word “associate”. try to remember that “associate”, in terms of math, refers to grouping with parentheses. in other words, in an example of the associative property, the numbers will stay in the same order, but the parentheses will move.

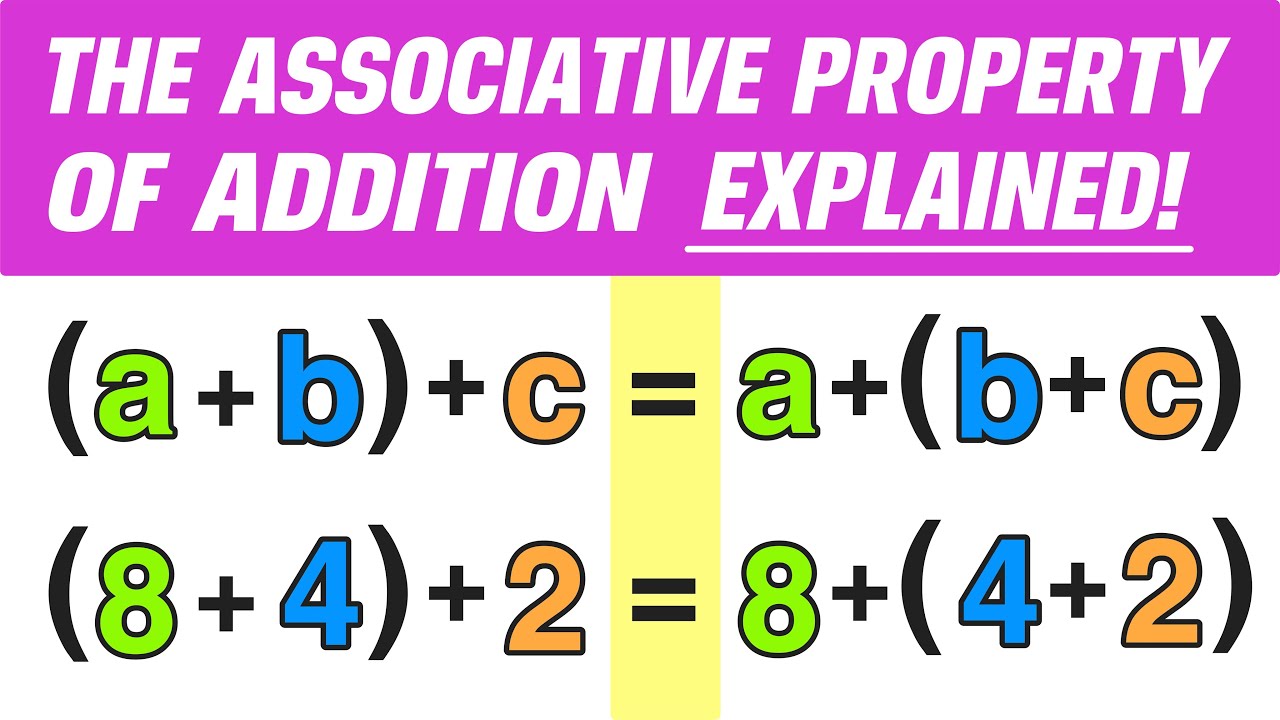
The Associative Property Of Addition Explained Youtube Example 2: simple associative property with multiplication. use the associative property to solve 7 × 4 × 5.7 × 4 × 5. check to see that the operation is addition or multiplication. show step. all the numbers are being multiplied, so the associative property can be used. change the grouping of the numbers and solve. Associative comes from the word “associate”. try to remember that “associate”, in terms of math, refers to grouping with parentheses. in other words, in an example of the associative property, the numbers will stay in the same order, but the parentheses will move. Similarly, the associative property of multiplication states that \((a \cdot b) \cdot c = a \cdot (b \cdot c)\). the associative property essentially means that the order in which we perform several additions (or multiplications) does not matter, which allows us to more simply write the above expressions as \(a b c\) and \(a \cdot b \cdot c. In this basic lesson i explain briefly what the commutative and associative properties of addition & multiplication mean. then we check if subtraction & division also have those properties — turns out they don't. lastly, i simplify various expressions using the commutative and associative properties of addition and multiplication.

The Associative Property Of Multiplication Explained Youtube Similarly, the associative property of multiplication states that \((a \cdot b) \cdot c = a \cdot (b \cdot c)\). the associative property essentially means that the order in which we perform several additions (or multiplications) does not matter, which allows us to more simply write the above expressions as \(a b c\) and \(a \cdot b \cdot c. In this basic lesson i explain briefly what the commutative and associative properties of addition & multiplication mean. then we check if subtraction & division also have those properties — turns out they don't. lastly, i simplify various expressions using the commutative and associative properties of addition and multiplication.