Intuitive Explanation Of Maxwell Electromagnetic Equations
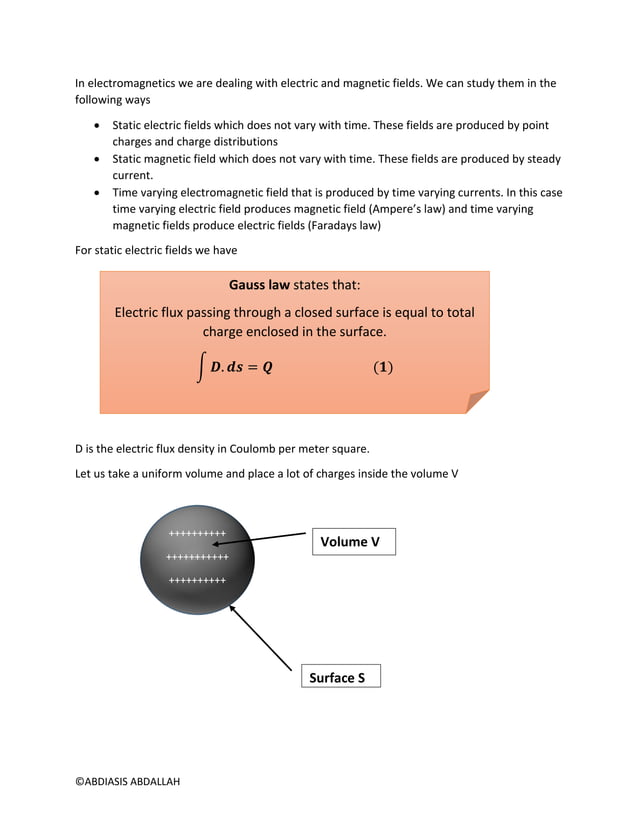
Intuitive Explanation Of Maxwell Electromagnetic Equations An intuitive explanation of lorentz force law and maxwell's equations in integral form and differential form, with focus on their interconnections and individual roles. faraday's law of electromagnetic induction, derivation and misconceptions. James clerk maxwell [1831 1879] was an einstein newton level genius who took a set of known experimental laws (faraday's law, ampere's law) and unified them into a symmetric coherent set of equations known as maxwell's equations. maxwell was one of the first to determine the speed of propagation of electromagnetic (em) waves was the same as the.

Maxwell S Equations An Intuitive View For Engineers Figure 1. maxwell equations: four lines that provide a complete description of light, electricity and magnetism. physicists are fond of abstracting concepts into mathematical expressions and operators. on the other hand, we, engineers, we like to understand concepts and feed formulas to computer simulators. This is extremely similar to maxwell faraday’s law. it’s pretty much its opposite. it governs how a changing \bold {e} e field affect \bold {b} b field, or generate a curl in \bold {b} b field. it gives the direction \bold {e}\rightarrow \bold {b} e → b. however, there’s a bit more to it: the equation also involves \bold {j} j current. They tell us about the sources and sinks of the fields. the two equations involving the curl have time derivative terms and a current term. the time derivative terms describe electromagnetic induction. their signs are opposite, which is what allows negative feedback so we can have oscillating electromagnetic waves. Electromagnetism. maxwell's equations, or maxwell–heaviside equations, are a set of coupled partial differential equations that, together with the lorentz force law, form the foundation of classical electromagnetism, classical optics, electric and magnetic circuits. the equations provide a mathematical model for electric, optical, and radio.
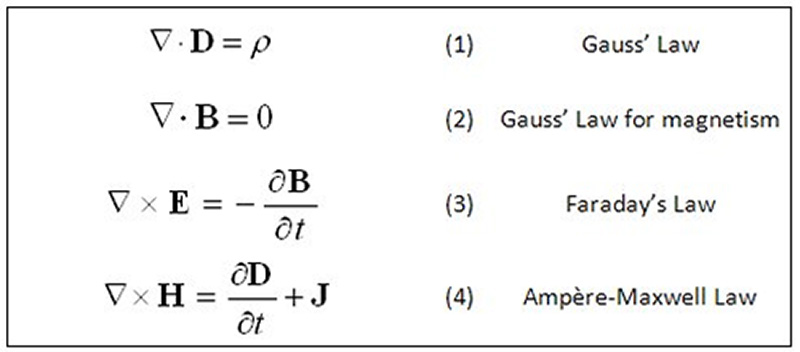
An Intuitive View Of Maxwell S Equations Electrical Engineering News They tell us about the sources and sinks of the fields. the two equations involving the curl have time derivative terms and a current term. the time derivative terms describe electromagnetic induction. their signs are opposite, which is what allows negative feedback so we can have oscillating electromagnetic waves. Electromagnetism. maxwell's equations, or maxwell–heaviside equations, are a set of coupled partial differential equations that, together with the lorentz force law, form the foundation of classical electromagnetism, classical optics, electric and magnetic circuits. the equations provide a mathematical model for electric, optical, and radio. Until maxwell’s work, the known laws of electricity and magnetism were those we have studied in chapters 3 through 17.in particular, the equation for the magnetic field of steady currents was known only as \begin{equation} \label{eq:ii:18:1} \flpcurl{\flpb}=\frac{\flpj}{\epso c^2}. \end{equation} maxwell began by considering these known laws and expressing them as differential equations, as. Magnetic fields are generated by moving charges or by changing electric fields. this fourth of maxwell’s equations, equation 16.2.17, encompasses ampère’s law and adds another source of magnetic fields, namely changing electric fields. maxwell’s equations and the lorentz force law together encompass all the laws of electricity and magnetism.

Maxwell S Equations And Displacement Current Owlcation Until maxwell’s work, the known laws of electricity and magnetism were those we have studied in chapters 3 through 17.in particular, the equation for the magnetic field of steady currents was known only as \begin{equation} \label{eq:ii:18:1} \flpcurl{\flpb}=\frac{\flpj}{\epso c^2}. \end{equation} maxwell began by considering these known laws and expressing them as differential equations, as. Magnetic fields are generated by moving charges or by changing electric fields. this fourth of maxwell’s equations, equation 16.2.17, encompasses ampère’s law and adds another source of magnetic fields, namely changing electric fields. maxwell’s equations and the lorentz force law together encompass all the laws of electricity and magnetism.

Most Intuitive Understanding Of The Maxwell Equations Youtube

Comments are closed.