Integration By Substitution Introduction
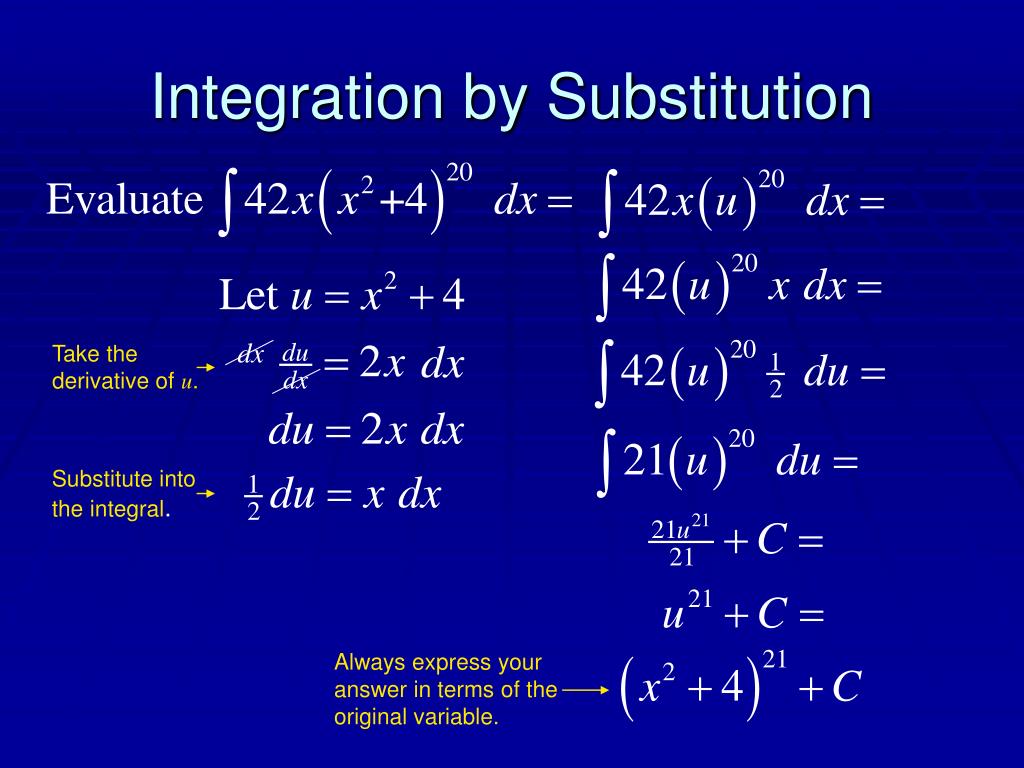
Ppt Integration By Substitution Powerpoint Presentation Free When our integral is set up like that, we can do this substitution: then we can integrate f (u), and finish by putting g (x) back as u. like this: example: ∫ cos (x 2) 2x dx. we know (from above) that it is in the right form to do the substitution: now integrate: ∫ cos (u) du = sin (u) c. Example 4.1.1: integrating by substitution. evaluate ∫ xsin(x2 5) dx. solution. knowing that substitution is related to the chain rule, we choose to let u be the "inside" function of sin(x2 5). (this is not always a good choice, but it is often the best place to start.) let u = x2 5, hence du = 2xdx.

Integration By Substitution Introduction Youtube Ubstitution35.1. introductionthe chain rule provides a method for replacing a complicated. ntegral by a simpler integral. the method is called integration by substitution (\integration" is. the act of nding an integral). examplezfind cos(x 1) dx:solution we know a rule that comes close to working here, namely, r cos x dx = sin x c, but w. Calculus. in calculus, integration by substitution, also known as u substitution, reverse chain rule or change of variables, [1] is a method for evaluating integrals and antiderivatives. it is the counterpart to the chain rule for differentiation, and can loosely be thought of as using the chain rule "backwards.". Let u = cos(θ) u = cos (θ) and take differentials of both sides. substitute these into the integral and see if you can simplify. theorem: substitution method for indefinite integrals. if u = g(x) is a differentiable function whose range is an interval i and f is continuous on i, then. ∫f(g(x))g′(x)dx = ∫f(u)du. The following are the steps that are helpful in performing this method of integration by substitution. step 1: choose a new variable t for the given function to be reduced. step 2: determine the value of dx, of the given integral, where f (x) is integrated with respect to x. step 3: make the required substitution in the function f (x.
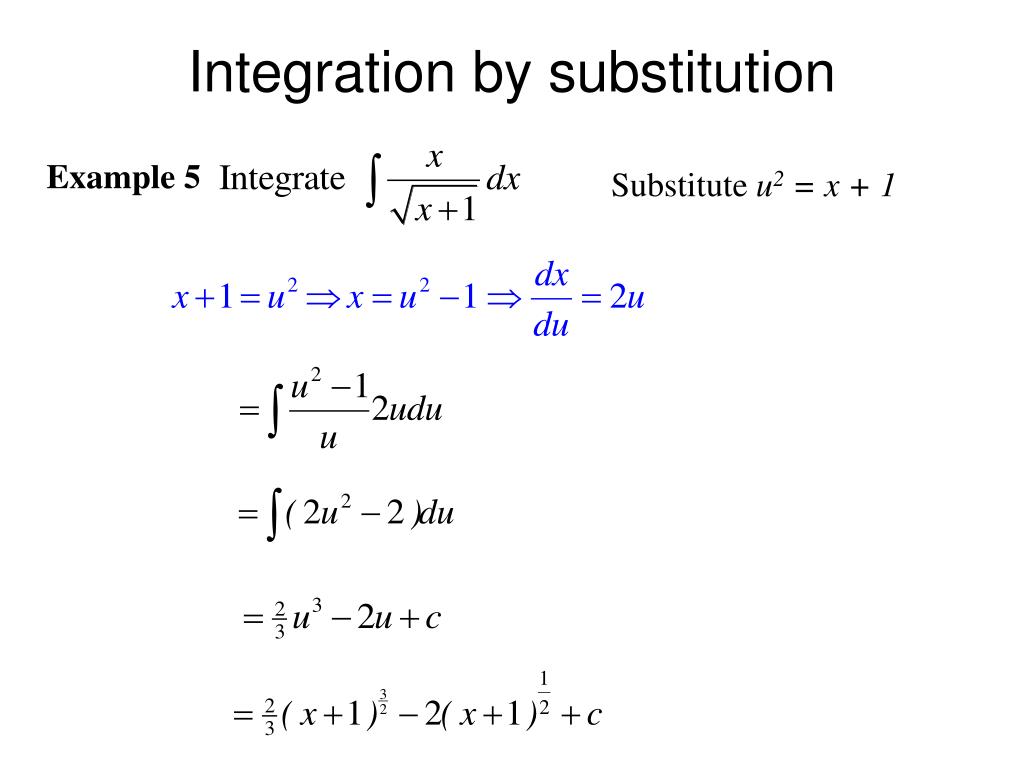
Ppt Integration By Substitution Powerpoint Presentation Free Let u = cos(θ) u = cos (θ) and take differentials of both sides. substitute these into the integral and see if you can simplify. theorem: substitution method for indefinite integrals. if u = g(x) is a differentiable function whose range is an interval i and f is continuous on i, then. ∫f(g(x))g′(x)dx = ∫f(u)du. The following are the steps that are helpful in performing this method of integration by substitution. step 1: choose a new variable t for the given function to be reduced. step 2: determine the value of dx, of the given integral, where f (x) is integrated with respect to x. step 3: make the required substitution in the function f (x. Guidelines for integration by substitution. 1. let u be a function of x (usually part of the integrand). 2. solve for x and dx in terms of u and du. 3. convert the entire integral to u variable form and try to fit it to one or more of the basic integration formulas. if none fits, try a different substitution. In calculus, the integration by substitution method is also known as the “reverse chain rule” or “u substitution method”. we can use this method to find an integral value when it is set up in the special form. it means that the given integral is of the form: ∫ f (g (x)).g' (x).dx = f (u).du.

Introduction To Integration By Substitution Youtube Guidelines for integration by substitution. 1. let u be a function of x (usually part of the integrand). 2. solve for x and dx in terms of u and du. 3. convert the entire integral to u variable form and try to fit it to one or more of the basic integration formulas. if none fits, try a different substitution. In calculus, the integration by substitution method is also known as the “reverse chain rule” or “u substitution method”. we can use this method to find an integral value when it is set up in the special form. it means that the given integral is of the form: ∫ f (g (x)).g' (x).dx = f (u).du.

Integration By Substitution An Introduction Year 2 Maths Pure

Comments are closed.