Illustrative Math Algebra 2 Unit 5 Lesson 6 Teerani
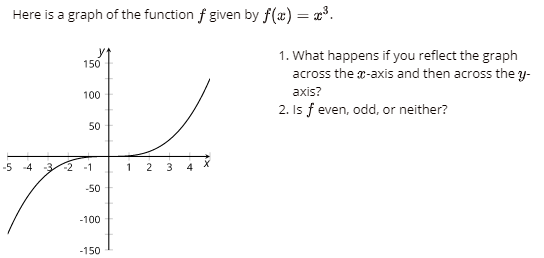
Illustrative Math Algebra 2 Unit 5 Lesson 6 Teerani Algebra 2 unit 1 unit 2 unit 3 unit 4 unit 5 unit 6 unit 7 alg2.5 transformations of functions in this unit, students consider functions as a whole and understand how they can be transformed to fit the needs of a situation, which is an aspect of modeling with mathematics (mp4). This product is based on the im k 12 mathtm by illustrative mathematics® and offered under a cc by 4.0 license. unit title: transformations of functionslesso.
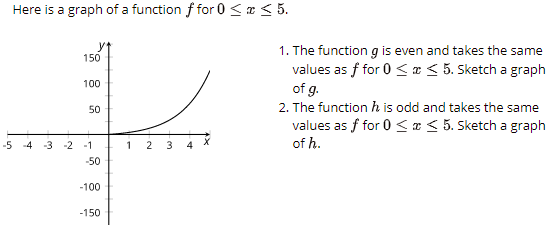
Illustrative Math Algebra 2 Unit 5 Lesson 6 Teerani Description: <p>a graph of function f on a coordinate plane. x axis from negative 4 to 4, by 1’s. y axis from negative 4 to 4, by 1’s. from left to right, the function begins in the second quadrant, moves downward and to the right, crossing the x axis at approximately negative 4, continues downward reaching minimum at negative 3 point 4 comma negative 3 point 0. The point a is at the 0 radian position, b is rotated \frac{7\pi}{12} radians from a, and c is rotated \frac{5\pi}{4} radians from a. for each point a , b , and c , find how high the position on the ferris wheel is off the ground. Problem 5. write an expression for two of the graphs in terms of . <p>graph of function f, a, b, c, and d. x axis from negative 6 to 8, by 2’s. y axis from negative 6 to 8, by 2’s. from left to right, function f starts at the origin, moves upward and to the right to about 2 comma 4, downward to about 4 comma 0, and ends around 5 comma 1. The illustrative mathematics name and logo are not subject to the creative commons license and may not be used without the prior and express written consent of illustrative mathematics. these materials include public domain images or openly licensed images that are copyrighted by their respective owners.
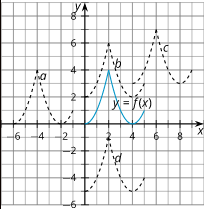
Illustrative Math Algebra 2 Unit 5 Lesson 6 Teerani Problem 5. write an expression for two of the graphs in terms of . <p>graph of function f, a, b, c, and d. x axis from negative 6 to 8, by 2’s. y axis from negative 6 to 8, by 2’s. from left to right, function f starts at the origin, moves upward and to the right to about 2 comma 4, downward to about 4 comma 0, and ends around 5 comma 1. The illustrative mathematics name and logo are not subject to the creative commons license and may not be used without the prior and express written consent of illustrative mathematics. these materials include public domain images or openly licensed images that are copyrighted by their respective owners. Students are given opportunity to make use of structure as they complete the graphs of an even function, an odd function, and a function that is neither (mp7). they also learn to use an input of \text x when determining if a function is even, odd, or neither from the structure of its equation. technology isn't required for this lesson, but. An open top box is formed by cutting squares out of a 5 inch by 7 inch piece of paper and then folding up the sides. the volume v(x) in cubic inches of this type of open top box is a function of the side length x in inches of the square cutouts and can be given by v(x)=(7 2x)(5 2x)(x).
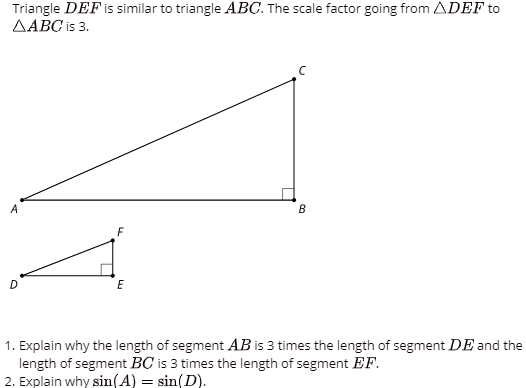
Illustrative Math Algebra 2 Unit 6 Lesson 6 Teerani Students are given opportunity to make use of structure as they complete the graphs of an even function, an odd function, and a function that is neither (mp7). they also learn to use an input of \text x when determining if a function is even, odd, or neither from the structure of its equation. technology isn't required for this lesson, but. An open top box is formed by cutting squares out of a 5 inch by 7 inch piece of paper and then folding up the sides. the volume v(x) in cubic inches of this type of open top box is a function of the side length x in inches of the square cutouts and can be given by v(x)=(7 2x)(5 2x)(x).
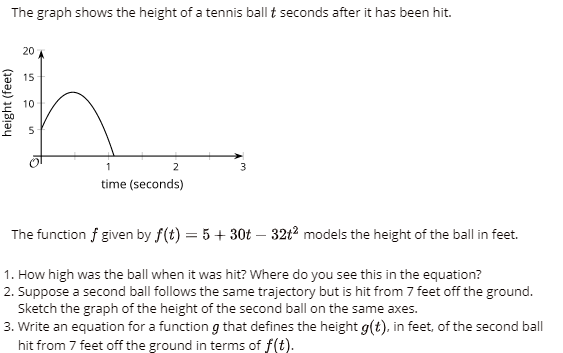
Illustrative Math Algebra 2 Unit 5 Lesson 2 Teerani

Comments are closed.