How To Solve Linear And Nonlinear Systems Of Equations Ft The Math

How To Solve Linear And Nonlinear Equations Definition 11.6.1. a system of nonlinear equations is a system where at least one of the equations is not linear. just as with systems of linear equations, a solution of a nonlinear system is an ordered pair that makes both equations true. in a nonlinear system, there may be more than one solution. we will see this as we solve a system of. This means the system of equations has two valid answers. this is an example of a nonlinear system of equations: y=x^ {2} 4 y = x2 − 4 (quadratic) y=x 2 y = x 2 (linear) these points of intersection are the solutions to the system of equations. they are ordered pairs that satisfy all equations in the system.

How To Solve Linear And Nonlinear Systems Of Equations Ft The Math Here is the solution: step 4: here is the graph of the line intersecting the circle at (2, – 3) substitute the expression of from the top equation to the of the bottom equation. apply the distributive property, then move everything to the left. factor out the trinomial, then set each factor equal to zero to solve for. Solve a system of nonlinear equations by substitution. step 1. identify the graph of each equation. sketch the possible options for intersection. step 2. solve one of the equations for either variable. step 3. substitute the expression from step 2 into the other equation. step 4. See example 7.4.1. there are three possible types of solutions to a system of equations representing a circle and a line: (1) no solution, the line does not intersect the circle; (2) one solution, the line is tangent to the parabola; (3) two solutions, the line intersects the circle in two points. see example 7.4.2. The solutions are the same. linear systems have exactly zero, one, or infinitely many solutions. by contrast, nonlinear systems can have any number of solutions. the function f(x) = x2. 4 has two roots: x = 2 and x = 2. unlike linear systems, there is no grand solvability theorem for nonlinear systems.

110 Solving Systems Of Non Linear Equations Using Elimination Youtube See example 7.4.1. there are three possible types of solutions to a system of equations representing a circle and a line: (1) no solution, the line does not intersect the circle; (2) one solution, the line is tangent to the parabola; (3) two solutions, the line intersects the circle in two points. see example 7.4.2. The solutions are the same. linear systems have exactly zero, one, or infinitely many solutions. by contrast, nonlinear systems can have any number of solutions. the function f(x) = x2. 4 has two roots: x = 2 and x = 2. unlike linear systems, there is no grand solvability theorem for nonlinear systems. Solve a system of nonlinear equations by substitution. step 1. identify the graph of each equation. sketch the possible options for intersection. step 2. solve one of the equations for either variable. step 3. substitute the expression from step 2 into the other equation. step 4. Systems of equations: trolls, tolls (2 of 2) testing a solution to a system of equations. systems of equations with graphing: y=7 5x 5 & y=3 5x 1. systems of equations with graphing: exact & approximate solutions. setting up a system of equations from context example (pet weights).
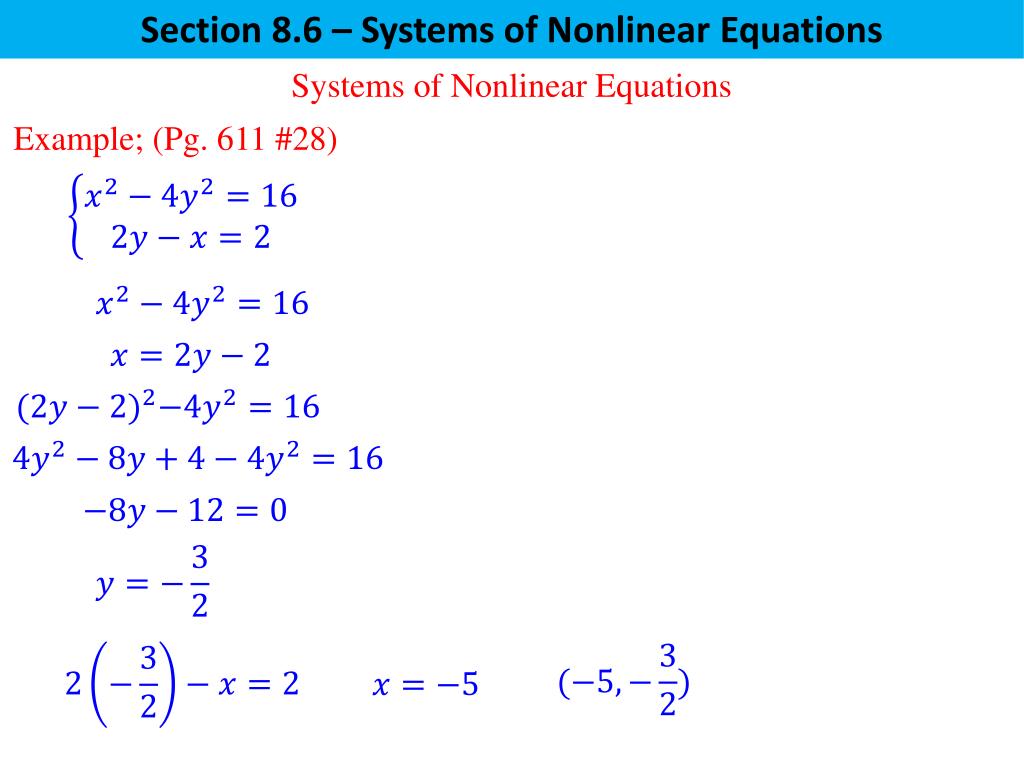
How To Solve Linear And Nonlinear Equations Solve a system of nonlinear equations by substitution. step 1. identify the graph of each equation. sketch the possible options for intersection. step 2. solve one of the equations for either variable. step 3. substitute the expression from step 2 into the other equation. step 4. Systems of equations: trolls, tolls (2 of 2) testing a solution to a system of equations. systems of equations with graphing: y=7 5x 5 & y=3 5x 1. systems of equations with graphing: exact & approximate solutions. setting up a system of equations from context example (pet weights).

Comments are closed.