Higher Order Ordinary Differential Equations

Higher Order Differential Equations Part 6 Youtube The general linear differential equation can be written as. l(y) = ∂ny ∂t p1(t)∂n − 1y ∂t p1 − n(t)∂y ∂t pn(t)y = g(t). the good news is that all the results from second order linear differential equation can be extended to higher order linear differential equations. we list without proof the results. In the spirit of the second order solution, and for the same reasons, we have the solutions. erx, xerx, x2erx, …, xk − 1erx. we take a linear combination of these solutions to find the general solution. example 2.3.4. solve. y (4) − 3y ‴ 3y ″ − y ′ = 0. solution. we note that the characteristic equation is.

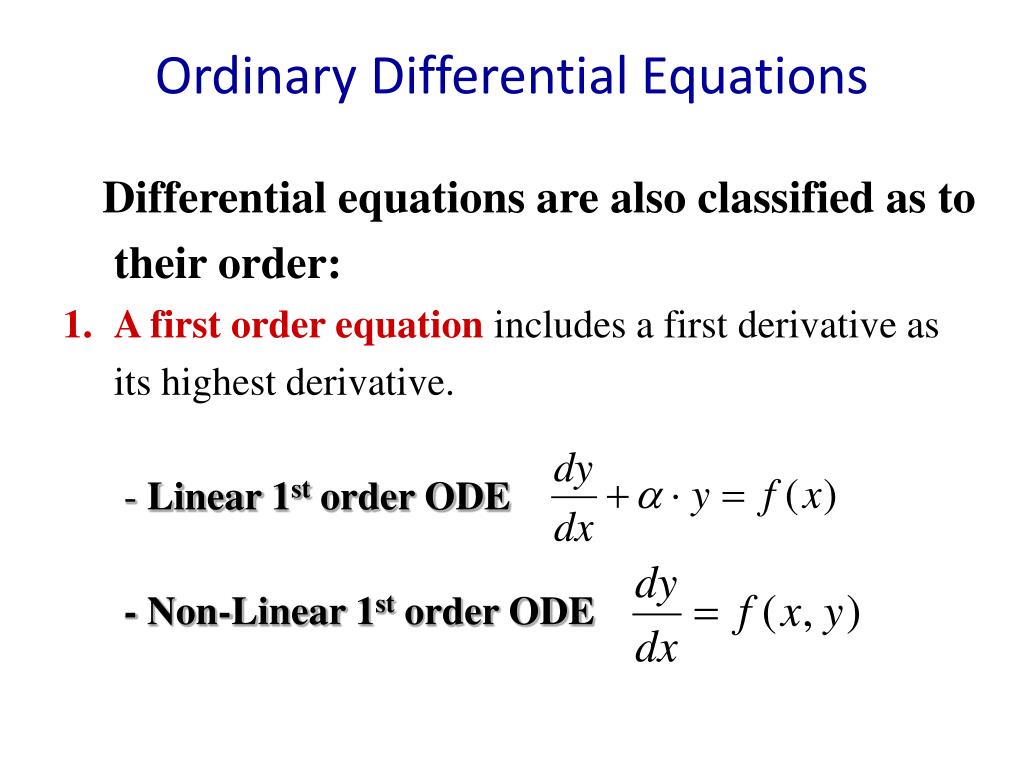
Ppt Part 7 Ordinary Differential Equations Odes Powerpoint Higher order linear ordinary differential equations i: introduction and homogeneous equations david levermore department of mathematics university of maryland 21 march 2011 because the presentation of this material in lecture will differ from that in the book, i felt that notes that closely follow the lecture presentation might be appreciated. 1. This chapter will actually contain more than most text books tend to have when they discuss higher order differential equations. we will definitely cover the same material that most text books do here. however, in all the previous chapters all of our examples were 2 nd order differential equations or 2×2 2 × 2 systems of differential equations. Higher order linear di erential equations math 240 linear de linear di erential operators familiar stu example homogeneous equations introduction we now turn our attention to solving linear di erential equations of order n. the general form of such an equation is a 0(x)y(n) a 1(x)y(n 1) a n(x)y0 a (x)y = f(x); where a 0;a 1;:::;a n; and f. Outline 1 introduction: secondorderlinearequations generaltheory equationswithconstantcoefficients 2 generalsolutionsoflinearequations 3.

4 Ways To Solve Differential Equations Wikihow Higher order linear di erential equations math 240 linear de linear di erential operators familiar stu example homogeneous equations introduction we now turn our attention to solving linear di erential equations of order n. the general form of such an equation is a 0(x)y(n) a 1(x)y(n 1) a n(x)y0 a (x)y = f(x); where a 0;a 1;:::;a n; and f. Outline 1 introduction: secondorderlinearequations generaltheory equationswithconstantcoefficients 2 generalsolutionsoflinearequations 3. Higher order differential equations 1. higher order equations consider the di erential equation (1) y(n)(x) = f(x;y(x);y0(x);:::;y(n 1)(x)): 1.1. the existence and uniqueness theorem suppose x 0 is a given \initial point" x = x 0, and suppose a 0, a 1, , a n 1 are given constants. then there is exactly one solution to the di erential. 8.4 higher order linear ordinary differential equations . the nth order ordinary differential equation a y r x dx dy a dx d y a dx d y a dx d y a dx d y n nn n n n 2 1 2 2 2 2 1 2 1 1 can be solved as follows. form the auxiliary equation . find all n values for . form the complementary function yc, which will be a linear combination of 1 2x x , , ,.

Higher Order Ordinary Differential Equations Lecture 9 Youtube Higher order differential equations 1. higher order equations consider the di erential equation (1) y(n)(x) = f(x;y(x);y0(x);:::;y(n 1)(x)): 1.1. the existence and uniqueness theorem suppose x 0 is a given \initial point" x = x 0, and suppose a 0, a 1, , a n 1 are given constants. then there is exactly one solution to the di erential. 8.4 higher order linear ordinary differential equations . the nth order ordinary differential equation a y r x dx dy a dx d y a dx d y a dx d y a dx d y n nn n n n 2 1 2 2 2 2 1 2 1 1 can be solved as follows. form the auxiliary equation . find all n values for . form the complementary function yc, which will be a linear combination of 1 2x x , , ,.