High Order Ordinary Differential Equations With More Derivatives From

By gudangdomain On Nov 8, 2024 Last updated

High Order Ordinary Differential Equations With More Derivatives From Here we show how to derive higher order differential equation systems, with higher order derivatives, from f=ma by chaining together simple systems. our exa. The general linear differential equation can be written as. l(y) = ∂ny ∂t p1(t)∂n − 1y ∂t p1 − n(t)∂y ∂t pn(t)y = g(t). the good news is that all the results from second order linear differential equation can be extended to higher order linear differential equations. we list without proof the results.

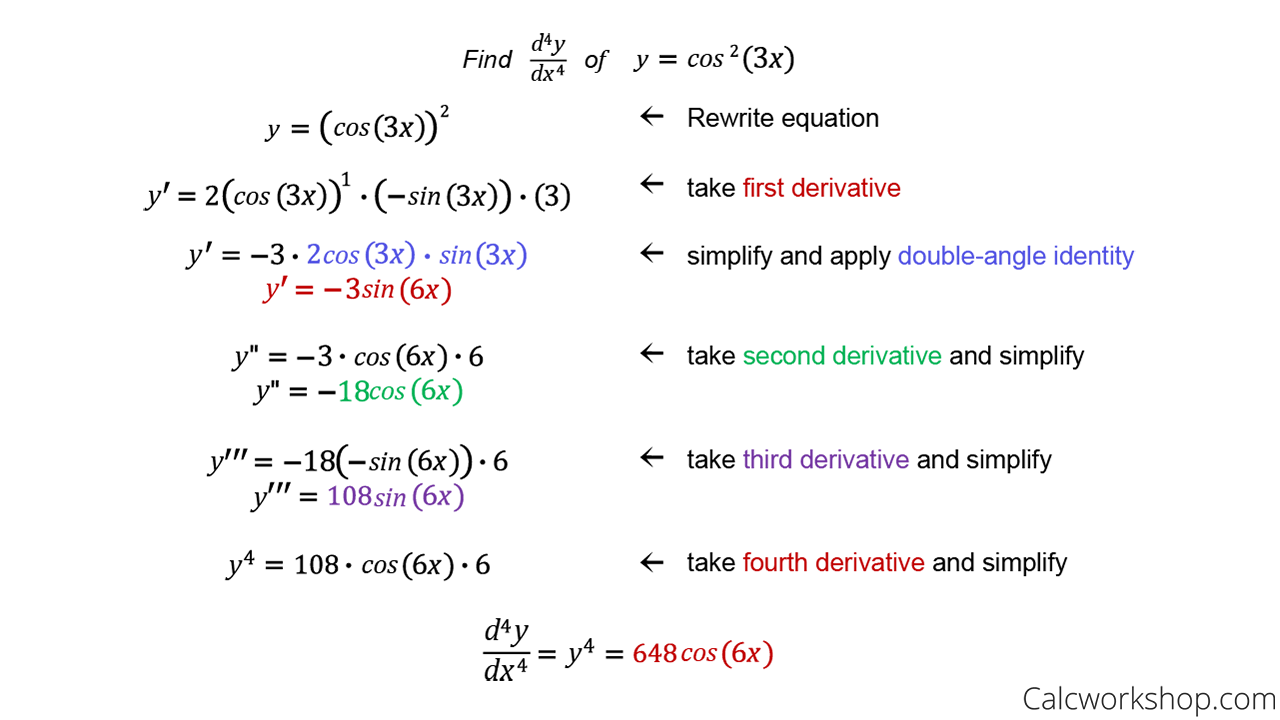
Higher Order Derivatives This chapter will actually contain more than most text books tend to have when they discuss higher order differential equations. we will definitely cover the same material that most text books do here. however, in all the previous chapters all of our examples were 2 nd order differential equations or 2×2 2 × 2 systems of differential equations. Higher order differential equations 1. higher order equations consider the di erential equation (1) y(n)(x) = f(x;y(x);y0(x);:::;y(n 1)(x)): 1.1. the existence and uniqueness theorem suppose x 0 is a given \initial point" x = x 0, and suppose a 0, a 1, , a n 1 are given constants. then there is exactly one solution to the di erential. Moreover, this solution has at least n continuous derivatives over (tl,tr). if the functions a1,a2,··· ,an, and f all have k continuous derivatives over (tl,tr) then this solution has at least k n continuous derivatives over (tl,tr). remark. for ﬁrst order linear equations (n = 1) this theorem was essentially proved. Homogeneous linear equations: pg. 3 reduction of order: pg. 4 method of undetermined coefficients: pg. 6 variation of parameters: pg. 9 . using wronskians to verify independent solutions. because higher order differential equations contain more than one solution, methods are required to verify that these solutions are unique and independent.

Ppt Part 7 Ordinary Differential Equations Odes Powerpoint Moreover, this solution has at least n continuous derivatives over (tl,tr). if the functions a1,a2,··· ,an, and f all have k continuous derivatives over (tl,tr) then this solution has at least k n continuous derivatives over (tl,tr). remark. for ﬁrst order linear equations (n = 1) this theorem was essentially proved. Homogeneous linear equations: pg. 3 reduction of order: pg. 4 method of undetermined coefficients: pg. 6 variation of parameters: pg. 9 . using wronskians to verify independent solutions. because higher order differential equations contain more than one solution, methods are required to verify that these solutions are unique and independent. Derivative operator. higher order derivative operators dk: ck(i) !c0(i) are de ned by composition: dk = d dk 1; so that dk(f) = dkf dxk: a linear di erential operator of order n is a linear combination of derivative operators of order up to n, l = dn a 1dn 1 a n 1d a n; de ned by ly = y(n) a 1y (n 1) a n 1y 0 a ny; where the a i are. We learn how to solve linear higher order differential equations. 3.1.1 initial value and boundary value problems initial value problem in section 1.2 we defined an initial value problem for a general nth order differential equation. for a linear differential equation, an nth order initial value problem is solve: a n1x2 d ny dx 1 a n211x2 d 21y.

Higher Order Ordinary Differential Equations Derivative operator. higher order derivative operators dk: ck(i) !c0(i) are de ned by composition: dk = d dk 1; so that dk(f) = dkf dxk: a linear di erential operator of order n is a linear combination of derivative operators of order up to n, l = dn a 1dn 1 a n 1d a n; de ned by ly = y(n) a 1y (n 1) a n 1y 0 a ny; where the a i are. We learn how to solve linear higher order differential equations. 3.1.1 initial value and boundary value problems initial value problem in section 1.2 we defined an initial value problem for a general nth order differential equation. for a linear differential equation, an nth order initial value problem is solve: a n1x2 d ny dx 1 a n211x2 d 21y.

4 Ways To Solve Differential Equations Wikihow

Higher Order Derivatives Machinelearningmastery

We were solutely delighted to have you here, ready to embark on a journey into the captivating world of High Order Ordinary Differential Equations With More Derivatives From. Whether you were a dedicated High Order Ordinary Differential Equations With More Derivatives From aficionado or someone taking their first steps into this exciting realm, we have crafted a space that is just for you.

High-Order Ordinary Differential Equations with More Derivatives (from Physics)

High-Order Ordinary Differential Equations with More Derivatives (from Physics) Introduction of Higher Order Differential Equations | Lecture 1 Higher Order Constant Coefficient Differential Equations: y'''+y'=0 and y''''-3y'''+3y''-y'=0 Solving General High-Order, Linear Ordinary Differential Equations (ODEs) DiffEq 4 Higher Order Linear ODEs Undetermined Coefficients: Solving non-homogeneous ODEs Chapter0805: Higher Order Ordinary Differential Equations: Background SOLVING FIRST ORDER LINEAR DIFFERENTIAL EQUATIONS| EPISODE-5 | @alphamathematicalinstitute Differential equations, a tourist's guide | DE1 Chapter 08.05: Lesson: Higher Order Ordinary Differential Equations: Background Higher Order Differential Equations - Ordinary Differential Equations | Lecture 19 This is why you're learning differential equations Chapter0805: Higher Order Ordinary Differential Equations: Example Using Euler's Method Differential Equations - 4.1 Higher Order Linear ODEs (Lecture Part 2 of 2) Higher order ordinary differential equations; Complimentary function Converting a Higher Order ODE Into a System of First Order ODEs Ordinary Differential Equations - Intro

Conclusion

After a comprehensive review, one can conclude that this particular write-up delivers insightful data regarding High Order Ordinary Differential Equations With More Derivatives From. Throughout the content, the author shows remarkable understanding pertaining to the theme. Notably, the segment on this element stands out as extremely valuable. Additionally, the write-up is noteworthy in simplifying complex concepts in an clear manner. In addition, the journalist imparts case studies that make the information more relatable. One more trait that makes this manuscript distinctive is the comprehensive analysis of varied aspects related to High Order Ordinary Differential Equations With More Derivatives From. The commentators systematic manner makes certain that observers achieve a thorough comprehension of the subject matter. Thanks for your attention to the content. If you would like to know more, dont think twice to get in touch employing comments or email. I am eager to your reactions. In final remarks, as you continue exploring, provided here are various akin publications that you may find valuable:Wishing you enjoyable reading!