Golden Ratio For Art Beginners Arthistory

Golden Ratio For Art Beginners Arthistory Youtube 1 to 1.618if curious for more of my art, where i show my #wip daily:instagram instagram ours.for.all i'm a textile artist with a primary focu. The golden ratio is a mathematical proportion defined by the ratio of 1 to 1.618, represented by the greek letter phi. it is an irrational number, meaning its value cannot be expressed exactly as a simple fraction. the golden ratio is also called the golden section, golden mean, divine proportion, extreme and mean ratio, and the divine proportion.

The Golden Ratio In Art Is One Of The Coolest Things You Ll Ever To use the golden rectangle for your art, just follow these simple steps: make a 1×1 square. this will be the smallest square on your canvas. create another equal size square to the right of the original square. create a 2×2 square under your original two 1×1 squares. Step 1 – construct a simple square. step 2 – draw a line down the middle of the square. step 3 – grab your compass and place one point at the intersection at the bottom middle and draw down from the edge of top right corner, as shown below. step 4 – complete the golden rectangle. The golden ratio, also known as “phi” and more popularly known as the fibonacci sequence, is an irregular equation. a ratio of 1 to 1.618 is what is referred to as an irrational number, similar to that of the famous einstein equation “pi”. for the sake of beautiful aesthetics, this ratio can be a helpful tool for capturing balance and. The golden ratio is a mathematical ratio that is represented by the number 1.618. the golden ratio also has many other names, such as the golden number and golden section, among others, and is also known as the greek letter phi. it is used in art to create the best placement of subject and form.
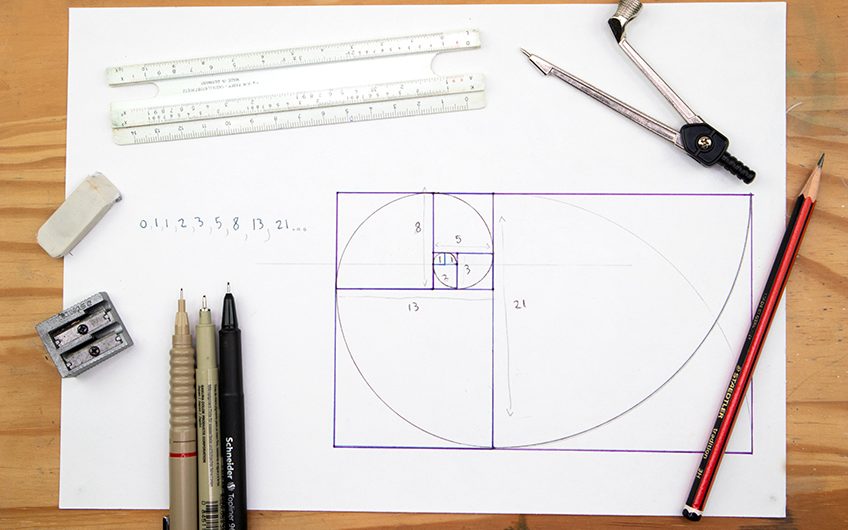
Golden Ratio In Art Learn How To Use The Golden Ratio In Art The golden ratio, also known as “phi” and more popularly known as the fibonacci sequence, is an irregular equation. a ratio of 1 to 1.618 is what is referred to as an irrational number, similar to that of the famous einstein equation “pi”. for the sake of beautiful aesthetics, this ratio can be a helpful tool for capturing balance and. The golden ratio is a mathematical ratio that is represented by the number 1.618. the golden ratio also has many other names, such as the golden number and golden section, among others, and is also known as the greek letter phi. it is used in art to create the best placement of subject and form. Today we explore how to use the golden ratio to make a compostion balance in a painting.the golden ratio for art beginners.information about jamie's classes . Mar 22, 2023 • by rosie lesso, ma contemporary art theory, ba fine art. the golden ratio, also known as the divine proportion, is a mathematical ratio of 1:1.618, or phi, with a decimal that stretches to infinity, closely linked to the fibonacci sequence. sometimes it is also referred to as the golden section, the golden mean, the golden.

Golden Ratio In Art Learn How To Use The Golden Ratio In Art Today we explore how to use the golden ratio to make a compostion balance in a painting.the golden ratio for art beginners.information about jamie's classes . Mar 22, 2023 • by rosie lesso, ma contemporary art theory, ba fine art. the golden ratio, also known as the divine proportion, is a mathematical ratio of 1:1.618, or phi, with a decimal that stretches to infinity, closely linked to the fibonacci sequence. sometimes it is also referred to as the golden section, the golden mean, the golden.

Comments are closed.