Geometry Circles Using Equations Of Circles Hard
Using Equations Of Circles Worksheets A circle is defined as the set (locus) of points in a plane equidistant from a given point (the center of the circle.) equations of circles: circle with center at origin (0,0) x2 y2 = r2. with the center at (0,0) and the radius r. in the example at the right, x2 y2 = 9. the center is (0,0) and the radius = 3. Circle on a graph. let us put a circle of radius 5 on a graph: now let's work out exactly where all the points are. we make a right angled triangle: and then use pythagoras: x 2 y 2 = 5 2. there are an infinite number of those points, here are some examples:.

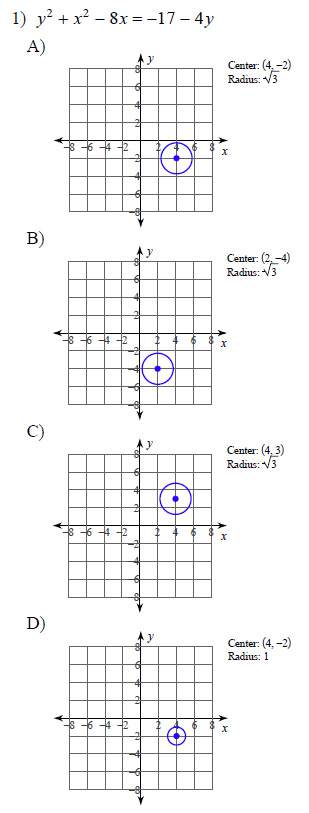
Geometry Circles Writing Equations Of Circles Hard Youtube Standard equation of a circle if the center of a circle is not at the origin, you can use the distance formula to write an equation of the circle. for example, the circle shown at the right has center (3, 5) and radius 4. let (x, y) represent any point on the circle. use the distance formula to find the lengths of the legs. High school geometry answered question. brendon watson 2023 03 16. to draw a pie chart, you need to represent each part of the data as a proportion of 360, because there are 360 degrees in a circle. for example, if 55 out of 270 vehicles are vans, we will represent this on the circle as a segment with an angle of: 55 27 × 360 = 73 degrees. Use the information provided to write the equation of each circle. 9) center: (13 , −13) radius: 4 (x − 13)2 (y 13)2 = 16 10) center: (−13 , −16) point on circle: (−10 , −16) (x 13)2 (y 16)2 = 9 11) ends of a diameter: (18 , −13) and (4, −3) (x − 11)2 (y 8)2 = 74 12) center: (10 , −14) tangent to x = 13 (x −. 11. the equation x2 y2 6 x 4 y = d. describes a circle. a) determine the y coordinate of the center of the circle. b) the radius of the circle is 6 units. what is the value of "d" in the given equation. solution. 12. given circle: x2 9 x y2 = 4.75.

Circles Formula Sheet Geometry Pdf Use the information provided to write the equation of each circle. 9) center: (13 , −13) radius: 4 (x − 13)2 (y 13)2 = 16 10) center: (−13 , −16) point on circle: (−10 , −16) (x 13)2 (y 16)2 = 9 11) ends of a diameter: (18 , −13) and (4, −3) (x − 11)2 (y 8)2 = 74 12) center: (10 , −14) tangent to x = 13 (x −. 11. the equation x2 y2 6 x 4 y = d. describes a circle. a) determine the y coordinate of the center of the circle. b) the radius of the circle is 6 units. what is the value of "d" in the given equation. solution. 12. given circle: x2 9 x y2 = 4.75. The equation indicates how a circle would need to shift to return to the origin. therefore, the signs in the equation are opposite the signs in the coordinate. for example, an origin of (3, –4) would need to shift left 3 and up 4, which is (x 3)^2 (y 4)^2=r^2. confusing equation of a circle with quadratic equations. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you. revision notes on 3.2.1 equation of a circle for the edexcel a level maths: pure syllabus, written by the maths experts at save my exams.

Geometry Equations Of Circles Youtube The equation indicates how a circle would need to shift to return to the origin. therefore, the signs in the equation are opposite the signs in the coordinate. for example, an origin of (3, –4) would need to shift left 3 and up 4, which is (x 3)^2 (y 4)^2=r^2. confusing equation of a circle with quadratic equations. Having conducted and published postgraduate research into the mathematical theory behind quantum computing, he is more than confident in dealing with mathematics at any level the exam boards might throw at you. revision notes on 3.2.1 equation of a circle for the edexcel a level maths: pure syllabus, written by the maths experts at save my exams.

Comments are closed.