Fundamental Theorems Of Mathematics
Fundamental Theorems Of Mathematics In mathematics, a fundamental theorem is a theorem which is considered to be central and conceptually important for some topic. for example, the fundamental theorem of calculus gives the relationship between differential calculus and integral calculus . [ 1 ]. Second fundamental theorem of calculus. when we have a continuous function f (x) on an interval [a, b], and its indefinite integral is f (x), then: b. ∫. a. f (x) dx = f (b) − f (a) in other words the definite integral of f (x) from a to b equals the difference in the values of f (x) at b and a. this makes calculating a definite integral.
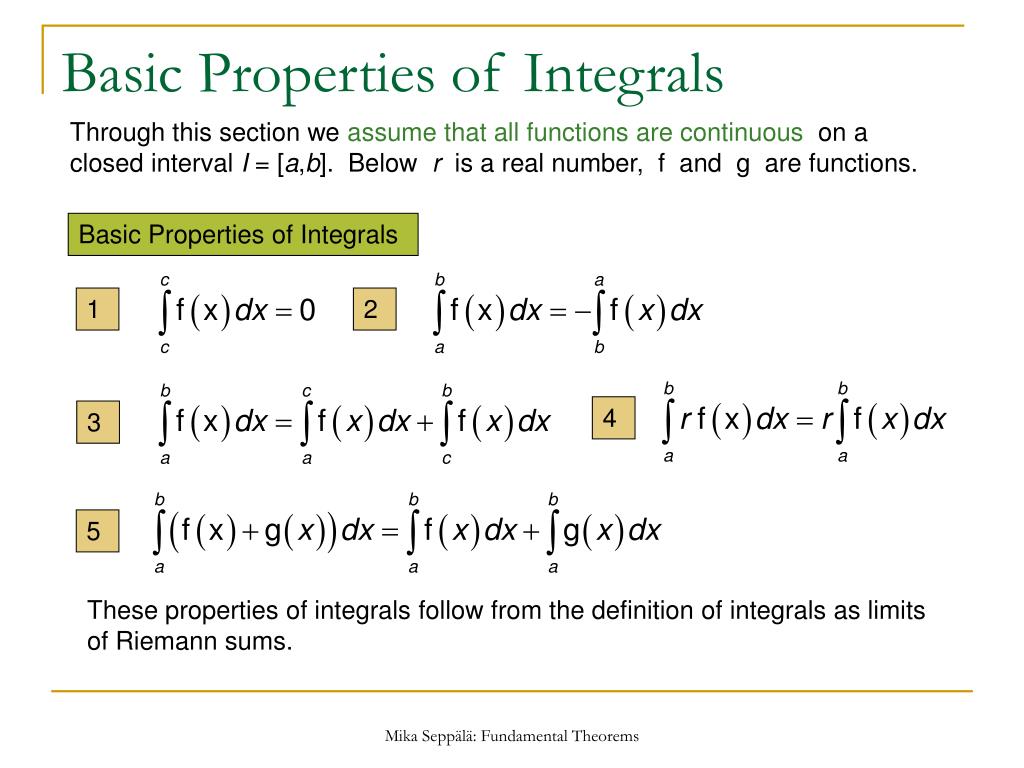
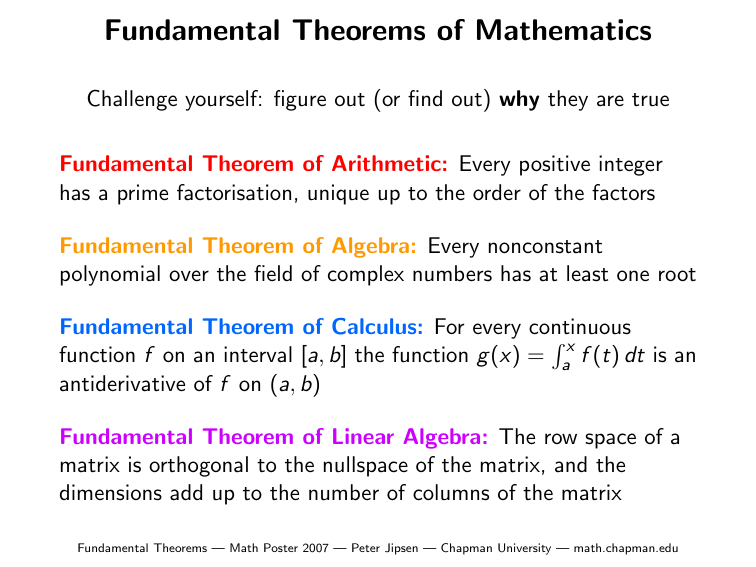
Ppt Fundamental Theorems Of Calculus Powerpoint Presentation Free Fundamental theorem of algebra (complex analysis) fundamental theorem of arbitrage free pricing (financial mathematics) fundamental theorem of arithmetic (number theory) fundamental theorem of calculus ; fundamental theorem on homomorphisms (abstract algebra) fundamental theorems of welfare economics ; furry's theorem (quantum field theory). Fundamental theorem of linear algebra: the row space of a matrix is orthogonal to the nullspace of the matrix, and the dimensions add up to the number of columns of the matrix fundamental theorems — math poster 2007 — peter jipsen — chapman university — math.chapman.edu. The fundamental theorem(s) of multivariable calculus: (note: technically, all these theorems are a special case of an n dimensional stokes' theorem.) green's theorem: let c be a simple, closed path, orientated counterclockwise, that encloses q. then stokes' theorem: if the surface s is bounded by the curve c, then. The fundamental theorem of calculus, part 1 shows the relationship between the derivative and the integral. the fundamental theorem of calculus, part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand. the total area under a curve can be found using this formula.
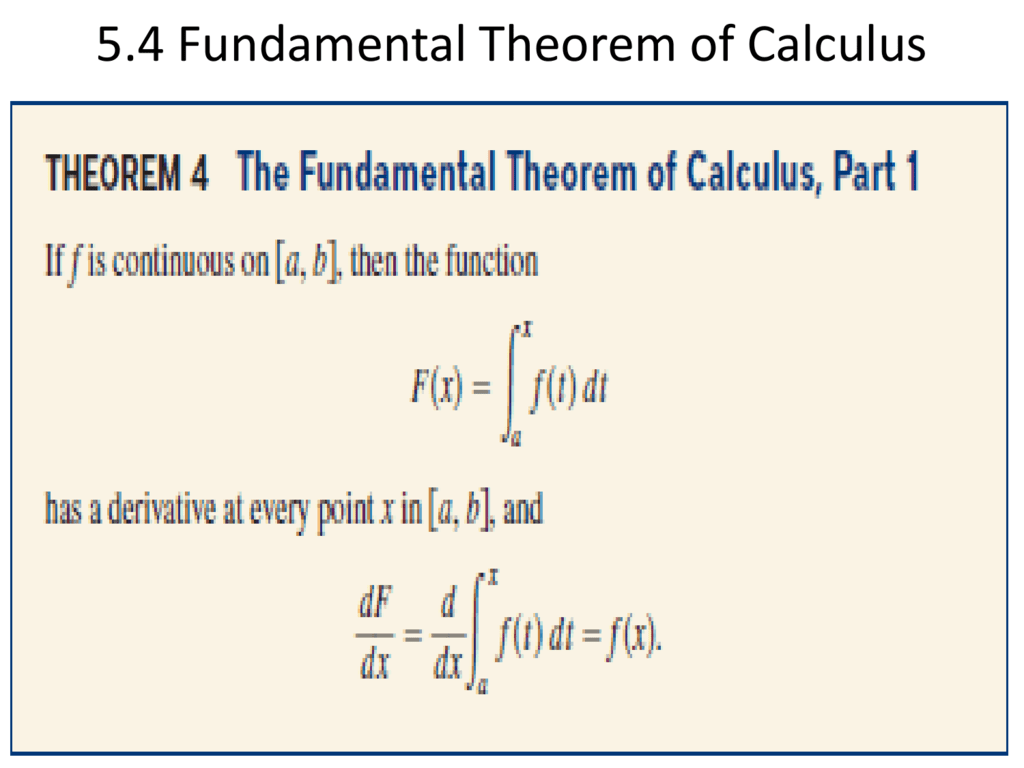
5 4 Fundamental Theorem Of Calculus The fundamental theorem(s) of multivariable calculus: (note: technically, all these theorems are a special case of an n dimensional stokes' theorem.) green's theorem: let c be a simple, closed path, orientated counterclockwise, that encloses q. then stokes' theorem: if the surface s is bounded by the curve c, then. The fundamental theorem of calculus, part 1 shows the relationship between the derivative and the integral. the fundamental theorem of calculus, part 2 is a formula for evaluating a definite integral in terms of an antiderivative of its integrand. the total area under a curve can be found using this formula. A fundamental theorem is a statement or proposition so named because it has consequences for the subject matter that are difficult to overestimate. put another way, a fundamental theorem lies at the very heart of the subject. a theorem is called fundamental if other theorems can follow from it by accepting it as a foundation to build upon other. Some fundamental theorems in mathematics oliver knill abstract. an expository hitchhikers guide to some theorems in mathematics. criteria for the current list of 272 theorems are whether the result can be formulated elegantly, whether it is beautiful or useful and whether it could serve as a guide [6] without leading to panic.

Comments are closed.