Fibonacci Sequence Formula Spiral Properties List Formulas Golden

Fibonacci Sequence Formula Spiral Properties List Formulas Golden The fibonacci sequence has several interesting properties. 1) fibonacci numbers are related to the golden ratio. any fibonacci number can be calculated (approximately) using the golden ratio, f n = (Φ n (1 Φ) n) √5 (which is commonly known as "binet formula"), here φ is the golden ratio and Φ ≈ 1.618034. Named after the italian mathematician leonardo fibonacci, this sequence has a unique set of properties and is found in various natural and man made phenomena. in this article, we will explore the formula, spiral, properties, list, formulas, golden ratio, diagrams, and examples associated with the fibonacci sequence. what is fibonacci sequence?.

Fibonacci Sequence Definition Formula List Examples Diagrams For example, the next term after 21 can be found by adding 13 and 21. therefore, the next term in the sequence is 34. fibonacci sequence formula. the fibonacci sequence of numbers “f n ” is defined using the recursive relation with the seed values f 0 =0 and f 1 =1: f n = f n 1 f n 2. The mathematical ideas the fibonacci sequence leads to, such as the golden ratio, spirals and self similar curves, have long been appreciated for their charm and beauty, but no one can really explain why they are echoed so clearly in the world of art and nature. the story began in pisa, italy in the year 1202. This can be generalized to a formula known as the golden power rule. golden power rule: ϕn = fnϕ fn−1 ϕ n = f n ϕ f n − 1. where fn f n is the nth fibonacci number and ϕ ϕ is the golden ratio. example 10.4.5 10.4. 5: powers of the golden ratio. find the following using the golden power rule: a. and b. Properties of fibonacci sequence. important properties of fibonacci sequence are: we can easily calculate the fibonacci numbers using the binet formula: f n = (Φ n – (1 Φ) n) √5. u sing this formula we can easily calculate the n th term of the fibonacci sequence as, for finding fourth term of fibonacci sequence,.
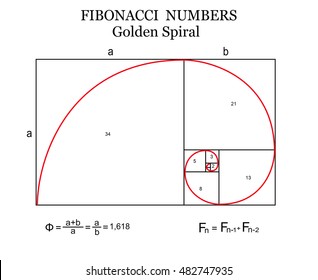
Fibonacci Spiral Known Golden Spiral Basic Stock Vector Royalty Free This can be generalized to a formula known as the golden power rule. golden power rule: ϕn = fnϕ fn−1 ϕ n = f n ϕ f n − 1. where fn f n is the nth fibonacci number and ϕ ϕ is the golden ratio. example 10.4.5 10.4. 5: powers of the golden ratio. find the following using the golden power rule: a. and b. Properties of fibonacci sequence. important properties of fibonacci sequence are: we can easily calculate the fibonacci numbers using the binet formula: f n = (Φ n – (1 Φ) n) √5. u sing this formula we can easily calculate the n th term of the fibonacci sequence as, for finding fourth term of fibonacci sequence,. The fibonacci sequence has some important properties, which we will discuss below. fibonacci sequence and golden ratio. two successive fibonacci numbers give the value ${\phi =\dfrac{1 \sqrt{5}}{2}}$ or, 1.618…, which is known as the golden ratio, also known as phi (an irrational number). for the given spiral, the golden ratio follows the. Makes a spiral. when we make squares with those widths, we get a nice spiral: do you see how the squares fit neatly together? for example 5 and 8 make 13, 8 and 13 make 21, and so on. this spiral is found in nature! see: nature, the golden ratio, and fibonacci. the rule. the fibonacci sequence can be written as a "rule" (see sequences and series).

Fibonacci Spiral The Fibonacci Golden Spiral The fibonacci sequence has some important properties, which we will discuss below. fibonacci sequence and golden ratio. two successive fibonacci numbers give the value ${\phi =\dfrac{1 \sqrt{5}}{2}}$ or, 1.618…, which is known as the golden ratio, also known as phi (an irrational number). for the given spiral, the golden ratio follows the. Makes a spiral. when we make squares with those widths, we get a nice spiral: do you see how the squares fit neatly together? for example 5 and 8 make 13, 8 and 13 make 21, and so on. this spiral is found in nature! see: nature, the golden ratio, and fibonacci. the rule. the fibonacci sequence can be written as a "rule" (see sequences and series).

Fibonacci Sequence Golden Spiral Stock Photography Image 29677232

Fibonacci And The Golden Section Inner Workings Resources

Comments are closed.