Fibonacci Sequence Definition Formula List Examples Diagrams

Fibonacci Sequence Definition Formula List Examples Diagrams For example, the next term after 21 can be found by adding 13 and 21. therefore, the next term in the sequence is 34. fibonacci sequence formula. the fibonacci sequence of numbers “f n ” is defined using the recursive relation with the seed values f 0 =0 and f 1 =1: f n = f n 1 f n 2. Solved examples. find the sum of the first 15 fibonacci numbers. solution: as we know, the sum of the fibonacci sequence = ∑ i = 0 n f i = f n 2 – f 2. = f n 2 − 1, where f n is the nth fibonacci number, and the sequence starts from f 0. thus, the sum of the first 15 fibonacci numbers = (15 2) th term – 2 nd term.

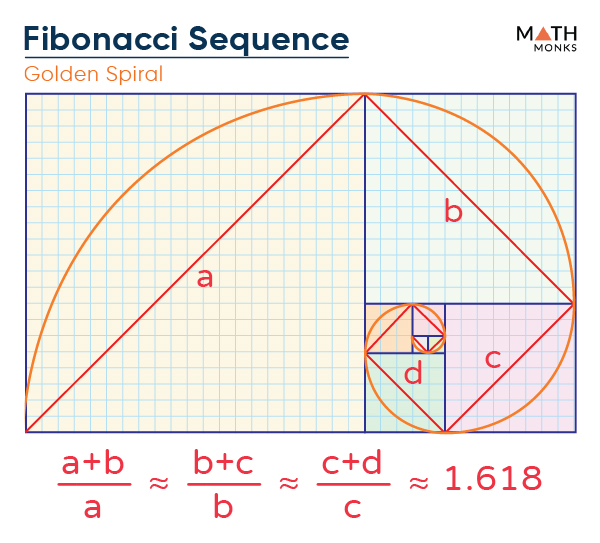
Fibonacci Sequence Definition Formula List Examples Diagrams Fibonacci sequence formula. the fibonacci sequence of numbers, say “fn” where the suffix n denotes the order or rank of term, is defined by. initial term: f 0 = 0. first term: f 1 = 1. these two terms together are known as the kick off part. formula for next terms: f n = f n − 1 f n − 2. The fibonacci sequence has several interesting properties. 1) fibonacci numbers are related to the golden ratio. any fibonacci number can be calculated (approximately) using the golden ratio, f n = (Φ n (1 Φ) n) √5 (which is commonly known as "binet formula"), here φ is the golden ratio and Φ ≈ 1.618034. In mathematics, the fibonacci sequence is a sequence in which each number is the sum of the two preceding ones. numbers that are part of the fibonacci sequence are known as fibonacci numbers, commonly denoted fn . many writers begin the sequence with 0 and 1, although some authors start it from 1 and 1 [1][2] and some (as did fibonacci) from 1. Fibonacci sequence, the sequence of numbers 1, 1, 2, 3, 5, 8, 13, 21, …, each of which, after the second, is the sum of the two previous numbers; that is, the n th fibonacci number fn = fn − 1 fn − 2. the sequence was noted by the medieval italian mathematician fibonacci (leonardo pisano) in his liber abaci (1202; “book of the abacus.

Fibonacci Sequence Definition Formula List Examples Diagrams In mathematics, the fibonacci sequence is a sequence in which each number is the sum of the two preceding ones. numbers that are part of the fibonacci sequence are known as fibonacci numbers, commonly denoted fn . many writers begin the sequence with 0 and 1, although some authors start it from 1 and 1 [1][2] and some (as did fibonacci) from 1. Fibonacci sequence, the sequence of numbers 1, 1, 2, 3, 5, 8, 13, 21, …, each of which, after the second, is the sum of the two previous numbers; that is, the n th fibonacci number fn = fn − 1 fn − 2. the sequence was noted by the medieval italian mathematician fibonacci (leonardo pisano) in his liber abaci (1202; “book of the abacus. Fibonacci was not the first to know about the sequence, it was known in india hundreds of years before! about fibonacci the man. his real name was leonardo pisano bogollo, and he lived between 1170 and 1250 in italy. "fibonacci" was his nickname, which roughly means "son of bonacci". The fibonacci sequence is an integer sequence defined by a simple linear recurrence relation. the sequence appears in many settings in mathematics and in other sciences. in particular, the shape of many naturally occurring biological organisms is governed by the fibonacci sequence and its close relative, the golden ratio. the first few terms are.