Exterior Angles Of Triangle Definition Formula Properties
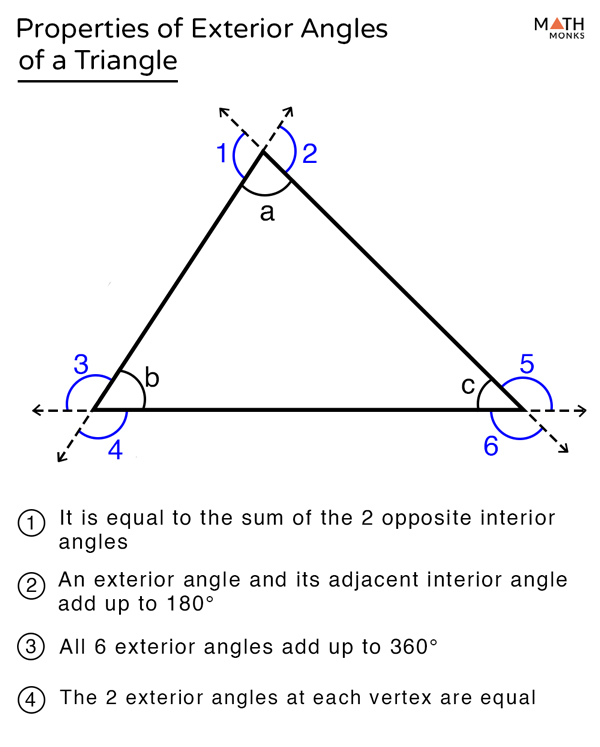
Exterior Angle Of A Triangle Definition Theorem Proof Examples Are the exterior angles of a triangle always obtuse? no, the exterior angles of a triangle may not always be obtuse (more than 90°). however, the sum of all the three exterior angles should always be 360°. for example, if two exterior angles of a triangle are 165° (obtuse) and 141° (obtuse), the third one is 54° (acute). The above statement can be explained using the figure provided as: according to the exterior angle property of a triangle theorem, the sum of measures of ∠abc and ∠cab would be equal to the exterior angle ∠acd. general proof of this theorem is explained below: proof: consider a ∆abc as shown in fig. 2, such that the side bc of ∆abc is.
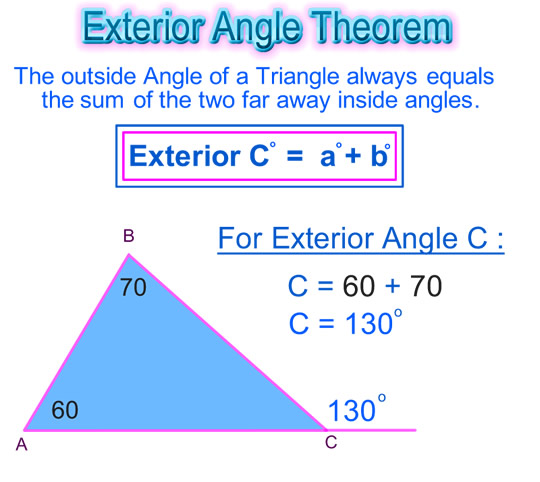
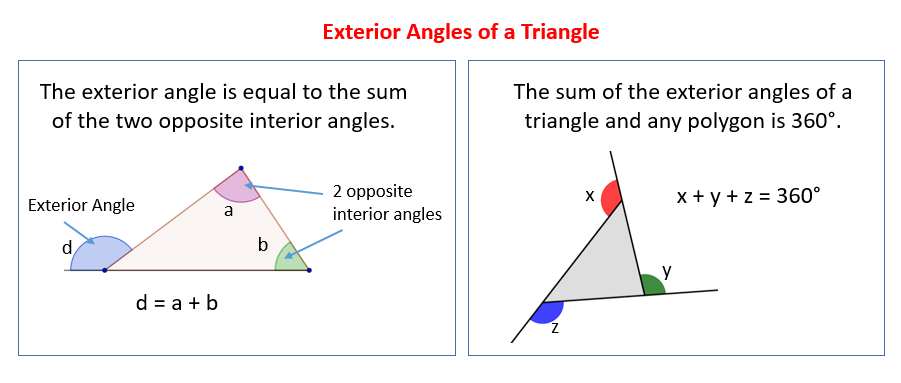
Exterior Angle Of A Triangle Passy S World Of Mathematics Properties. an exterior angle of a triangle is equal to the sum of the opposite interior angles. for more on this see triangle external angle theorem. if the equivalent angle is taken at each vertex, the exterior angles always add to 360° in fact, this is true for any convex polygon, not just triangles. see exterior angles of a polygon. Example 1: find the values of x and y by using the exterior angle theorem of a triangle. solution: ∠x is the exterior angle. ∠x 92 = 180º (linear pair of angles) ∠x = 180 92 = 88º. applying the exterior angle theorem, we get, ∠y 41 = 88. ∠y = 88 41 = 47º. therefore, the values of x and y are 88º and 47º respectively. The exterior angle sum theorem states that the exterior angles of any polygon will always add up to 360 ∘. figure 4.18.3. m∠1 m∠2 m∠3 = 360 ∘. m∠4 m∠5 m∠6 = 360 ∘. the exterior angle theorem states that an exterior angle of a triangle is equal to the sum of its remote interior angles. (remote interior angles are the. An exterior angle of a triangle is equal to the sum of the two opposite interior angles, thus an exterior angle is greater than any of its two opposite interior angles; for example, in Δabc, ∠5 = ∠a ∠b. the sum of an exterior angle and its adjacent interior angle is equal to 180 degrees; for example, ∠5 ∠c = 180°.
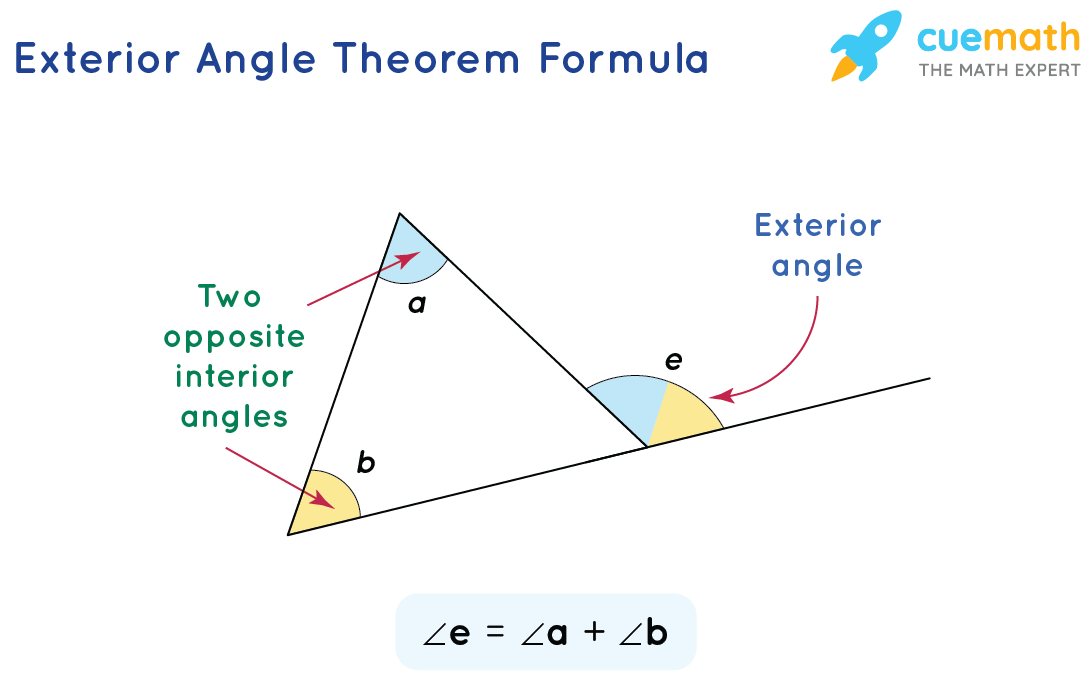
Exterior Angle Formula Concept And Solved Examples The exterior angle sum theorem states that the exterior angles of any polygon will always add up to 360 ∘. figure 4.18.3. m∠1 m∠2 m∠3 = 360 ∘. m∠4 m∠5 m∠6 = 360 ∘. the exterior angle theorem states that an exterior angle of a triangle is equal to the sum of its remote interior angles. (remote interior angles are the. An exterior angle of a triangle is equal to the sum of the two opposite interior angles, thus an exterior angle is greater than any of its two opposite interior angles; for example, in Δabc, ∠5 = ∠a ∠b. the sum of an exterior angle and its adjacent interior angle is equal to 180 degrees; for example, ∠5 ∠c = 180°. Exterior angles of a triangle have three fundamental characteristics: each exterior angle and corresponding interior angle of a triangle make up a linear pair of angles. accordingly, the interior and exterior angles add up to \(180^\circ\). the sum of the two opposing internal angles determines the measure of the exterior angle of a triangle. An exterior angle of a triangle is equal to the sum of the two opposite interior angles. example: find the values of x and y in the following triangle. solution: x 50° = 92° (sum of opposite interior angles = exterior angle) x = 92° – 50° = 42°. y 92° = 180° (interior angle adjacent exterior angle = 180°.) y = 180° – 92° = 88°.
Exterior Angles Of A Triangle Video Lessons Examples Step By Step Exterior angles of a triangle have three fundamental characteristics: each exterior angle and corresponding interior angle of a triangle make up a linear pair of angles. accordingly, the interior and exterior angles add up to \(180^\circ\). the sum of the two opposing internal angles determines the measure of the exterior angle of a triangle. An exterior angle of a triangle is equal to the sum of the two opposite interior angles. example: find the values of x and y in the following triangle. solution: x 50° = 92° (sum of opposite interior angles = exterior angle) x = 92° – 50° = 42°. y 92° = 180° (interior angle adjacent exterior angle = 180°.) y = 180° – 92° = 88°.

Comments are closed.