Exponential Derivatives In Calculus Mth101 Mathemagics By Mehdi

Exponential Derivatives In Calculus Mth101 Mathemagics By Mehdi 🌟 welcome to mathemagics, your go to destination for mastering the enchanting world of mathematics! in this video, mehdi, your dedicated mth101 course lectu. Ln. ⁡. (z) is increasing or decreasing at the following points. z = 1 z = 1. z =5 z = 5. z = 20 z = 20. solution. here is a set of practice problems to accompany the derivatives of exponential and logarithm functions section of the derivatives chapter of the notes for paul dawkins calculus i course at lamar university.

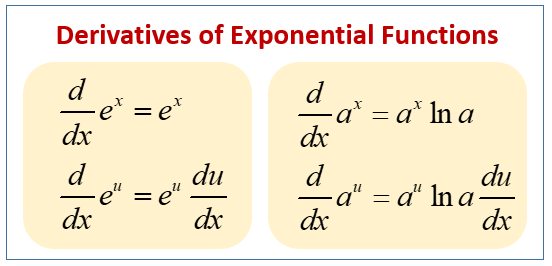
Calculus Exponential Derivatives Examples Solutions Videos This page titled 2.7: derivatives of exponential functions is shared under a cc by nc sa 4.0 license and was authored, remixed, and or curated by joel feldman, andrew rechnitzer and elyse yeager via source content that was edited to the style and standards of the libretexts platform. now that we understand how derivatives interact with products. The function e(x) = ex is called the natural exponential function. its inverse, l(x) = logex = lnx is called the natural logarithmic function. figure 3.33 the graph of e(x) = ex is between y = 2x and y = 3x. for a better estimate of e, we may construct a table of estimates of b ′ (0) for functions of the form b(x) = bx. Derivatives of exponential functions. in order to differentiate the exponential function. f (x) = a^x, f (x) = ax, we cannot use power rule as we require the exponent to be a fixed number and the base to be a variable. instead, we're going to have to start with the definition of the derivative: \begin {aligned} f' (x) &= \lim {h \rightarrow 0. The exponential function f (x) = e x has the property that it is its own derivative. this means that the slope of a tangent line to the curve y = e x at any point is equal to the y coordinate of the point. we can combine the above formula with the chain rule to get. example: differentiate the function y = e sin x.

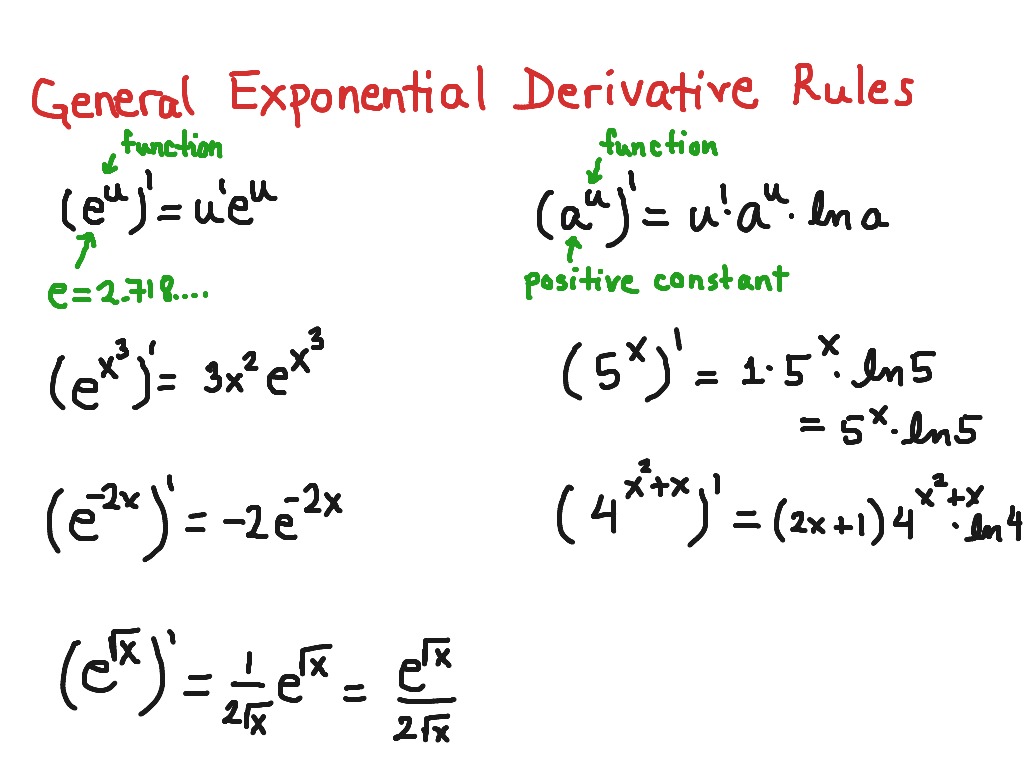
Exponential Derivative Rules Math Showme Derivatives of exponential functions. in order to differentiate the exponential function. f (x) = a^x, f (x) = ax, we cannot use power rule as we require the exponent to be a fixed number and the base to be a variable. instead, we're going to have to start with the definition of the derivative: \begin {aligned} f' (x) &= \lim {h \rightarrow 0. The exponential function f (x) = e x has the property that it is its own derivative. this means that the slope of a tangent line to the curve y = e x at any point is equal to the y coordinate of the point. we can combine the above formula with the chain rule to get. example: differentiate the function y = e sin x. Note that we need to require that x> 0 x> 0 since this is required for the logarithm and so must also be required for its derivative. it can also be shown that, d dx (ln|x|) = 1 x x ≠ 0 d d x (ln | x |) = 1 x x ≠ 0. using this all we need to avoid is x = 0 x = 0. in this case, unlike the exponential function case, we can actually find the. Exponential of a derivative is simply a shift operator, i.e. ea∂f(x) = f(a x) this can be easily verified from a taylor series ea∂ = ∞ ∑ n = 0(a∂)n n! and f(x) = ∞ ∑ n = 0cnxn n! and applying one on another by using ∂mxn = n! (n − m)! xn − m for n ≥ m and zero otherwise. what if, instead of constant a, there is a.