Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume

By gudangdomain On Nov 8, 2024 Last updated

Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume This video provides an example of how to find the dimensions of a right circular cylinder that will minimized production costs.site: mathispower4u. One common application of calculus is calculating the minimum or maximum value of a function. for example, companies often want to minimize production costs or maximize revenue. in manufacturing, it is often desirable to minimize the amount of material used to package a product with a certain volume.

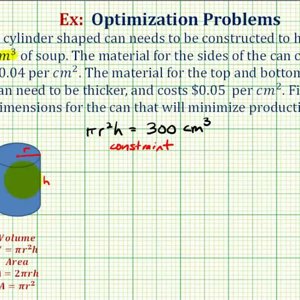
Calculus Videos Page 2 Physics Forums Science Articles Homework The volume of a cylindrical can is given by πr2h, where r is the radius of the base and h is the height. the area of the surface is given by: 2πrh ( area of the side) πr2 ( area of the bottom), there is no top. from the given v, you can express h = v πr2. substitute to the second equation to get s(r) = 2v r πr2. Consider the same open top box, which is to have volume \(216\,\text{in}^3\). suppose the cost of the material for the base is \(20¢ \text{in}^2\) and the cost of the material for the sides is \(30¢ \text{in}^2\) and we are trying to minimize the cost of this box. write the cost as a function of the side lengths of the base. Essentially, you must minimize the surface area of the cylinder. step 1: write the primary equation: the surface area is the area of the two ends (each πr²) plus the area of the side or lateral area. to minimize (primary equation): a = 2πr² 2πrh. step 2: the primary equation contains two independent variables, r and h. It can depend on only one variable. the steps: 1. draw a picture of the physical situation. see the figure. we’ve called the radius of the cylinder r, and its height h. 2. write an equation that relates the quantity you want to optimize in terms of the relevant variables.

Optimization Minimize Material Cost For A Fixed Volume Youtube Essentially, you must minimize the surface area of the cylinder. step 1: write the primary equation: the surface area is the area of the two ends (each πr²) plus the area of the side or lateral area. to minimize (primary equation): a = 2πr² 2πrh. step 2: the primary equation contains two independent variables, r and h. It can depend on only one variable. the steps: 1. draw a picture of the physical situation. see the figure. we’ve called the radius of the cylinder r, and its height h. 2. write an equation that relates the quantity you want to optimize in terms of the relevant variables. Step 1: draw a rectangular box and introduce the variable to represent the length of each side of the square base; let represent the height of the box. let denote the surface area of the open top box. figure 8. we want to minimize the surface area of a square based box with a given volume. Show solution. 30. x2 −y x 2 − y. for the following exercises (31 36), draw the given optimization problem and solve. 31. find the volume of the largest right circular cylinder that fits in a sphere of radius 1. show solution. 32. find the volume of the largest right cone that fits in a sphere of radius 1. 33.

4 6 Optimization Minimize Cost Of Can Youtube Step 1: draw a rectangular box and introduce the variable to represent the length of each side of the square base; let represent the height of the box. let denote the surface area of the open top box. figure 8. we want to minimize the surface area of a square based box with a given volume. Show solution. 30. x2 −y x 2 − y. for the following exercises (31 36), draw the given optimization problem and solve. 31. find the volume of the largest right circular cylinder that fits in a sphere of radius 1. show solution. 32. find the volume of the largest right cone that fits in a sphere of radius 1. 33.

Get ready to delve into a myriad of Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume-related content that will ignite your curiosity, deepen your understanding, and perhaps even spark a newfound passion. Our goal is to be your go-to resource for all things Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume, providing you with articles, insights, and discussions that cater to your every interest and question.

Ex: Optimization - Minimize the Cost to Make a Can with a Fixed Volume

Ex: Optimization - Minimize the Cost to Make a Can with a Fixed Volume Optimization - Minimize Cost Of A Box Optimization (Minimize Material Cost For a Fixed Volume) Optimization - Minimize Cost of a Box with a Top given a Volume Maximize Volume of an Open Top Box (Optimization) | Calculus 1 Exercises Optimization: Minimize Surface Area of a Box Given the Volume Dimensions of Cylinder that Minimize Cost | Calculus Optimization Problem Optimization: cost of materials | Applications of derivatives | AP Calculus AB | Khan Academy I'm Scared I'll Hurt My Shoulder With These New SLAP Tear Exercises - HELP! Ex: Derivative Application - Minimize the Cost of a Fenced Area Optimization - Minimize Cost given a Specific Volume of an Open Top Box Calculus - Optimization - Open Box Optimization Example: Minimizing Surface Area Given a Fixed Volume Minimizing cost to construct a can Optimization Example (box) Minimizing the Surface Area of a Cylinder with a Fixed Volume Ex: Optimizing the Volume of a Box With Fixed Surface Area How to minimize the surface area of a can with a given volume Maximizing Volume with Cost Constraint 3.6 Optimization Problem #2 - Calculus | MCV4U

Conclusion

After a comprehensive review, it is unmistakable that this specific article gives helpful intelligence related to Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume. In the entirety of the article, the reporter shows a wealth of knowledge about the area of interest. Significantly, the examination of this feature stands out as exceptionally insightful. Whats more, the post shines in elucidating complex concepts in an plain manner. Additionally, the content creator provides applicable examples that enhance the learning experience. An extra component that marks this document as special is the elaborate review of different aspects related to Ex Optimization Minimize The Cost To Make A Can With A Fixed Volume. The authors rigorous method affirms that readership gain a well-rounded understanding of the subject matter. Thank you for reading the document. If you seek more information, please go ahead and reach out utilizing social media platforms. I am enthusiastic about your reactions. In closing, for further insights, provided here are various comparable publications that could potentially be valuable:Enjoy discovering more!