Ex 10 1 14 Equation Of A Circle With Centre 2 2 Passes
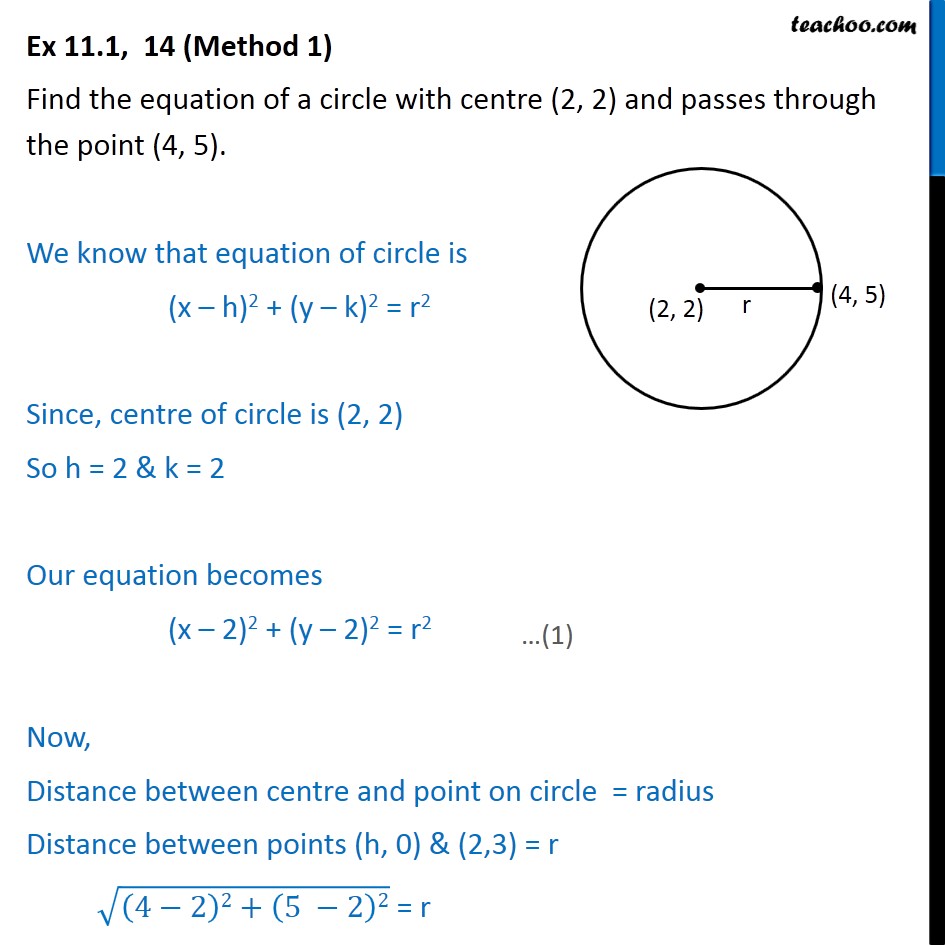
Standard Equation Of A Circle With Center At The Origin Calculator At Transcript. ex 10.1, 14 (method 1) find the equation of a circle with centre (2, 2) and passes through the point (4, 5). we know that equation of circle is (x – h)2 (y – k)2 = r2 since, centre of circle is (2, 2) so h = 2 & k = 2 our equation becomes (x – 2)2 (y – 2)2 = r2 now, distance between centre and point on circle = radius distance between points (h, 0) & (2,3) = r. Ai explanations are generated using openai technology. ai generated content may present inaccurate or offensive content that does not represent symbolab's view. calculate circle's equation using center, radius and diameter step by step. math notebooks have been around for hundreds of years. you write down problems, solutions and notes to go back.
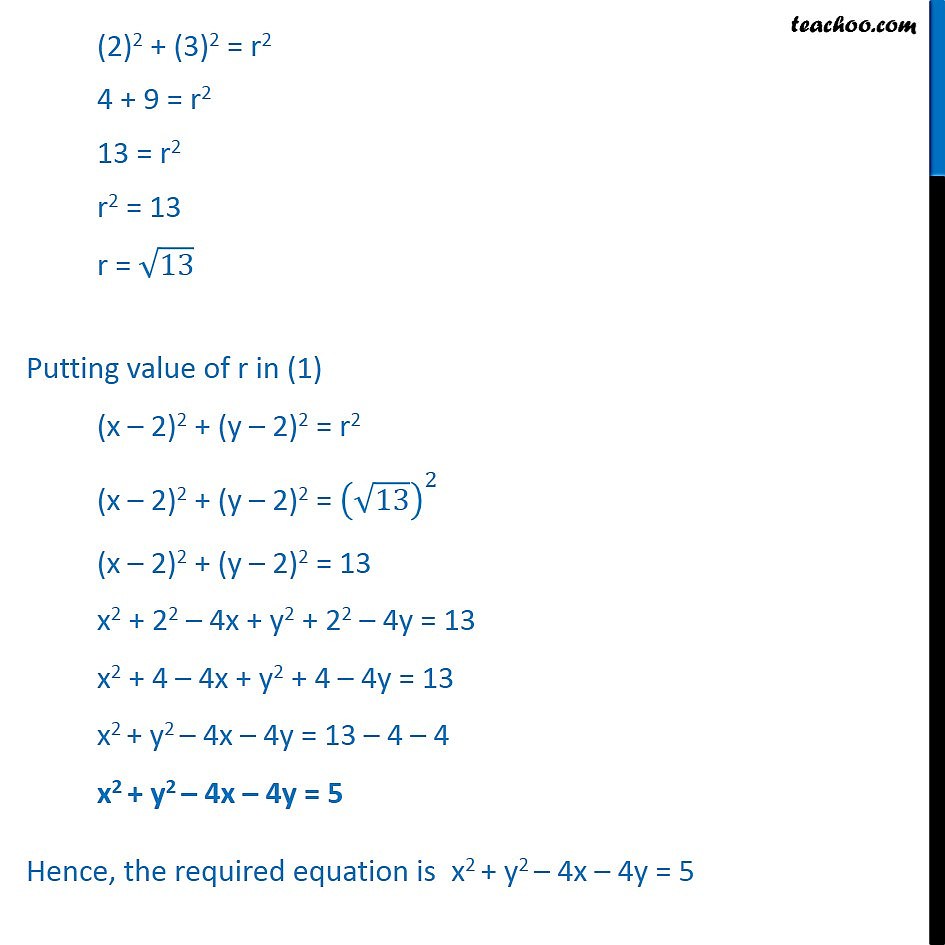
Ex 10 1 14 Equation Of A Circle With Centre 2 2 Passes Find the equation of the circle which touches x axis and whose centre is (1, 2). if one end of a diameter of the circle x 2 y 2 – 4x – 6y 11 = 0 is (3, 4), then find the coordinate of the other end of the diameter. find the equation of the circle having (1, –2) as its centre and passing through 3x y = 14, 2x 5y = 18. He provides courses for maths, science and computer science at teachoo. ex 10.1, 11 find the equation of the circle passing through the points (2, 3) and (–1, 1) and whose centre is on the line x – 3y – 11 = 0. let the equation of the circle be (x – h)2 (y – k)2 = r2. since the circle passes through points (2, 3) point (2, 3) will. Ex 10.1, 12 (method 1) find the equation of the circle with radius 5 whose centre lies on x axis and passes through the point (2, 3). we know that equation of circle is (x – h)2 (y – k)2 = r2 centre of circle is denoted by (h, k) since it lies on x axis , k = 0 hence centre of. Ex 10.2 class 10 maths question 4. prove that the tangents drawn at the ends of a diameter of a circle are parallel. solution: ex 10.2 class 10 maths question 5. prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre. solution: ex 10.2 class 10 maths question 6.

Standard Equation Of A Circle Formula Explained At Jason Drew Blog Ex 10.1, 12 (method 1) find the equation of the circle with radius 5 whose centre lies on x axis and passes through the point (2, 3). we know that equation of circle is (x – h)2 (y – k)2 = r2 centre of circle is denoted by (h, k) since it lies on x axis , k = 0 hence centre of. Ex 10.2 class 10 maths question 4. prove that the tangents drawn at the ends of a diameter of a circle are parallel. solution: ex 10.2 class 10 maths question 5. prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre. solution: ex 10.2 class 10 maths question 6. Ex 1: find the center and the radius of the circle (x− 3)2 (y 2)2 = 16. ex 2: find the center and the radius of the circle x2 y2 2x− 3y − 43 = 0. ex 3: find the equation of a circle in standard form, with a center at c (−3,4) and passing through the point p (1,2). ex 4: find the equation of a circle in standard form, with a center. The centre of the circle is given as (h, k ) = (2, 2) since the circle passes through the point (4, 5), the radius (r) of the circle is the distance between the points (2, 2) and (4, 5). therefore, r = √ (2 4)² (2 5)² = √ ( 2)² ( 3)² = √ 4 9 = √ 13. thus, the equation of the circle is (x h) 2 (y k) 2 = r 2 (x 2.

Ex Find Standard Equation Of A Circle Given Center And Point On The Ex 1: find the center and the radius of the circle (x− 3)2 (y 2)2 = 16. ex 2: find the center and the radius of the circle x2 y2 2x− 3y − 43 = 0. ex 3: find the equation of a circle in standard form, with a center at c (−3,4) and passing through the point p (1,2). ex 4: find the equation of a circle in standard form, with a center. The centre of the circle is given as (h, k ) = (2, 2) since the circle passes through the point (4, 5), the radius (r) of the circle is the distance between the points (2, 2) and (4, 5). therefore, r = √ (2 4)² (2 5)² = √ ( 2)² ( 3)² = √ 4 9 = √ 13. thus, the equation of the circle is (x h) 2 (y k) 2 = r 2 (x 2.

Equation Of A Circle Examples With Answers
General Equation Of A Circle Worksheet

Comments are closed.