Ex 1 Free Undamped Motion Ivp Problem Spring System

Ex 1 Free Undamped Motion Ivp Problem Spring System Youtube This video solves a free undamped motion problem.site: mathispower4u. This video solves a free undamped motion problem.

Ex 1 Free Undamped Motion Ivp Problem Spring System Differential To get an ivp we specify the auxiliary conditions u(0) = u 0, u'(0) = v 0. initial position initial velocity the de for the motion of the mass is mu'' ku = 0. summary: the ivp mu'' ku = 0, u(0) = u 0, u'(0) = v 0 is said to model undamped free vibrations (simple harmonic motion) or sometimes the terms unforced undamped oscillations are used. Here we only discuss the case g(t) = 0, i.e free vibrations, no external force. a solution u(t) of the ode on an interval containing t= 0 that satis es the initial conditions is called the response of the system. spring mass systems: free undamped vibration (or simple harmonic motion) 1. spring on a table (horizontal spring).) mu00= ku 2. a. Ction of the system. a solution u(t) of the de on an interval containingt = 0 t. sp. ing mass systems: free undamped vibration (or simple harmonic motion)1. a exible spring is suspend. d vertically from a rigid support and the mass m is attached to the end. by hooke's law, the spring itself exerts a restoring force f opposite to the direction of. Solution: the gravitational force on the mass (that is, its weight) is mg = 9 .8 n , which exactly balances the restoring force of the spring when the mass is at rest at the equilibrium position. the mass stretches the spring by 0.098m, so the spring constant is k = 9 . 8 n 0.098 m = 100 n m .
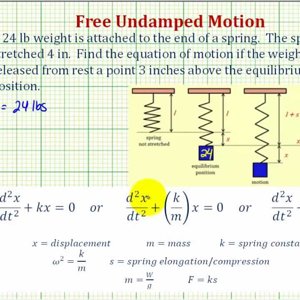
Introduction To Free Undamped Motion Spring System Physics Forums Ction of the system. a solution u(t) of the de on an interval containingt = 0 t. sp. ing mass systems: free undamped vibration (or simple harmonic motion)1. a exible spring is suspend. d vertically from a rigid support and the mass m is attached to the end. by hooke's law, the spring itself exerts a restoring force f opposite to the direction of. Solution: the gravitational force on the mass (that is, its weight) is mg = 9 .8 n , which exactly balances the restoring force of the spring when the mass is at rest at the equilibrium position. the mass stretches the spring by 0.098m, so the spring constant is k = 9 . 8 n 0.098 m = 100 n m . Free oscillations of a mechanical system we first consider the case when there are no external forces acting on the system, and then we have the following homogeneous de. °¯( ) ( ) ° ® = = = 0 0; ' 0 0 '' ' 0 y y y v y m k y c y case 1: simple harmonic motion: there is no damping i.e. when c = 0. ( ) ( ) 22 00 '' ' 0 '' 0; '' 0 0; '0. Example: suppose that the motion of a spring mass system is governed by the initial value problem u'' 5u' 4u = 0, u(0) = 2,u'(0) =1 determine the solution of the ivp and find the time at which the solution is largest. the characteristic equation is r2 5r 4 = 0, so the roots are r = 1 and r = 4. the system is over damped.

Introduction To Free Undamped Motion Spring System Youtube Free oscillations of a mechanical system we first consider the case when there are no external forces acting on the system, and then we have the following homogeneous de. °¯( ) ( ) ° ® = = = 0 0; ' 0 0 '' ' 0 y y y v y m k y c y case 1: simple harmonic motion: there is no damping i.e. when c = 0. ( ) ( ) 22 00 '' ' 0 '' 0; '' 0 0; '0. Example: suppose that the motion of a spring mass system is governed by the initial value problem u'' 5u' 4u = 0, u(0) = 2,u'(0) =1 determine the solution of the ivp and find the time at which the solution is largest. the characteristic equation is r2 5r 4 = 0, so the roots are r = 1 and r = 4. the system is over damped.

Spring Mass Systems Free Undamped Motion Examples Youtube

Comments are closed.